Posts with this logo use 
December 30, 2007
Non-Mathematician Rediscovers Rn, Cn
Posted by John Baez
I did a lot of historical reading about Hamilton, quaternions and the like when writing my review article about the octonions. When Hamilton invented the quaternions in 1843, it was a big deal: people hadn’t realized you could just make up new rules for multiplication. A lot of people joined in the fun, inventing their own hypercomplex number systems. Most of these systems aren’t terribly interesting. Hamilton’s pal John T. Graves found one of the really special ones: the octonions. Clifford found a whole bunch. But in general, most mathematicians now prefer to study hypercomplex number systems en masse rather than individually. They’re now called “real algebras”, and there are lots of nice general theorems about them.
This does not prevent amateurs from continuing to invent hypercomplex number systems and become excited about them. An amusing example was brought to my attention by David Farrell:
- Rodney Rawlings, Non-mathematician devises hypercomplex numbers, with possible implications for mathematics and the philosophy of science.
Brace yourself: Rawlings links his discovery to the Objectivist philosophy of Ayn Rand!
Transgression of n-Transport and n-Connections
Posted by Urs Schreiber
Since it will play a role both for what is currently indicated in section 5 of the article on Lie -algebra connections and their application to String- and Chern-Simons -transport as well as for the next followup of my work with Konrad Waldorf, I am thinking again in more detail about
Trangression of -transport and -connections
Abstract. After going through some ground work concerning generalized smooth spaces and their differential graded commutative algebras of forms, I talk about the issue of transgression of transport -functors and Lie -valued -connections to smooth mapping spaces.
This builds on the general idea of -functorial transgression as the image of an internal hom as first voiced in this old comment and then later incorporated in the discussion of The charged -particle and detailed a bit more in the entry Multiplicative Structure of Transgressed -Bundles.
Currently only a few sketches are present in the above pdf, as I am going to develop this as we go along.
One important aspect, emphasized in the above abstract, is that the discussion greatly profits from a good general understanding of the relation between generalized smooth spaces and their differential graded-commutative algebras of differential forms. I started making comments on that here and now Todd Trimble thankfully chimed in by providing this detailed reply, which I will reproduce below.
But first, I’ll reproduce the introductory remarks from my notes to set the stage.
December 29, 2007
BF-Theory as a Higher Gauge Theory
Posted by Urs Schreiber
I’ll quickly say a couple of words on the occasion of
F. Girelli, H. Pfeiffer, E. M. Popsecu
Topological Higher Gauge Theory - from BF to BFCG theory
arXiv:0708.3051
about the interpretation of the class of topological field theories known as BF-Theory, motivated also by the results Jim, Hisham and myself are talking about in Lie -Connections and their Application to String- and Chern-Simons -Transport.
It’s about if and how exactly to interpret the BF-theory Lagrangian as a functional on Lie -algebra valued forms.
The QG-TQFT Blues
Posted by John Baez
Here at last is the music video we’ve all been waiting for!
- Professor Elvis Zap, The Quantum Gravity Topological Quantum Field Theory Blues.
Elvis Zap, also known as Scott Carter, is a quantum topologist from way down south. He’s one of the guys who first got me interested in possible applications of higher-dimensional knot theory to quantum gravity. That eventually led me to -categories, and I’ve been on a downhill slide ever since. I know the blues he’s singin’ about.
Lyrics follow… and more.
December 28, 2007
Challenges for the Future
Posted by David Corfield
Benjamin Mann of DARPA has constructed a list of 23 challenges for mathematics over the next century.
Whereas Hilbert notes about his 23 problems
I have generally mentioned problems as definite and special as possible, in the opinion that it is just such definite and special problems that attract us the most and from which the most lasting influence is often exerted upon science,
Mann’s challenges are generally open-ended, resembling Hilbert’s sixth problem:
6. Mathematical treatment of the axioms of physics
The investigations on the foundations of geometry suggest the problem: To treat in the same manner, by means of axioms, those physical sciences in which mathematics plays an important part; in the first rank are the theory of probabilities and mechanics.
rather than his thirteenth:
13. Impossibility of the solution of the general equation of the 7-th degree by means of functions of only two arguments.
Some motivation accompanies Mann’s list, but it would be good to see experts write a few paragraphs for each challenge on that inviting parchment background.
December 26, 2007
Geometric Representation Theory (Lecture 17)
Posted by John Baez
This time in the Geometric Representation Theory seminar, James Dolan explains ‘degroupoidification’ — the process of turning a span of groupoids into a linear operator between vector spaces. We’ve been telling people about this for a while now, for example in week256 of This Week’s Finds. But now Jim reveals more about what’s really going on.
December 25, 2007
Lie oo-Connections and their Application to String- and Chern-Simons n-Transport
Posted by Urs Schreiber
Hisham Sati, Jim Stasheff and myself are working on writing up some ideas on Lie -algebra cohomology and its application to String- and Chern-Simons -Transport, further exploring the second edge of the cube.
We would like to share this document:
H. Sati, J. Stasheff, U. S.
-algebra connections and applications to String- and Chern-Simons -Transport
arXiv:http://arxiv.org/abs/0801.3480
(pdf of the latest version)
This consists of three parts:
Part A : Overview and physical applications
Part B: Lie -algebras, their cohomology and their String-like extensions
Part C: Categorified Cartan-Ehresmann connections and lifts through String-like extensions.
This can be thought of as providing details to the discussion provided in my slide show String- and Chern-Simons -Transport. -Café regulars will recognize a certain synthesis of topics I used to discuss here, like inner automorphism -groups, String and Chern-Simons Lie -algebras, -Curvature , obstruction theory and other things. To some extent, the main idea here found its final form after John posed a nice problem in Higher Gauge Theory and Elliptic Cohomology.
Much progress on the relation of the general formalism to (heterotic) string theory and supergravity/M-theory occurred when I visited Hisham Sati in Yale. The full implications are only briefly indicated here.
We’d be grateful for whatever comment you might have.
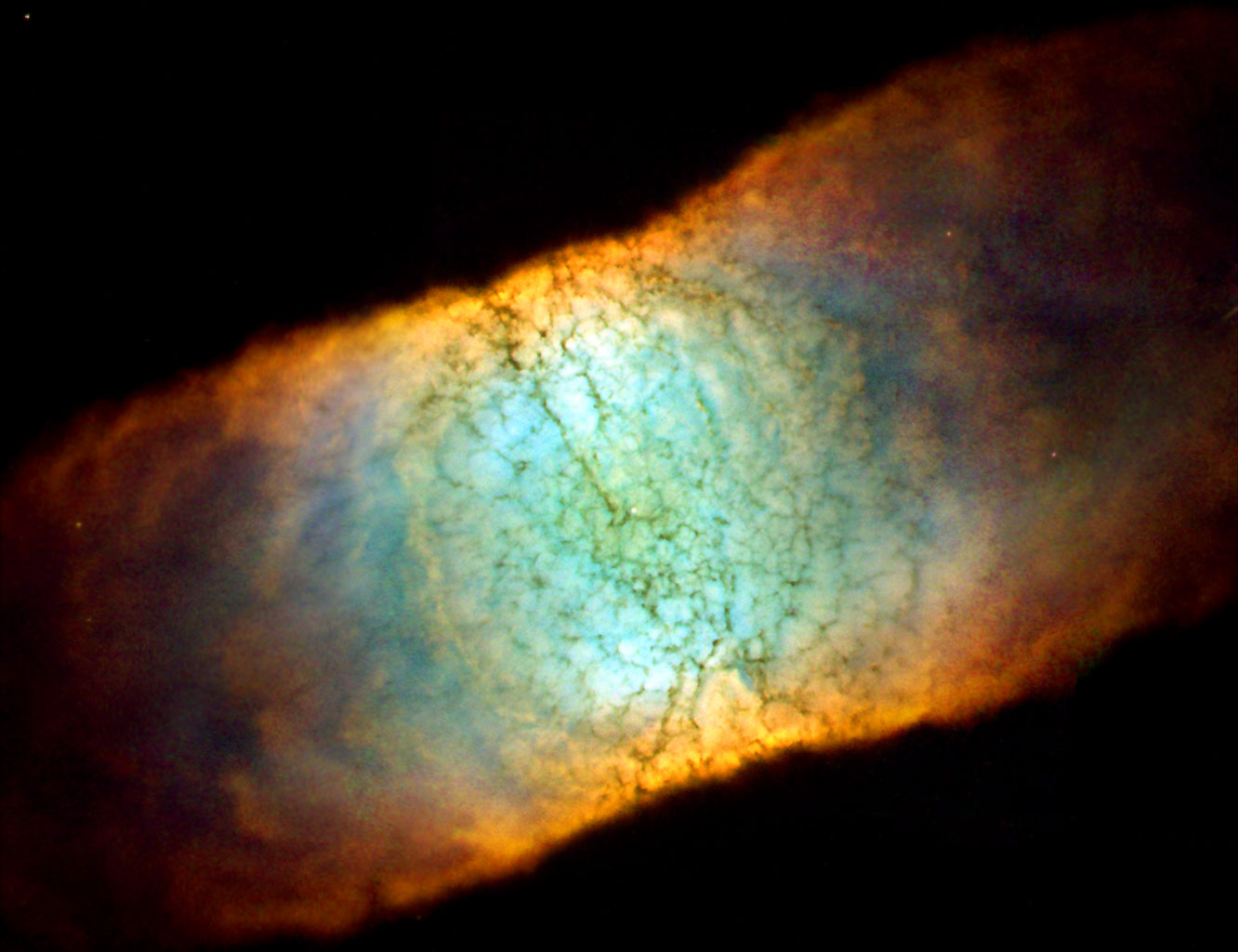
This Week’s Finds in Mathematical Physics (Week 260)
Posted by John Baez
In week260 of This Week’s Finds, learn about the Vishniac instability in the Retina Nebula:
Then try my Christmas eve guide to free books on math and physics. There are more and more available! Soon those expensive textbooks will be obsolete. Here’s a nice illustration of Taylor series for the sine function, from Robert Nearing’s online book Mathematical Tools for Physics:
And finally: the ‘exceptional series’ of Lie algebras.
December 21, 2007
Roytenberg on Weak Lie 2-Algebras
Posted by John Baez
It’s been circulating informally since October, but now it’s available on the arXiv — a proposal for the definition of a fully general categorified Lie algebra!
- Dmitry Roytenberg, On weak Lie 2-algebras.
Abstract: A Lie 2-algebra is a linear category equipped with a functorial bilinear operation satisfying skew-symmetry and Jacobi identity up to natural transformations which themselves obey coherence laws of their own. Functors and natural transformations between Lie 2-algebras can also be defined, yielding a 2-category. Passing to the normalized chain complex gives an equivalence of 2-categories between Lie 2-algebras and 2-term “homotopy everything” Lie algebras; for strictly skew-symmetric Lie 2-algebras, these reduce to -algebras, by a result of Baez and Crans. Lie 2-algebras appear naturally as infinitesimal symmetries of solutions of the Maurer–Cartan equation in some differential graded Lie algebras and -algebras. In particular, (quasi-) Poisson manifolds, (quasi-) Lie bialgebroids and Courant algebroids provide large classes of examples.
Progic VI
Posted by David Corfield
I talked about Bayesian networks back here as a prelude to the shift from propositional to first-order relational structures. But before we take that step, it’s worth mentioning that there are other graphical models used in probabilistic reasoning. (If you prefer videos try this or this.)
In particular, there’s a class of models called Markov networks, which unlike Bayesian networks involve undirected edges. Given an undirected graph, a distribution is given by assigning non-negative real functions, , to cliques, , in the graph. These are called potential functions.
Then the probability of the node variables being in a given state is given by the product of the potential functions evaluated at the corresponding clique states, divided by a normalizing factor. All of which should put you in mind of certain models from statistical physics.
Non-Commutative Structures in Arithmetic and Geometry
Posted by Urs Schreiber
guest post by Minhyong Kim
In June of next year, the will be an informal workshop at University
College London on
Non-commutative structures in arithmetic and geometry
The idea is for a small group of experts from rather different areas to get together to explain to each other what it is they do, and possibly discover some unifying themes. The areas represented will be
Non-commutative Iwasawa theory, non-commutative geometry, non-abelian class field theory, non-abelian Hodge theory, Anabelian geometry, and, hopefully, some non-abelian physics.
A preliminary website is here .
Note also the related program at the Newton Institute.
Geometric Representation Theory (Lecture 16)
Posted by John Baez
Sick of Christmas shopping? Tired of the crowded malls, the rush, the commercialism? Take a break and learn some math! It’s free, it’s fun, and it’s good for you.
This time in the Geometric Representation Theory seminar, I start by quickly fixing the mistake I made when attempting to state the ‘Fundamental Theorem of Hecke Operators’ in lecture 14.
But then I begin the harder and more interesting job of trying to explain what’s really going on! Namely, ‘groupoidification’.
This is what our seminar is really about. We were just taking our time getting there, building up some of the key examples we’ll be using.
The first step is to see that groups acting on sets give groupoids. This nicely fits the idea of a groupoid as a ‘set with built-in symmetries’.
December 17, 2007
Basic Bundle Theory and K-Cohomology Invariants
Posted by Urs Schreiber
My friend Branislav Jurčo (who -Café regulars know from his Notes on Generalized Bundle Gerbes) has been working on a book on bundles and on K-theory. Now this is finally done:
Dale Husemöller, Michael Joachim, Branislav Jurco and Martin Schottenloher
Basic Bundle Theory and K-Cohomology Invariants
Lecture Notes in Physics 726, Springer-Verlag Berlin Heidelberg 2008
It even contains a discussion of String structures, which are really lifts to 2-bundles. (See also Jurčo on gerbes and stringy applications.)