The n-Category Café

There are lots of ways of categorically presenting “algebraic theories;” three of the most well-known are operads, Lawvere theories, and monads. In fact, operads and monads lie near opposite ends of a continuum of such notions, ranging from “less expressive and more controlled” (operads) to “more expressive and less controlled” (monads). One uniform framework for such “notions of theory” and their corresponding “functorial semantics” is the theory of generalized operads and multicategories.

My goal in this post is to explain how a couple of fairly obscure-seeming kinds of generalized operad are actually implicit in some very classical algebraic topology. In particular, they provide a way to “make good categorical sense” out of two constructions on topological operads that have always confused me: the “category of operators” associated to an operad, and the two different monads on based and unbased spaces associated to a “reduced” operad.

In week282 of This Week’s Finds, visit Mercury:

Learn how this planet’s powerful magnetic field interacts with the solar wind to produce flux transfer events and plasmoids. Then read about the web of connections between associative, commutative, Lie and Poisson algebras, and how this relates to quantization. In the process, you’ll meet linear operads, their generating functions, and Stirling numbers of the first kind!

With the continued development of the nLab and the Polymath Projects, and the rise of Math Overflow as a competitor to sci.math.research, mathematicians are busy talking about new ways to take advantage of technology. The arXiv is great for papers. Blogs are great for conversations. But how to more effectively gather, store, and make accessible the folk wisdom that traditionally spread through informal person-to-person conversations?

There surely won’t be just one answer to this question. And surely the answers won’t be found just by discussion. We’ll need to grope our way there by trying many different things and seeing what works.

But humans being human, it’s irresistible and probably necessary to talk about this question. Other disciplines must be having similar discussions — does anyone know where? It would be good to see them.

Here on the $n$-Café, conversations keep drifting towards this subject…

Following a suggestion by some publishing company, there is the idea of creating a book that collects contributions from various authors on the topic Mathematical Foundations of Quantum Field and Perturbative String Theory .

We have an idea for a proposed “Call for Papers”. But we would like to get some comments on this, from people who have experience with such issues.

Mathematical Foundations of Quantum Field and Perturbative String Theory – Call for Comments on Call for Papers

College textbooks are really expensive these days. In California, a law was passed to tackle this problem. But it seems to lack teeth.

One way to tackle this problem is to develop free online textbooks. I think a wiki-based approach could be good. People are trying it. Will it ever catch on?

It might also make sense for the NSF, or other funding agencies, to pay for scholars to write free online textbooks — or improve existing ones.

Now this has finally happened.

Welcome to week 2 of the cobordism and TFT seminar at UCR. This is the first of a series of lectures given by Julie Bergner (with occasional stand-ins by others). The previous lecture was an introductory lecture given by John Baez found here. This week’s lecture introduces cobordisms and oriented manifolds.

I’ve recently run into the question of how best to lay out a fairly large commutative diagram. Some diagrams have a “natural” shape such as a cube or a simplex, but as far as I can tell that is not the case for the diagrams in question. They aren’t complicated, mostly just a bunch of naturality squares stuck together. But different people seem to have different aesthetic viewpoints on what makes the layout of a diagram “look good.” So I thought I’d share my data so far, and see whether anyone here has additional insights.

In week281 of This Week’s Finds, learn about the newly discovered ring of Saturn — the Phoebe ring — and how it explains the mystery of Iapetus.

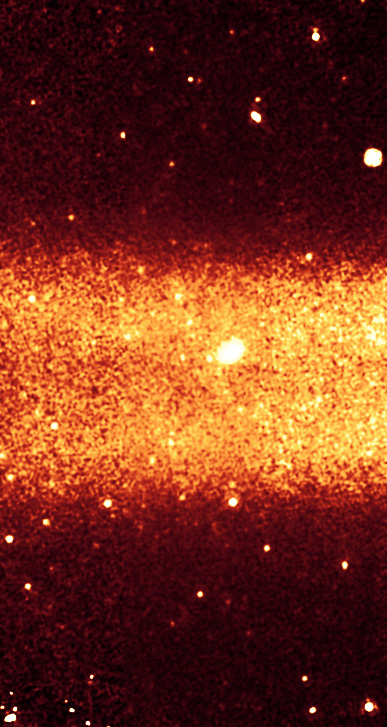
See Egan’s new applet that produces ever-expanding tilings with 10-fold quasisymmetry. Delve deeper into the history of these tilings, which date back to the Timurid dynasty. Go back all the way to the Topkapi Scroll… then go modern and check out tiling patterns in spherical and hyperbolic geometry. Finally, hear about strings in 4d BF theory, and spin foam models based on the representation theory of 2-groups.

Sparked by Arnold Neumaier’s work on “a computer-aided system for real mathematics,” we had a very stimulating discussion, mostly about various different foundations for mathematics. But that thread started getting rather long, and John pointed out that people without some experience in formal logic might be feeling a bit lost. In this post I’ll pontificate a bit about logic, type theory, and foundations, which have also come up recently in another thread. Hopefully this too-brief summary of background will make the discussion in the next post about the meaning of structuralism more readable. (Some of this post is intended as a response to comments on the previous discussions, but I probably won’t carefully seek them out and make links.)

Over in a discussion at Math Overflow I was reminded about Halmos’ great article on writing mathematics, which I highly recommend to all graduate students (or anyone else, for that matter).

• P. R. Halmos, How to write mathematics, L’Enseignement Mathématique, Vol.16 (1970) [31 pages, 3.3MB].

The math-blogosphere is abuzz with interest in the new Math Overflow, a mathematics questions and answers site. Already we at the Café have been helped with the answer to a query on the Fourier transform of a certain kernel, and there are some juicy questions for us to answer there too, including

• What is the size of the category of finite dimensional $F_q$ vector spaces?, where size is in the sense of the Leinster-Euler characteristic.
• “Wick rotation” of tropical geometry, very much a matrix mechanics kind of question.

You can read a discussion on Math Overflow, and a debate concerning its advantages relative to $n$Lab.

Check out Greg Egan’s new Girih applet that generates quasiperiodic tilings with ten-fold rotational symmetry, using a method called "inflation". You’ll see patterns like this, that keep zooming in endlessly…

This quarter Julie Bergner has begun a seminar at UC Riverside on cobordisms and topological field theories. The abstract on the seminar homepage states:

In this seminar we’ll work through recent notes of Lurie giving an outline of his proof of the Cobordism Hypothesis, relating cobordism classes of manifolds and topological field theories. This work brings together several areas of recent mathematical interest: topological field theories, cobordisms of manifolds, and homotopical approaches to higher categories. We’ll go over basic definitions and examples of all of the above and then work towards understanding Lurie’s proof.

The main reference for this seminar is Jacob Lurie’s paper on the classification of topological field theories.

You can also see the list of scheduled speakers on the homepage. I believe the plan is that Julie will give most of the talks while others fill in once in a while.

Our $n$Lab entry for ETCS has

The axioms of ETCS can be summed up in one sentence as:

$\bullet$ The category of sets is a well-pointed topos with a natural numbers object satisfying the axiom of choice.

Do we take then models of ETCS as forming the 2-category of well-pointed toposes with a natural numbers object satisfying the axiom of choice? And is $Set$ initial in this 2-category? If so, is it then an initial (2)-algebra of sorts? And if all that, is there an analogue terminal (2)-coalgebra?

I know about the idea of well-founded and non-well-founded sets as minimal and maximal fixed points for the powerset functor on the category of classes, as in algebraic set theory. I was wondering if there is something similar going on in a structural set theory such as ETCS.

It seems that this Friday I’ll give a talk to the group of Ieke Moerdijk, where I just started a new position (as I mentioned).

Over on the $n$Lab I am preparing some notes along which such a talk might proceed:

Path-Structured Smooth $(\infty,1)$-Toposes (wiki page)

Abstract. A smooth topos is a context in which (synthetic) differential geometry exists. An $(\infty,1)$-topos is a context in which higher groupoids exist. Merging these two concepts yields the notion of a smooth $(\infty,1)$-topos: a context in which $\infty$-Lie groupoids exist.

A lined topos is a context in which each space has a notion of path. A path-structured smooth $(\infty,1)$-topos is a context in which each $\infty$-Lie groupoid comes with its smooth path $\infty$-groupoid, naturally.

Path-structured and smooth $(\infty,1)$-toposes are the context in which gauge fields given by principal $\infty$-bundles with connection exist.

I promise some entries with mathematical content soon, but today I wanted to draw attention to some upcoming workshops affiliated with the MSRI semester on knot homology theories . The two workshops are:

• The Connections for Women Workshop,

organized by Eli Grigsby, Olga Plamenevskaya, Katrin Wehrheim, and what is called

• The Introductory Workshop,

organized by Aaron Lauda, Robert Lipshitz, Dylan Thurston.

I’ll leave any actual information regarding the content until below the fold. Just so people who do not already love knot homology theories keep on reading, I will quote the organizer’s invitation:

Both workshops will feature a mix of survey, introductory, and research talks, which are aimed at non-experts. One goal is to familiarize workers in one of the fields represented with the tools and ideas of the other fields.

They seem to have some funding for graduate students and fresh Ph.D.’s, so this is a good opportunity for graduate students to get some exposure to some exciting topics.

I have just about finished a paper on the magnitude of metric spaces, for which comments, corrections or questions are welcome.

• Heuristic and computer calculations for the magnitude of metric spaces (15pp. 800KB).

This paper provides more motivation for the asymptotic conjecture in my paper with Tom Leinster described in a recent post by Tom.

As the title suggests, at the core of this paper are some back-of-the-envelope calculations and some big number crunching with Maple. The former leading to some conjectures which are supported by the latter.

I have two questions for patrons of the Café, the first of which does not require any familiarity with the paper.

1. Is there a natural phenomenon which decreases exponentially with distance (as opposed to decreasing exponentially with time)?
2. Is there a better term than ‘penguin valuation’ for the invariant valuation $P$ that is defined in the paper?

Guest post by Emily Riehl

A popular slogan is that $(\infty,1)$-categories (also called quasi-categories or $\infty$-categories) sit somewhere between categories and spaces, combining some of the features of both. The analogy with spaces is fairly clear, at least to someone who is happy to regard spaces as Kan complexes, which are simplicial sets in which every horn can be filled. The analogy with categories is somewhat more subtle. We choose to model $(\infty,1)$-categories as quasi-categories, which are a particular type of simplicial set. When we regard the 0-simplices of an quasi-category as its objects and the 1-simplices as its morphisms, we can define a weak composition law that is well-defined, unital, and associative only up to homotopy. This means exactly that when we replace the 1-simplices by homotopy classes of 1-simplices we obtain an ordinary category, called the homotopy category of our quasi-category.

However, a lot of data is lost when we replace an quasi-category by its homotopy category. In particular, there exists a 3-simplex that witnesses the fact that a particular composite $(h g)f$ of 1-simplices $f$, $g$, and $h$ (where some composite $h g$ of $g$ and $h$ has already been chosen) is also a composite of $g f$ (a chosen composite of $f$ and $g$) and $h$. This is well-known by those who are familiar with the construction of the homotopy category mentioned above, but a question remains: what sort of associativity data is provided by the $n$-simplices, for $n \gt 3$?

I got an email from the author of this paper:

• Dennis Borisov, Comparing definitions of weak higher categories, I.

asking for comments. Unfortunately I’m completely busy with work on other subjects — and some of you reading this are much better than me at comparing various definitions of $n$-category! So maybe you can say something?

Israel Gelfand died yesterday, at the age of 96.

He played a preeminent role in Russian mathematics for many years. His legendary seminar in Moscow ran for 50 years, and set the pattern for many others, including Sullivan’s seminar at CUNY and Drinfeld’s at Chicago. His name is attached to many mathematical achievements, including:

• the Gelfand representation of a Banach algebra,
• an important special case, the Gelfand–Naimark theorem describing commutative C*-algebras,
• the Gelfand–Naimark–Segal construction describing general C*-algebras,
• the Gelfand–Fuks cohomology of a foliation,
• the Gelfand–Kirillov dimension of an associative algebra,
• the Bernstein–Gelfand–Gelfand resolution for representations of simple Lie groups.

But this are just the tip of the iceberg! For example, seeing these you might never guess that he helped write a 5-volume work on distribution theory.

John kindly offered to host the contents of my old blog – The Philosophy of Real Mathematics. They are up already here. Unfortunately, the internal links to others of my pages won’t work. Where for instance they say

http://www.dcorfield.pwp.blueyonder.co.uk/2006/02/articulating-your-program.html,

they need to say

http://math.ucr.edu/home/baez/corfield/2006/02/articulating-your-program.html.

John tells me that something called a shell script might be able to perform the translation. Does anyone have an idea of how this could be achieved?

Aaron Lauda, Anthony Licata and Alistair Savage (all of whom we are lucky to have speaking at the AMS meeting at Riverside this Fall) have just announced their Special Issue on “Categorification in Representation Theory” of the International Journal of Mathematics and Mathematical Statistics.

The official Call for Papers is here.

Congratulations to Steve Lack for winning one of this year’s The Australian Mathematical Society Medals, in honour of which he gets a page at nLab.

I’m desperately catching up on about a hundred emails I didn’t read while I was in Corfu — an island where, strangely, hotels on the beach lack wireless internet.

Here’s the most fun one so far:

Dear John,

Nerve.com is interviewing mathematicians for our very popular weekly feature, “Sex Advice From…,” in which we invite groups of people to be relationship-advice gurus for a day. I found your cool blog, and wondered if you or any mathematician friends might be interested in being interviewed and giving some advice?

The interview usually takes about 20 minutes, can be done over the phone, and is a lot of fun. Or, if you prefer, I can email you the questions to answer. We publish about six or seven of your answers, along with your first name and a photo. We would also be happy to link to your blog/website, if you like.

Here are some past “Sex Advice From…” columns, if you’d like to check them out:

http://advice.nerve.com/2009/08/21/sex-advice-from-pastors/

http://advice.nerve.com/2009/08/07/sex-advice-from-goths/

http://advice.nerve.com/2009/07/17/sex-advice-from-grandparents/

If you’re not familiar with us:

Nerve is the premier online magazine for love, sex and culture. We have a readership of close to one million highly educated twenty- and thirty-somethings. We interview several directors, musicians, authors and actors each month. Our award-winning in-depth interviews include, most recently, Ben Folds, Salman Rushdie, Maureen Dowd, Norman Mailer, John Malkovich, Philip Seymour Hoffman, Arianna Huffington, David Cronenberg, John Updike and Philip Roth.

Thanks for your time, and I hope to hear from you soon!

Kind regards,
Nelson Antonio Bermudez