More Magnitude of Metric Spaces and Problems with Penguins
Posted by Simon Willerton
I have just about finished a paper on the magnitude of metric spaces, for which comments, corrections or questions are welcome.
This paper provides more motivation for the asymptotic conjecture in my paper with Tom Leinster described in a recent post by Tom.
As the title suggests, at the core of this paper are some back-of-the-envelope calculations and some big number crunching with Maple. The former leading to some conjectures which are supported by the latter.
I have two questions for patrons of the Café, the first of which does not require any familiarity with the paper.
- Is there a natural phenomenon which decreases exponentially with distance (as opposed to decreasing exponentially with time)?
- Is there a better term than ‘penguin valuation’ for the invariant valuation that is defined in the paper?
I will use motivating those two questions as an excuse to describe the contents of the paper.
To start with the first question, let me first remind you of the definition of a weighting of a finite metric space that was given in the paper with Tom (it didn’t appear in his original metric spaces post).
Suppose that is a finite metric space. A weighting on is an assignment of a weight to each point in such that for each the weight equation for is satisfied:
If a weighting for exists then , the magnitude of is defined to be the sum of the weights:
This algebraic description comes by analogy with Tom’s corresponding definitions for finite categories, but it doesn’t come with any intuition, at least not for me. So I developed the following picture. Each point in is thought to be an organism which can regulate the amount of heat it generates (or absorbs). The amount of heat that the organism is generating is given by the weight , where a negative weight indicates that heat is being absorbed, or, in other words, some kind of refrigeration or air-conditioning is happening at the point . The amount that the generated heat is felt falls off exponentially with distance from the organism. Each organism wishes to maintain a constant body temperature of one unit and they all regulate their heat to achieve that: this translates into precisely the weight equations being satisfied.
Now, I realise that there are many problems with that picture, however I do find it helpful and it does give me some kind of useful intuition — I’ll mention some related things below. But this does lead to the first question as to whether one can think of any genuine phenomena which decay exponentially with distance. A chemist friend mentioned possibly short-range repulsion of molecules, but I couldn’t really find anything on the internet about that.
Before getting to the second question, I should add that the original analogy that I had in mind was that the weight represented the ‘ego’ of an organism (or person or amoeba as I sometimes thought of them); so a positive weight represented extroversion and a negative weight represented introversion. The weight equation then represented the idea that the strength of someone’s personality drops off exponentially with distance and that each organism needed a certain level of ‘companionship’ so as not to go insane — so if they were very isolated they needed a strong extrovert personality to make up for it. However, it was pointed out to me that this perspective was rather artificial, unbelievable and idiosyncratic. Richard Thomas helped me come up with this, seemingly more acceptable, heat analogy.
Here’s an example of a weighting which demonstrates the analogy. In the picture below, we take the metric space to be a grid of points on a square in . The area of is proportional to the weight of the point at its centre, with a red disc meaning a positive weight and a blue disc meaning a negative weight.
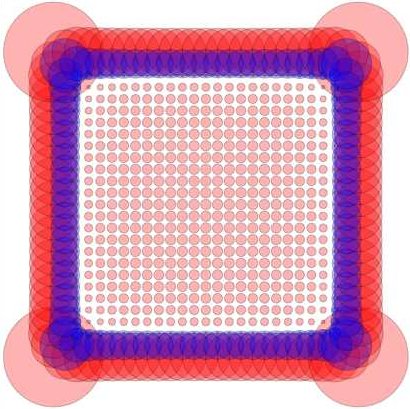
Tom pointed out to me that this is reminiscent of emperor penguins huddling on the Antarctic ice over the winter – you can see this in a clip of the BBC’s Life In The Freezer series. The penguins in the middle of the huddle are nice and cosy, being surrounded by other warm bodies, whereas the penguins on the edge of the huddle are more exposed and have to use up more energy generating heat. In the above picture of the weighting on the square grid, you see that the ‘penguins’ on the boundary have to generate so much heat to keep warm that the ones just inside from the boundary have to ‘switch on the air-conditioning’ (i.e., have negative weight as represented by the blue discs) in order not to get too hot.
What we are actually interested in are compact metric spaces rather than just finite ones. We can try to define the magnitude of a compact metric space by picking as sequence of finite subsets of with in the Hausdorff topology and defining the magnitude of to be the limit of the magnitude of these finite approximations to : so . Whilst we don’t know that this works in general, it does seem to work for compact subsets of Euclidean spaces. The definition means that a good approximation to the magnitude of a compact set (if it exists) can be obtained by calculating the magnitude of a suitably dense finite subset.
Now suppose is the closure of an open subset of , for instance a square in . Then if is ‘large’ you can do a heuristic argument to estimate the contribution to the magnitude of from the points in the ‘bulk’ of (or from the ‘cosy penguins’ if you will). Denoting the volume of the -dimensional ball by , the estimate of this bulk contribution is
I have no idea how to give a similar heuristic for what is happening at the boundary – in particular I can’t quantitatively explain the negative weights. However I can come up with a functional which seems to give the right answer in several cases. For this you need to recall the intrinsic volumes which I denote by , but which Tom denoted by in his metric spaces post. These are defined on certain compact subsets of : I’m not sure of the exact domain of definiton, but they are certainly defined on finite unions of convex sets and and smooth submanifolds. They have various properties, but the pertinent ones here are and for can be localized to the boundary. For instance, is half the “surface area” of , or half the -volume of the boundary, if you prefer; and is the Euler characteristic of .
Motivated by a wild assumption of some kind of naturality, define the functional
By the properties of the intrinsic volumes mentioned above, is of the form
When we have
We’re just about ready for the second question now, but let’s conclude the mathematics first. The functional seemed to be a bit of a guess at some estimate of the magnitude when is a ‘large’ space. The amazing thing, and one of the key points of the paper, is that for squares, discs and cubes, i.e., the convex spaces that I did calculations for, these two functionals seem to agree to a large extent. So one is led to conjecture that
One the other hand, for annuli and tori these two functionals just seem to agree asymptotically, meaning that if we denote by the metric space with distance scaled up by a factor then
The paper includes some nice graphs of this behaviour. However, it is important to say here [which I didn’t do before David Speyer reminded me] that I don’t know what sort of spaces to expect this asymptotic behaviour for (other than the closure of open sets of Euclidean space ). In particular, David Speyer pointed out in an earlier post the case of the bent line in which two straight line segments are stuck together at an angle: numerical calculations for the magnitude seem to show a small, constant discrepancy with the penguin valuation. I have also done some asymptotic analysis with the -sphere which seems to show that the lower order terms are not right in that case – however I’m not so confident in the calculations at the moment.
Now to get to the question. What can I call ? I tried to think of a name for it for a long time. In the end I plumped for the term ‘penguin valuation’ for the following reasons.
- I couldn’t think of anything else.
- In the paper with Tom the symbol had already been used for it.
- [Erm, a bit weak this one, and thought up afterwards.] It does kind of create an image in the mind of contribution from the cosy penguins and from the penguins on the boundary.
However, some might find the term overly whimsical. Does anyone have any better ideas?
I’ll finish with a trivia question for you.
- What’s the connection between the Leicester mathematics department and the BBC penguin video?

Re: More Magnitude of Metric Spaces and Problems with Penguins
I haven’t even looked at the paper yet (I will!) and haven’t even finished reading your post, but thought I would go ahead and respond to this:
A plane wave (in Fourier space) can be expressed as
When the material is lossy, the wave vector decomposes into a real and imaginary parts so we’ve got:
which is a plane wave whose amplitude decreases exponentially with distance from the source.
That may or may not help. Back to the post! :)