April 30, 2024
Line Bundles on Complex Tori (Part 5)
Posted by John Baez
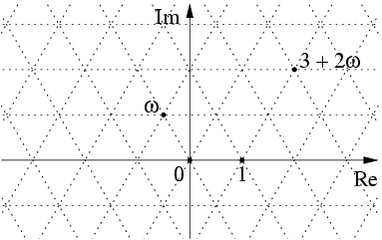
The Eisenstein integers are the complex numbers of the form where and are integers and . They form a subring of the complex numbers and also a lattice:
Last time I explained how the space of hermitian matrices is secretly 4-dimensional Minkowski spacetime, while the subset
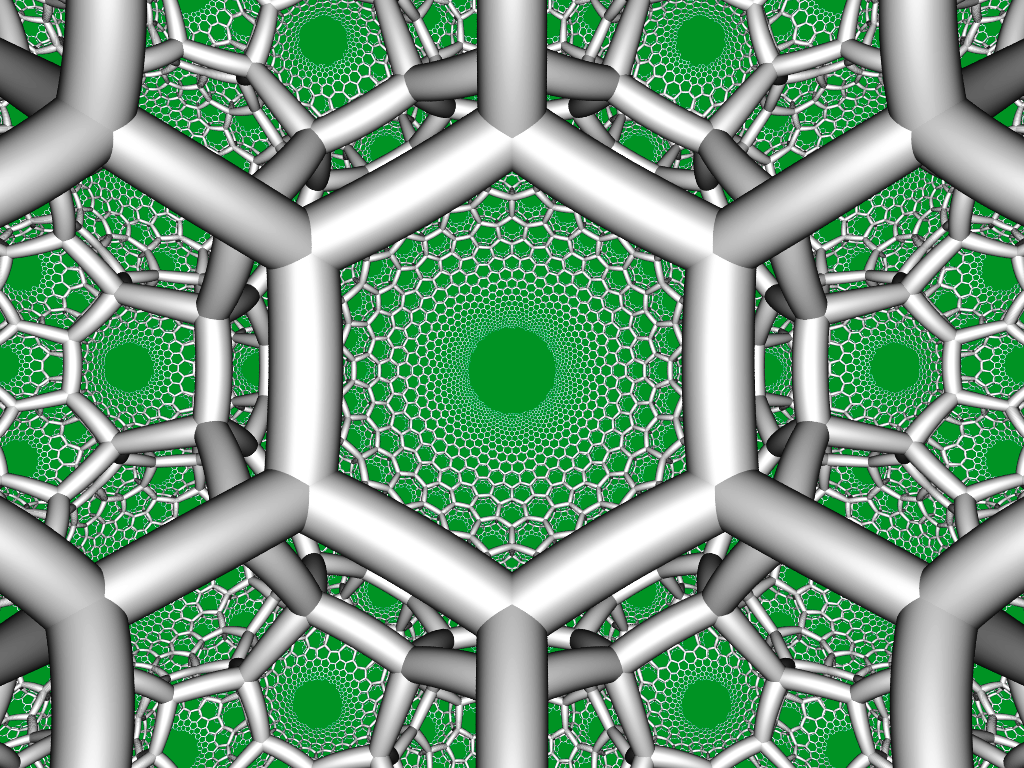
is 3-dimensional hyperbolic space. Thus, the set of hermitian matrices with Eisenstein integer entries forms a lattice in Minkowski spacetime, and I conjectured that consists exactly of the hexagon centers in the hexagonal tiling honeycomb — a highly symmetrical structure in hyperbolic space, discovered by Coxeter, which looks like this:
Now Greg Egan and I will prove that conjecture.
April 26, 2024
Line Bundles on Complex Tori (Part 4)
Posted by John Baez
Last time I introduced a 2-dimensional complex variety called the Eisenstein surface
where is the lattice of Eisenstein integers. We worked out the Néron–Severi group of this surface: that is, the group of equivalence classes of holomorphic line bundles on this surface, where we count two as equivalent if they’re isomorphic as topological line bundles. And we got a nice answer:
where consists of hermitian matrices with Eisenstein integers as entries.
Now we’ll see how this is related to the ‘hexagonal tiling honeycomb’:
We’ll see an explicit bijection between so-called ‘principal polarizations’ of the Eisenstein surface and the centers of hexagons in this picture! We won’t prove it works — I hope to do that later. But we’ll get everything set up.
April 25, 2024
Line Bundles on Complex Tori (Part 3)
Posted by John Baez
You thought this series was dead. But it was only dormant!
In Part 1, I explained how the classification of holomorphic line bundles on a complex torus breaks into two parts:
the ‘discrete part’: their underlying topological line bundles are classified by elements of a free abelian group called the Néron–Severi group .
the ‘continuous part’: the holomorphic line bundles with a given underlying topological line bundle are classified by elements of a complex torus called the Jacobian .
In Part 2, I explained duality for complex tori, which is a spinoff of duality for complex vector spaces. I used this to give several concrete descriptions of the Néron–Severi group .
But the fun for me lies in the examples. Today let’s actually compute a Néron–Severi group and begin seeing how it leads to this remarkable picture by Roice Nelson:
This is joint work with James Dolan.
April 23, 2024
Moving On From Kent
Posted by David Corfield
Was it really seventeen years ago that John broke the news on this blog that I had finally landed a permanent academic job? That was a long wait – I’d had twelve years of temporary contracts after receiving my PhD.
And now it has been decided that I am to move on from the University of Kent. The University is struggling financially and has decreed that a number of programs, including Philosophy, are to be cut. Whatever the wisdom of their plan, my time here comes to an end this July.
What next? It’s a little early for me to retire. If anyone has suggestions, I’d be happy to hear them.
We started this blog just one year before I started at Kent. To help think things over, in the coming weeks I thought I’d revisit some themes developed here over the years to see how they panned out:
- Higher geometry: categorifying the Erlanger program
- Category theory meets machine learning
- Duality
- Categorifying logic
- Category theory applied to philosophy
- Rationality of (mathematical and scientific) theory change as understood through historical development
April 19, 2024
The Modularity Theorem as a Bijection of Sets
Posted by John Baez
guest post by Bruce Bartlett
John has been making some great posts on counting points on elliptic curves (Part 1, Part 2, Part 3). So I thought I’d take the opportunity and float my understanding here of the Modularity Theorem for elliptic curves, which frames it as an explicit bijection between sets. To my knowledge, it is not stated exactly in this form in the literature. There are aspects of this that I don’t understand (the explicit isogeny); perhaps someone can assist.
April 18, 2024
The Quintic, the Icosahedron, and Elliptic Curves
Posted by John Baez
Old-timers here will remember the days when Bruce Bartlett and Urs Schreiber were regularly talking about 2-vector spaces and the like. Later I enjoyed conversations with Bruce and Greg Egan on quintics and the icosahedron. And now Bruce has come out with a great article linking those topics to elliptic curves!
- Bruce Bartlett, The quintic, the icosahedron, and ellliptic curves, Notices of the American Mathematical Society 71 (April 2024), 447–453.
It’s expository and fun to read.
April 17, 2024
Pythagorean Triples and the Projective Line
Posted by John Baez
Pythagorean triples like may seem merely cute, but they’re connected to some important ideas in algebra. To start seeing this, note that rescaling any Pythagorean triple gives a point with rational coordinates on the unit circle:
Conversely any point with rational coordinates on the unit circle can be scaled up to get a Pythagorean triple.
Now, if you’re a topologist or differential geometer you’ll know the unit circle is isomorphic to the real projective line as a topological space, and as a smooth manifold. You may even know they’re isomorphic as real algebraic varieties. But you may never have wondered whether the points with rational coordinates on the unit circle form a variety isomorphic to the rational projective line .
It’s true! And since is plus a point at infinity, this means there’s a way to turn rational numbers into Pythagorean triples. Working this out explicitly, this gives a nice explicit way to get our hands on all Pythagorean triples. And as a side-benefit, we see that points with rational coordinates are dense in the unit circle.
April 15, 2024
Semi-Simplicial Types, Part II: The Main Results
Posted by Mike Shulman
(Jointly written by Astra Kolomatskaia and Mike Shulman)
This is part two of a three part series of expository posts on our paper Displayed Type Theory and Semi-Simplicial Types. In this part, we cover the main results of the paper.
April 10, 2024
Machine Learning Jobs for Category Theorists
Posted by John Baez
Former Tesla engineer George Morgan has started a company called Symbolica to improve machine learning using category theory.
When Musk and his AI head Andrej Karpathy didn’t listen to Morgan’s worry that current techniques in deep learning couldn’t “scale to infinity and solve all problems,” Morgan left Tesla and started Symbolica. The billionaire Vinod Khosla gave him $2 million to prove that ideas from category theory could help.
Khosla later said “He delivered that, very credibly. So we said, ‘Go hire the best people in this field of category theory.’ ” He says that while he still believes in OpenAI’s continued success building large language models, he is “relatively bullish” on Morgan’s idea and that it will be a “significant contribution” to AI if it works as expected. So he’s invested $30 million more.
 Posts with this logo use
Posts with this logo use