October 30, 2008
Twisted Differential Nonabelian Cohomology
Posted by Urs Schreiber
This is something we are currently working on, various aspects of which have been the subject of recent discussion here:
Hisham Sati, U. S., Zoran Škoda, Danny Stevenson
Twisted differential nonabelian cohomology
Twisted -brane -bundles and their Chern-Simons -bundles with characteristic -classes
(pdf,
60 pages theory, 40 pages application currently (but still incomplete))
Abstract. We introduce nonabelian differential cohomology classifying -bundles with smooth connection and their higher gerbes of sections, generalizing [SWIII]. We construct classes of examples of these from lifts, twisted lifts and obstructions to lifts through shifted central extensions of groups by the shifted abelian -group . Notable examples are String 2-bundles [BaSt] and Fivebrane 6-bundles [SSS2]. The obstructions to lifting ordinary principal bundles to these, hence in particular the obstructions to lifting -structures to -structures and further to -structures [SSS2, DHH], are abelian Chern-Simons 3- and 7-bundles with characteristic class the first and second fractional Pontryagin class, whose abelian cocycles have been constructed explicitly by Brylinski and McLaughlin [BML]. We realize their construction as an abelian component of obstruction theory in nonabelian cohomology by -Lie-integrating the -algebraic data in [SSS1]. As a result, even if the lift fails, we obtain twisted String 2- and twisted Fivebrane 6-bundles classified in twisted nonabelian (differential) cohomology and generalizing the twisted bundles appearing in twisted K-theory. We explain the Green-Schwarz mechanism in heterotic string theory in terms of twisted String 2-bundles and its magnetic dual version in terms of twisted Fivebrane 6-bundles. We close by transgressing differential cocycles to mapping spaces, thereby obtaining their volume holonomies, and show that for Chern-Simons cocycles this yields the action functionals for Chern-Simons theory and its higher dimensional generalizations, regarded as extended quantum field theories.
October 28, 2008
Google Books — More Open Access?
Posted by John Baez
News flash!
A while back, various parties including five companies in the Association of American Publishers sued Google over their ‘Google Book Search’ feature. But now they’ve reached a settlement, which seems likely to affect us all.
Lie Theory Through Examples 4
Posted by John Baez
This week in our seminar we’ll do some examples illustrating how a representation of a simply-connected complex simple Lie group gives rise to a function where is the ‘weight lattice’ of . Wonderfully, this function completely determines the representation (up to equivalence).
In physics, the most famous example is the meson octet, corresponding to the obvious representation of on . It looks like this…
October 26, 2008
This Week’s Finds in Mathematical Physics (Week 271)
Posted by John Baez
In week271 of This Week’s Finds, see massive volcanic eruptions on Jupiter’s moon Io. Learn about allotropes of sulfur, 2d quasicrystals formed by slicing higher-dimensional latices:

and a 4d quasicrystal formed by slicing the lattice. Read about Jeffrey Morton’s wonderful extension of the "groupoidification" idea. And hear what Stephen Summers has to say about new work on constructive quantum field theory!
October 24, 2008
Open Access at the University of California
Posted by John Baez
This fall I became chair of the library committee at U. C. Riverside. I hate committees, but I’m passionate about free world-wide access to scholarly research: journals, books, course materials, and so on. So when the request to head this committee came in my email, I couldn’t honestly duck it.
Groupoidfest 08
Posted by John Baez
I already announced this year’s Groupoidfest, which is being held here at UCR. The schedule won’t be finalized until a couple of weeks before it happens, but you can already see abstracts of some talks:
- Groupoidfest, November 22-23, 2008, Mathematics Department, University of California, Riverside, organized by Aviv Censor. Talk abstracts here.
The talks are roughly divided among three subjects: groupoids and operator algebras, Lie groupoids, and groupoidification. It would be nice if we achieved some communication between these three camps, since there’s room for a lot more interaction than we’re seeing now.
October 23, 2008
Hopf Algebraic Renormalization
Posted by Urs Schreiber
The basic idea and starting point of Hopf algebra methods in renormalization of quantum field theories.
Reviewing Ruelle’s Book
Posted by David Corfield
The November edition of the Notices of the American Mathematical Society is now available, and it includes my review of David Ruelle’s The Mathematician’s Brain.
October 22, 2008
What is Categorification?
Posted by John Baez
Some folks are starting to talk more and more about “categorification”. Others are getting more and more puzzled by what this word means.
Let me tell you what it means.
October 21, 2008
Codescent and the van Kampen Theorem
Posted by Urs Schreiber
It seems threre is a nice general picture which exhibits close relations between the following items
- fundamental -groupoids
- co -stacks
- codescent
- natural differential geometry
- the van Kampen Theorem.
I’ll chat about this and may have some questions, too.
John McKay Visits Kent
Posted by David Corfield
John McKay, of McKay corrrespondence fame, came to speak to us at Kent yesterday. In a hour we were given his views on the past, present and future of the study of finite simple groups. The past was accessible enough, back to Plato and Empedocles, and beyond them to the Scottish stones. We were told to pester the Ashmolean Museum if they are reluctant to show them, since they are obliged to do so.
Naturally, the present and future were more difficult. Before briefly giving you a chain of terms I managed to jot down, a question. What biographical detail connects McKay with Robert Moody?
Lie Theory Through Examples 3
Posted by John Baez
We spent last week catching up with the notes. I decided to spend this week’s seminar explaining how the concept of weight lattice, so important in representations of simple Lie groups and Lie algebras, connects to what we’ve been doing so far. My approach follows that of Frank Adams:
- J. Frank Adams, Lectures on Lie Groups, University of Chicago Press, Chicago, 2004.
This book puts the representation theory of Lie algebras in its proper place: subservient to the Lie groups! At least, that’s the right way to get started. Groups describe symmetries; a Lie algebra begins life as a calculational tool for understanding the corresponding Lie group. Only later, when you become more of an expert, should you dare treat Lie algebras as a subject in themselves.
October 20, 2008
Hepworth on 2-Vector Bundles and the Volume of a Differentiable Stack
Posted by Urs Schreiber
guest post by Bruce Bartlett
Recently, Richard Hepworth gave a seminar at Sheffield:
2-Vector Bundles and the Volume of a Differentiable Stack, (pdf, 9 pages).
Abstract: This seminar is an account of Alan Weinstein’s recent paper The Volume of a Differentiable Stack. I’ll explain that differentiable stacks are a generalization of smooth manifolds and that they crop up in many interesting situations, like the study of orbifolds or the study of flat connections. Just as every manifold has a tangent bundle, every stack has a tangent something, and I’ll explain that the something in question is a bundle of Baez–Crans 2-vector spaces. These 2-vector bundles are often horrible compared with vector bundles, but they still admit a ‘top exterior power’. We’ll see that sections of this top exterior power can be treated just like volume forms on a manifold, and in particular can be integrated to define the volume of a stack.
Categorification in New Scientist
Posted by John Baez
Here’s an article on knot theory that mentions categorification:
- Richard Elwes, Fundamental secrets are tied up in knots, New Scientist, October 15, 2008.
Richard Elwes is a mathematician and reporter based in Leeds, UK.
October 19, 2008
Talk in Göttingen: Second Nonabelian Differential Cohomology
Posted by Urs Schreiber
Tomorrow, October 20th, I’ll talk in Göttingen about
Second nonabelian differential cohomology
pdf notes (5 pages and references)
Morton on 2-Vector Spaces and Groupoids
Posted by John Baez
My student Jeffrey Morton has come out with a paper based on his thesis:
-
Jeffrey Morton, 2-vector spaces and groupoids.
Abstract: This paper describes a relationship between essentially finite groupoids and 2-vector spaces. In particular, we show to construct 2-vector spaces of Vect-valued presheaves on such groupoids. We define 2-linear maps corresponding to functors between groupoids in both a covariant and contravariant way, which are ambidextrous adjoints. This is used to construct a representation — a weak functor — from Span(Gpd) (the bicategory of groupoids and spans of groupoids) into 2Vect. In this paper we prove this and give the construction in detail. It has applications in constructing quantum field theories, among others.
October 16, 2008
Entropy, Diversity and Cardinality (Part 1)
Posted by David Corfield
Guest post by Tom Leinster
This is the first of two posts about
- the difficult problem of how to quantify biodiversity
- the concept of the cardinality of a metric space.
The connection is provided by that important and subtle notion, entropy.
The ideas I’ll present depend crucially on the insights of two people. First, André Joyal explained to me the connection between cardinality and entropy. Then, Christina Cobbold told me about the connection between entropy and biodiversity, and suggested that there might be a direct link between measures of biodiversity and the cardinality of a metric space. She was more right than she knew: it turns out that the cardinality of a metric space, which I’d believed to be a new concept coming from enriched category theory, was discovered 15 years ago by ecologists!
October 14, 2008
String- and Fivebrane-Structures
Posted by Urs Schreiber
Today appeared
Christopher L. Douglas, André G. Henriques, Michael A. Hill
Homological obstructions to string orientations
(arXiv)
which presents a new method to check if a given manifold admits a String-structure.
Freely Generated ω-Categories
Posted by Urs Schreiber
I try to review some aspects of some of the literature on -categories which are “freely generated”. Then I have some questions.
October 13, 2008
The Nature of Time
Posted by John Baez
The Foundational Questions Institute is having an essay contest on The Nature of Time. The top prize is $10,000, the second prize is $5,000, and so on.
It’s a fascinating topic, but I can’t say I’m thrilled with most of the essays. In fact, that’s a polite way of expressing my feelings, in keeping with the civil atmosphere of this café. I’ll mention my favorite essay below, and keep quiet about the worst.
Maybe you could do better. In fact, maybe you should give it a try! Just make sure to submit your essay before December 1st, 2008.
I would write one myself, but I don’t have… time.
October 12, 2008
This Week’s Finds in Mathematical Physics (Week 270)
Posted by John Baez
In week270 of This Week’s Finds, see lava flowing on Jupiter’s moon Io.
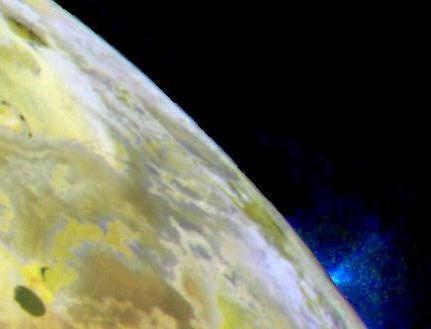
Hear about Greg
Egan’s brand new novel, Incandescence. Then - see talks about
my favorite three numbers. Read about the Mathieu group M12.
Learn how each regular polytope with 5-fold rotational symmetry is
secretly linked to a lattice in a space of twice as many dimensions.
And see a simple but surprising property of the number 12.
October 8, 2008
Semistrict Infinity-Categories and ω-Semi-Categories
Posted by Urs Schreiber
What is a semistrict -category? The strictest possible version of weak -category which is still general enough to satisfy the homotopy hypothesis?
Carlos Simpson in Homotopy types of strict 3-groupoids conjectured (on p. 27) that one remarkable answer is: -categories with strict composition but possibly weak identities – “-snucategories” (strict, non-unital).
Joachim Kock in Weak identity arrows in higher categories remarked that
The conjecture in its strong form has startling consequences, defying all trends in higher category theory: every weak higher category should be equivalent to one with strict composition!
and set out to formulate the conjecture in terms of the notion he calls a fair -category inspired by Tamsamani’s -categories. With Joyal he then proved the conjecture up to (with some restrictions) in Weak units and homotopy 3-types.
A little later Simona Paoli in Semistrict Tamsamani -groupoids and connected -types proposed notions of semistrict Tamsamani -categories, showed (as mentioned in TWF 245) that they do satisfy the homotopy hypothesis, and conjectured (p. 69) that one of these versions “corresponds” to Kock’s “fair -categories”.
Of course there may not be the semistrict version of -categories, and different choices may be useful for different purposes, as remarked in the very last two paragraphs on p. 22,23 of E. Cheng and N. Gurski’s The periodic table of n-categories for low dimensions I.
New Directions in the Philosophy of Mathematics
Posted by David Corfield
To celebrate the founding of MIMS, the mathematics department of the recently unified Manchester University, it was proposed that various workshops named ‘New Directions in…’ be run. They kindly agreed to allow Alexandre Borovik and me to organise one of these workshops on the Philosophy of Mathematics.
So, on Saturday 4 October, we began with Mary Leng, a philosopher at Liverpool, talking about whether the creation of mathematical theories, e.g., Hamilton’s quaternions, gives us any more reason to think mathematical entities exist than does the discovery of new consequences within existing theories. She concluded that it does not – both concern the drawing of consequences from suppositions, e.g., “Were there to be a 3 or 4-dimensional number system sharing specified properties with the complex numbers, then…”.
October 7, 2008
Yet Another Model ω-Question
Posted by Urs Schreiber
In the context of -Lie theory one wants to relate smooth -groupoids with -Lie-algebroids. The relation between the two seems to be induced by two adjunctions: the first relates -groupoids to spaces, the second relates spaces to -Lie algebroids.
With this in mind, it is interesting to compare the two standard model category structures on -groupoids and on -Lie algebroids (i.e. on DGCAs). It seems to me as if there is a chance that the model structure on -Lie algebroids is induced from transporting that on -groupoids through these two adjunctions. Which would nicely fit into the picture.
But is it true? Or something similar?
Below I repeat the question with all technical details given.
Lie Theory Through Examples 2
Posted by John Baez
In this week’s seminar on Lie Theory Through Examples, we’ll move on up to the lattice.
You’ve seen this lattice before: when you stack spheres in a triangular pyramid, their centers lie at points that form a lattice of this sort:

But to derive this lattice starting with the Lie group , we’ll need to talk a bit about the Killing form. That’s what allows us to measure angles and distances in the Lie algebra.
October 5, 2008
The Blog of Fun
Posted by David Corfield
October 2, 2008
More Model ω-Questions
Posted by Urs Schreiber
Here are some further questions related to model category theory in general and the folk model structure on -categories in particular, concerned with
- fibrant objects in -categories;
- model structure on -groupoids and crossed complexes;
- general question about weak universal property of homotopy (co)limits.
October 1, 2008
Mathematical Reality
Posted by David Corfield
Here is the draft of paper I’m contributing to a book on the work of Albert Lautman – Lautman and the Reality of Mathematics. I’m going to be talking about it this Saturday at a workshop I’m jointly organising. Comments are welcome.
Choosing the title for this post reminded me of a post with the same title written for my old blog, which is no longer hosted anywhere. I wrote back in December 2005:
 Posts with this logo use
Posts with this logo use 














