Concepts of Sameness (Part 4)

February 27, 2015

Posted by John Baez

$MathML-enabled post (click for more details).$

This time I’d like to think about three different approaches to ‘defining equality’, or more generally, introducing equality in formal systems of mathematics.

These will be taken from old-fashioned logic — before computer science, category theory or homotopy theory started exerting their influence. Eventually I want to compare these to more modern treatments.

If you know other interesting ‘old-fashioned’ approaches to equality, please tell me!

$MathML-enabled post (click for more details).$

The equals sign is surprisingly new. It was never used by the ancient Babylonians, Egyptians or Greeks. It seems to originate in 1557, in Robert Recorde’s book The Whetstone of Witte. If so, we actually know what the first equation looked like:

As you can see, the equals sign was much longer back then! He used parallel lines “because no two things can be more equal.”

Formalizing the concept of equality has raised many questions. Bertrand Russell published The Principles of Mathematics [R] in 1903. Not to be confused with the Principia Mathematica, this is where he introduced Russell’s paradox. In it, he wrote:

identity, an objector may urge, cannot be anything at all: two terms plainly are not identical, and one term cannot be, for what is it identical with?

In his Tractatus, Wittgenstein [W] voiced a similar concern:

Roughly speaking: to say of two things that they are identical is nonsense, and to say of one thing that it is identical with itself is to say nothing.

These may seem like silly objections, since equations obviously do something useful. The question is: precisely what?

Instead of tackling that head-on, I’ll start by recalling three related approaches to equality in the pre-categorical mathematical literature.

The indiscernibility of identicals

The principle of indiscernibility of identicals says that equal things have the same properties. We can formulate it as an axiom in second-order logic, where we’re allowed to quantify over predicates $P$ :

$\forall x \forall y [x = y \; \implies \; \forall P \, [P(x) \; \iff \; P(y)] ]$

We can also formulate it as an axiom schema in 1st-order logic, where it’s sometimes called substitution for formulas. This is sometimes written as follows:

For any variables $x, y$ and any formula $\phi$ , if $\phi'$ is obtained by replacing any number of free occurrences of $x$ in $\phi$ with $y$ , such that these remain free occurrences of $y$ , then

$x = y \;\implies\; [\phi \;\implies\; \phi' ]$

I think we can replace this with the prettier

$x = y \;\implies\; [\phi \;\iff \; \phi']$

without changing the strength of the schema. Right?

We cannot derive reflexivity, symmetry and transitivity of equality from the indiscernibility of identicals. So, this principle does not capture all our usual ideas about equality. However, as shown last time, we can derive symmetry and transitivity from this principle together with reflexivity. This uses an interesting form of argument where take “being equal to $z$ ” as one of the predicates (or formulas) to which we apply the principle. There’s something curiously self-referential about this. It’s not illegitimate, but it’s curious.

The identity of indiscernibles

Leibniz [L] is often credited with formulating a converse principle, the identity of indiscernibles. This says that things with all the same properties are equal. Again we can write it as a second-order axiom:

$\forall x \forall y [ \forall P [ P(x) \; \iff \; P(y)] \; \implies \; x = y ]$

or a first-order axiom schema.

We can go further if we take the indiscernibility of identicals and identity of indiscernibles together as a package:

$\forall x \forall y [ \forall P [ P(x) \; \iff \; P(y)] \; \iff \; x = y ]$

This is often called the Leibniz law. It says an entity is determined by the collection of predicates that hold of that entity. Entities don’t have mysterious ‘essences’ that determine their individuality: they are completely known by their properties, so if two entities have all the same properties they must be the same.

This principle does imply reflexivity, symmetry and transitivity of equality. They follow from the corresponding properties of $\iff$ in a satisfying way. Of course, if we were wondering why equality has these three properties, we are now led to wonder the same thing about the biconditional $\iff$ . But this counts as progress: it’s a step toward ‘logicizing’ mathematics, or at least connecting $=$ firmly to $\iff$ .

Apparently Russell and Whitehead used a second-order version of the Leibniz law to define equality in the Principia Mathematica [RW], while Kalish and Montague [KL] present it as a first-order schema. I don’t know the whole history of such attempts.

When you actually look to see where Leibniz formulated this principle, it’s a bit surprising. He formulated it in the contrapositive form, he described it as a ‘paradox’, and most surprisingly, it’s embedded as a brief remark in a passage that would be hair-curling for many contemporary rationalists. It’s in his Discourse on Metaphysics, a treatise written in 1686:

Thus Alexander the Great’s kinghood is an abstraction from the subject, and so is not determinate enough to pick out an individual, and doesn’t involve the other qualities of Alexander or everything that the notion of that prince includes; whereas God, who sees the individual notion or ‘thisness’ of Alexander, sees in it at the same time the basis and the reason for all the predicates that can truly be said to belong to him, such as for example that he would conquer Darius and Porus, even to the extent of knowing a priori (and not by experience) whether he died a natural death or by poison — which we can know only from history. Furthermore, if we bear in mind the interconnectedness of things, we can say that Alexander’s soul contains for all time traces of everything that did and signs of everything that will happen to him — and even marks of everything that happens in the universe, although it is only God who can recognise them all.

Several considerable paradoxes follow from this, amongst others that it is never true that two substances are entirely alike, differing only in being two rather than one. It also follows that a substance cannot begin except by creation, nor come to an end except by annihilation; and because one substance can’t be destroyed by being split up, or brought into existence by the assembling of parts, in the natural course of events the number of substances remains the same, although substances are often transformed. Moreover, each substance is like a whole world, and like a mirror of God, or indeed of the whole universe, which each substance expresses in its own fashion — rather as the same town looks different according to the position from which it is viewed. In a way, then, the universe is multiplied as many times as there are substances, and in the same way the glory of God is magnified by so many quite different representations of his work.

(Emphasis mine — you have to look closely to find the principle of identity of indiscernibles, because it goes by so quickly!)

There have been a number of objections to the Leibniz law over the years. I want to mention one that might best be handled using some category theory. In 1952, Max Black [B] claimed that in a symmetrical universe with empty space containing only two symmetrical spheres of the same size, the two spheres are two distinct objects even though they have all their properties in common.

As Black admits, this problem only shows up in a ‘relational’ theory of geometry, where we can’t say that the spheres have different positions — e.g., one centered at the points $(x,y,z)$ , the other centered at $(-x,-y,-z)$ — but only speak of their position relative to one another. This sort of theory is certainly possible, and it seems to be important in physics. But I believe it can be adequately formulated only with the help of some category theory. In the situation described by Black, I think we should say the spheres are not equal but isomorphic.

As widely noted, general relativity also pushes for a relational approach to geometry. Gauge theory, also, raises the issue of whether indistinguishable physical situations should be treated as equal or merely isomorphic. I believe the mathematics points us strongly in the latter direction.

A related issue shows up in quantum mechanics, where electrons are considered indistinguishable (in a certain sense), yet there can be a number of electrons in a box — not just one.

But I will discuss such issues later.

Extensionality

In traditional set theory we try to use sets as a substitute for predicates, saying $x \in S$ as a substitute for $P(x)$ . This lets us keep our logic first-order and quantify over sets — often in a universe where everything is a set — as a substitute for quantifying over predicates. Of course there’s a glitch: Russell’s paradox shows we get in trouble if we try to treat every predicate as defining a set! Nonetheless it is a powerful strategy.

If we apply this strategy to reformulate the Leibniz law in a universe where everything is a set, we obtain:

$\forall S \forall T [ S = T \; \iff \; \forall R [ S \in R \; \iff \; T \in R]]$

While this is true in Zermelo-Fraenkel set theory, it is not taken as an axiom. Instead, people turn the idea around and use the axiom of extensionality:

$\forall S \forall T [ S = T \; \iff \; \forall R [ R \in S \; \iff \; R \in T]]$

Instead of saying two sets are equal if they’re in all the same sets, this says two sets are equal if all the same sets are in them. This leads to a view where the ‘contents’ of an entity as its defining feature, rather than the predicates that hold of it.

We could, in fact, send this idea back to second-order logic and say that predicates are equal if and only if they hold for the same entities:

$\forall P \forall Q [\forall x [P(x) \; \iff \; Q(x)] \; \iff P = Q ]$

as a kind of ‘dual’ of the Leibniz law:

$\forall x \forall y [ \forall P [ P(x) \; \iff \; P(y)] \; \iff \; x = y ]$

I don’t know if this has been remarked on in the foundational literature, but it’s a close relative of a phenomenon that occurs in other forms of duality. For example, continuous real-valued functions $F, G$ on a topological space obey

$\forall F \forall G [\forall x [F(x) \; = \; G(x)] \; \iff F = G ]$

but if the space is nice enough, continuous functions ‘separate points’, which means we also have

$\forall x \forall y [ \forall F [ F(x) \; = \; F(y)] \; \iff \; x = y ]$

Notes

[B] Max Black, The identity of indiscernibles, Mind, New Series 61 (1952), 153–164.
[F] Peter Forrest, The identity of indiscernibles, Stanford Encyclopedia of Philosophy.
[KL] Donald Kalish and Richard Montague, Logic: Techniques of Formal Reasoning, Oxford U. Press, Oxford, 1964, pp. 220-221.
[L] Gottfried Wilhelm Leibniz, Discourse on Metaphysics, trans. Jonathan Bennett.
[R] Bertrand Russell, Principles of Mathematics § 64, 1903.
[RW] Bertrand Russell and Alfred North Whitehead, Principia Mathematica, ☆13, 1910.
Wikipedia, Identity of indiscernibles.
[W] Ludwig Wittgenstein, Tractatus Logico-Philosophicus 5.5303, ed. C. K. Ogden, Routledge & Kegan Paul, 1922.

Posted at February 27, 2015 10:21 AM UTC

TrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/2810

32 Comments & 0 Trackbacks

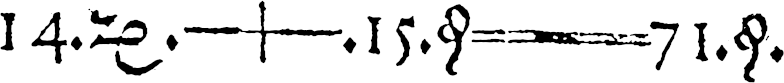
Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

I don’t think the schemas $x = y \Rightarrow (\phi \Rightarrow \phi')$ and $x = y \Rightarrow (\phi \Leftrightarrow \phi')$ are equivalent in the absence of symmetry of $=$ .

It looks to me like the latter schema entails symmetry of $=$ (and so transitivity of $=$ as well). Both of the following are instances of it: $\begin{aligned} x = y &\Rightarrow (x = x \Leftrightarrow x = y)\\ x = y &\Rightarrow (x = x \Leftrightarrow y = x)\\ \end{aligned}$ But then we can get $x = y \Rightarrow (x = y \Leftrightarrow y = x)$ , and so $x = y \Rightarrow y = x$ , without any appeal to reflexivity of $=$ , by using the symmetry and transitivity of $\Leftrightarrow$ .

(Alternately, from the first listed instance alone, we can get $x = y \Rightarrow x = x$ ; this isn’t reflexivity, but it’s close enough to get the job done.)

Posted by: Dave Ripley on February 27, 2015 4:16 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Thanks! I’ll straighten all these things out eventually, with help from anyone who enjoys these puzzles.

I was hoping that this axiom:

Substitution for formulas. For any variables $x, y$ and any formula $\phi$ , if $\phi'$ is obtained by replacing any number of free occurrences of $x$ in $\phi$ with $y$ , such that these remain free occurrences of $y$ , then

$x = y \;\implies\; [\phi \;\implies\; \phi' ]$

implies this:

For any variables $x, y$ and any formula $\phi$ , if $\phi'$ is obtained by replacing any number of free occurrences of $x$ in $\phi$ with $y$ , such that these remain free occurrences of $y$ , then

$x = y \;\implies\; [\not{\phi} \;\implies\; \not\phi' ]$

In classical logic, at least, these two together give

For any variables $x, y$ and any formula $\phi$ , if $\phi'$ is obtained by replacing any number of free occurrences of $x$ in $\phi$ with $y$ , such that these remain free occurrences of $y$ , then

$x = y \;\implies\; [\phi \;\iff\; \phi' ]$

Am I making a mistake somewhere?

Posted by: John Baez on February 27, 2015 4:12 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

If you’re making a mistake, then I don’t see it either. Does that mean that in the proof of symmetry and transitivity from indiscernibility of identicals, we could use classical logic to substitute for reflexivity?

Posted by: Mike Shulman on February 27, 2015 7:00 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Mike wrote:

Does that mean that in the proof of symmetry and transitivity from indiscernibility of identicals, we could use classical logic to substitute for reflexivity?

How would that go? I don’t see how.

(I may have more intuition than you for what weird tricks you can and cannot do using the exotic rules of classical logic. Or perhaps you’re seeing a trick I’m not.)

Posted by: John Baez on February 27, 2015 10:24 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Awesome! I think that does work, so the schemas are equivalent. (Whoops! Sorry.) But I also think the derivation of symmetry (and so transitivity) that I pointed to still works, too. So I reckon we can use classical logic’s weird tricks to get symmetry (and so transitivity, by the suggestion in post Part 3) from “just” $x = y \Rightarrow (\phi \Rightarrow \phi')$ , by combining ideas.

Here’s a sketch: $\begin{aligned} x = y &\Rightarrow (\neg x = x \Rightarrow \neg x = y)\\ x = y &\Rightarrow (x = y \Rightarrow x = x)\\ x = y &\Rightarrow x = x\\ x = y &\Rightarrow (x = x \Rightarrow y = x)\\ x = y &\Rightarrow y = x\\ \end{aligned}$

First and fourth lines are instances of the one-way substitution schema. Second line follows from the first classically. Third follows from the second in a variety of logics. Fifth follows from third and fourth in a variety of logics.

Posted by: Dave Ripley on February 27, 2015 11:05 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Yes, that’s what I had in mind.

Posted by: Mike Shulman on February 28, 2015 12:03 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Great!

Posted by: John Baez on February 28, 2015 12:23 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

I’ve always been rather puzzled by Recorde’s remark that “no two things can be more equal” than a pair of parallel lines. It seems to me that a pair of parallel lines would obviously be more equal if they were the same line. This seems related to your favorite remark that the only interesting equations are false ones.

One question: I don’t see how to deduce symmetry and transitivity from identity of indiscernibles in the absence of indiscernibility of identicals; can you explain?

And a thought: it’s not clear to me that the Leibniz law means that “entities don’t have mysterious ‘essences’ that determine their individuality”, given that (as you remarked earlier on) we can use equality itself as one of the properties to which the Leibniz law applies. E.g. it seems to me that might be the case that two entities $x$ and $y$ have all the same properties except for the properties “is equal to $x$ ” and “is equal to $y$ ”, of which $x$ has the first but not the second and $y$ has the second but not the first. In that case $x\neq y$ , but I think one could argue that this distinction is being made only by some “mysterious essence” (although to be honest, I don’t personally find it very mysterious).

Oh, and another thought: as you probably know, in type theory, $\forall x[P(x)\Leftrightarrow Q(x)] \;\Leftrightarrow\; P=Q$ is a manifestation of univalence. It decomposes into function extensionality (which follows from univalence) and the special case of univalence for propositions; the latter has been known since (I think) Russell by the name “propositional extensionality”.

Posted by: Mike Shulman on February 27, 2015 5:59 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Yes, one line is more equal itself than two lines are to each other, but what it isn’t is two.

Posted by: Jesse C. McKeown on February 27, 2015 6:07 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

No two lines could be more different than parallel lines, since these have no points in common.

Posted by: John Baez on February 27, 2015 6:59 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

But they at least have the same direction, whereas lines that intersect in the plane can’t have the same direction, and thus are distinguishable by direction.

Posted by: Layra on February 27, 2015 8:33 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

It is hard to draw the line with two origins convincingly

Posted by: Jesse C. McKeown on February 28, 2015 9:06 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Well, I actually just got through telling my intro-logic students that in mathematics, when we talk about “two things”, we don’t exclude the possibility that they are equal. E.g. “suppose given two integers $x$ and $y$ …”. But maybe that careful use of language is more recent than the equals sign.

Posted by: Mike Shulman on February 27, 2015 11:01 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

I wouldn’t say that one usage or the other is more careful; just that they’re differently careful. In the modern usage one sometimes has to say “two distinct Ws” and in the older usage sometimes “two (perhaps identical) Ws” or some such. Of course, with variable names, one doesn’t need to mention “two”: it is sufficient to say “Let $x$ and $y$ be [different $XOR$ perhaps identical] Ws”, and then off we go.

Posted by: Jesse C. McKeown on February 28, 2015 9:17 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Mike wrote:

I’ve always been rather puzzled by Recorde’s remark that “no two things can be more equal” than a pair of parallel lines. It seems to me that a pair of parallel lines would obviously be more equal if they were the same line.

Good point! So we should write equality as $-$ .

One question: I don’t see how to deduce symmetry and transitivity from identity of indiscernibles in the absence of indiscernibility of identicals; can you explain?

Whoops! Fixed.

And a thought: it’s not clear to me that the Leibniz law means that “entities don’t have mysterious ‘essences’ that determine their individuality”, given that (as you remarked earlier on) we can use equality itself as one of the properties to which the Leibniz law applies.

Good point. I’m quite sure a lot of philosophers believe that

entities don’t have mysterious ‘essences’ that determine their individuality: they are completely known by their properties, so if two entities have all the same properties they must be the same…

but maybe a bunch didn’t notice that unless you’re very careful to exclude it, “being equal to $x$ ” counts as a property. I’ll have to mention this.

Posted by: John Baez on February 27, 2015 4:32 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

This last issue crops up in metaphysical discussions of what to make of a universe containing two identical spheres. This was brought up by Max Black as discussed here.

Posted by: David Corfield on March 2, 2015 10:39 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Here’s a slightly different second-order definition from the usual Leibniz-Russell one, and which is quite intuitive. Suppose $D$ is some domain of things, and consider its identity relation, that is the diagonal, the set of ordered pairs $(a,a)$ with $a \in D$ . This is reflexive. So, let $R \subseteq D^2$ be a reflexive relation. $R$ has the identity relation as a subset; but contains a lot of “off-diagonal” stuff - pairs $(c,d)$ where $c \neq d$ . To get rid of this, take the smallest such reflexive relation, and we see that the identity relation on $D$ is the smallest reflexive relation on $D$ .

This definition is second-order, quantifying over relations: intuitively, $x =y$ if and only if, for any reflexive $R$ , $R(x,y)$ holds. So, more formally,

$x = y \leftrightarrow \forall R(\forall z R(z,z) \rightarrow R(x,y))$ .

Posted by: Jeffrey Ketland on March 2, 2015 10:48 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Thanks, Jeffrey, for this other second-order definition of equality! I’m in the mood for collecting definitions of equality. Do you have a reference to this, or it is your own spur-of-the-moment invention?

Posted by: John Baez on March 2, 2015 10:57 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

John, it’s in a paper I wrote about 10 years ago, but not published until 2011, “Identity and Indiscernibility”, Review of Symbolic Logic, along with a lot of information about the conditions for defining $=$ for relational structures $A$ .

Posted by: Jeffrey Ketland on March 2, 2015 11:13 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

That’s actually also the same as Martin-Lof’s definition of the identity type, under the usual impredicative encoding of inductive types.

Posted by: Mike Shulman on March 3, 2015 12:22 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Thanks for the comment! I mentioned Black and his spheres in my post… but there I began to argue that the correct solution is to say the two spheres are isomorphic, not equal. Or, more precisely: the symmetry of that universe that switches the two sphere is an isomorphism, but not the identity. It seems to me that we need the concept of isomorphism to correctly handle universes with symmetries (like ours).

Posted by: John Baez on March 2, 2015 10:50 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

John, here’s something (a result) connected to that. Let $A$ be a relational structure but do not assume $=$ is definable in $A$ . For $a,b$ elements of $dom(A)$ , we can define a notion of $a,b$ being “indiscernible” in $A$ , which write as: $a \sim_{A} b$ . Let $\pi_{ab} : dom(A) \to dom(A)$ be the transposition swapping $a$ and $b$ . Then we have:

$a \sim_A b \rightarrow \pi_{ab} \in Aut(A)$

So, if $a$ and $b$ are indiscernible (in $A$ ), then their transposition is an automorphism of $A$ .

Posted by: Jeffrey Ketland on March 2, 2015 11:08 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

John, another example that might be interesting for your paper if you want a case where identity is not definable: i.e., a relational structure $A$ where the identity relation $=_A$ is not (first-order) definable in $A$ . Let $A$ be the structure $(D,R)$ , with $D = \{0,1\}$ and $R = \{(0,0),(0,1),(1,0),(1,1)\}$ . We do not assume $x = y$ as a primitive predicate. Assume the predicate symbol denoting $R$ is $P$ . I.e., $R = P^A$ . The formula $x \sim_A y$ expressing indiscernibility is:

$\forall z (P(x,z) \leftrightarrow P(y,z)) \wedge \forall z (P(z,x) \leftrightarrow P(z,y))$ .

This is the strongest first-order way of discerning objects if $x = y$ is not taken as a primitive.

Then: the identity relation $=_A$ is not definable in $A$ .

To show this, first, one can see that $0$ and $1$ are indiscernible in $A$ : i.e., $0 \sim_A 1$ . But if $=_A$ were definable, then the formula $x \sim_A y$ would define it. So, $0 = 1$ . Contradiction.

Posted by: Jeffrey Ketland on March 3, 2015 9:41 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Did Robert Recorde replace an english word for being equal? Like it seems that for example http://en.wikipedia.org/wiki/Gerolamo_Cardano used the word aequalis where you would now place an equal sign.

John cited:

Several considerable paradoxes follow from this, amongst others that it is never true that two substances are entirely alike, differing only in being two rather than one. It also follows that a substance cannot begin except by creation, nor come to an end except by annihilation; …

I have problems to understand Leibniz in the way he is supposed to be understood. I first thought eventually my english is so bad, but then it seems also my french is at least equally bad, that is I think the french to english translation is approximately right (apart from the fact that it left away that passage about Thomas von Aquin), but I still understand it not as I am supposed to. This seems to be a transcript of the original (I haven’t found a scan):

Il[s’ensuiventdecela] plusieurs paradoxes considerables,comme entre autres qu’il n’est pas vray,que deux substances se ressemblent entierement et soyent differentes solo numero, et que ce que S. Thomas asseure sur ce point des anges ou intelligences (quod ibi omne individuum sit species infima) est vray de toutes les substances, pourveu qu’on prenne la difference specifique, comme la prennent les Geometres a l’egard de leur figures. Item qu’aucune substance ne scauroit commencer que par creation, ny perir que par annihilation.

That is I understand this sentence:

it is never true that two substances are entirely alike, differing only in being two rather than one.

as:

If two things differ only in being two rather than one (which I interpret as x=y) then they are never entirely alike (i.e. then it is not true that P(x) = P(y) for all P), which seems to me quite different from what you claim is the “Leibniz law”:

if two entities have all the same properties they must be the same.

?????

These kind of discussions seemed to have been popular at that times in Europe, like Paracelsus is supposed to have said (this link cites Paracelsus, Gesammelte Werke, Aschner-Ausgabe Bd. IV S. 339):

denn Gott hat am Anfang alle Dinge sorgfältig unterschieden und keinem eine Gestalt und Form wie dem anderen gegeben, sondern jedem eine Schelle angehängt, wie man sagt: “Man erkennt den Naren an der Schelle.”

Posted by: nad on February 27, 2015 10:30 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Nad wrote:

I understand this sentence:

it is never true that two substances are entirely alike, differing only in being two rather than one.

as:

If two things differ only in being two rather than one (which I interpret as $x=y$ ) then they are never entirely alike (i.e. then it is not true that $P(x) = P(y)$ for all $P$ ).

To me it seems you’ve dramatically changed the meaning of the sentence. To me this sentence:

It is never true that two substances are entirely alike, differing only in being two rather than one.

means the same thing as

It is never true that two substances are entirely alike yet differ by being two rather than one.

or in other words:

It is never true that two substances share every property but are not equal.

or in other words:

It is never true that $x, y$ have $P(x) \iff P(y)$ for all predicates $P$ but $x \ne y$ .

or in other words:

$\not \exists x \exists y [\forall P [P(x) \iff P(y)] \; \& \; x \ne y ]$

This is another way of stating the so-called Leibniz law

$$ \forall x \forall y [\forall P [P(x) \iff P(y)] \; \implies \; x = y ]

Posted by: John Baez on February 27, 2015 3:24 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Well you are the native speaker, so I try to believe, but I still have troubles with this. You wrote:

To me it seems you’ve dramatically changed the meaning of the sentence. To me this sentence:

It is never true that two substances are entirely alike, differing only in being two rather than one.

means the same thing as

It is never true that two substances are entirely alike yet differ by being two rather than one.

or in other words:

It is never true that two substances share every property but are not equal.

If two substances differ (only) by being two (substances) rather then one substance, then this doesn’t sound to me as if they are unequal (substances). But -as said- you are the native speaker.

I could eventually remotely get your interpretation with the french original, because I don’t fully know the expression “soyent differentes solo numero”, this seems to be 17th century french or may be some artificial (science-speak) dialect ? (I interprete soyent, as some form of “being” but solo seems italian, where it means alone, is “solo” an old form of “sauf”?). That is if you would leave away the “solo numero” then it seems one would have your meaning, but can one leave it away??…it seems to me one can not, but then as said my french is way worse than my english (I never spend a long time in France and my school french lies back 30 years)

That is on a first guess I would tend to translate:

que deux substances se ressemblent entierement et soyent differentes solo numero,

“that two substances completely resemble each other while being different only by their enumberation”…. OR even…but this would give again a new meaning and I don’t really think that this is the right translation:…. “that two substances completely resemble each other while being different only/alone by their cardinality”

the first translation would approximately be like the english translation you cited.

Posted by: nad on February 27, 2015 7:08 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Nad wrote:

If two substances differ (only) by being two (substances) rather then one substance, then this doesn’t sound to me as if they are unequal (substances).

I feel quite sure that in Leibniz’s old way of talking, “being two” implies “being not one” which implies “being unequal”.

In slightly more modern language, the Stanford Encyclopedia of Philosophy says:

The Identity of Indiscernibles is a principle of analytic ontology first explicitly formulated by Wilhelm Gottfried Leibniz in his Discourse on Metaphysics, Section 9 (Loemker 1969: 308). It states that no two distinct things exactly resemble each other. This is often referred to as ‘Leibniz’s Law’ and is typically understood to mean that no two objects have exactly the same properties.

[…]

The Identity of Indiscernibles (hereafter called the Principle) is usually formulated as follows: if, for every property F, object x has F if and only if object y has F, then x is identical to y. Or in the notation of symbolic logic:

∀F(Fx ↔ Fy) → x=y.

This formulation of the Principle is equivalent to the Dissimilarity of the Diverse as McTaggart called it, namely: if x and y are distinct then there is at least one property that x has and y does not, or vice versa.

Posted by: John Baez on February 27, 2015 10:30 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Ok. I am still not convinced but I certainly don’t want to make public bets of my 30 year old school french against the Stanford Encyclopedia of Philosophy. I am not such a fan of this symbolic logic, but I currently still think he said:

$\neg \forall x \forall y [x \simeq y \Rightarrow \forall P[P(x) = P(y)]]$

$\exists x \exists y[x \simeq y \wedge \exists P[P(x) \neq P(y)]]$

where I now wrote a $\simeq$ instead of $=$ in order to better account for this “solo numero” (which by the way could also be latin, but probably meaning the same as the italian). I know that the $\simeq$ is a no-no in symbolic logic but I currently can’t think of another way to express this more visibly. In principle $x=y$ should somewhat suffice, since x and y are denoted by two different letters, but I keep it now like this). That is with his “solo numero” he is rather speaking of something you prefer to call an isomorphism.

So I think what he had in mind is in some sense similar to Dedekind objections with regard to Zermelo-Fraenkel’s set theory (I think we had this discussion somewhere on Azimuth, but I can’t find it). That is one can interprete Dedekind in such a way that he may have favoured (also) a theory for what is now commonly called a Multiset (I.e. a “set” where you can have identical elements, which may e.g. differ by something which isn’t given by the properties of the set.) And by what I have sofar understood this principle seems also what you prefer to assemble under the term “categorification”, that is you look for structures which give in some “limit” or in some “glossing procedure” (“decategorification”), like e.g. coarse graining a known or given mathematical equality.

I should also point out that my interpretation of Leibniz is not solely due to the translation but also to the context he presents in section 8, i.e. the paragraph in your citation:

Furthermore, if we bear in mind the interconnectedness of things, we can say that Alexander’s soul contains for all time traces of everything that did and signs of everything that will happen to him — and even marks of everything that happens in the universe, although it is only God who can recognise them all.

and the overall discussion at that time like the aforementioned Doctrine of signatures.

Posted by: nad on February 28, 2015 8:42 AM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

$MathML-enabled post (click for more details).$

Nad wrote:

Did Robert Recorde replace an english word for being equal?

According to the MacTutor website,

The symbol ‘=’ was not immediately popular. The symbol || was used by some, and æ (or œ), from the Latin word aequalis meaning equal, was widely used into the 1700s.

Posted by: John Baez on February 27, 2015 3:47 PM | Permalink | Reply to this

$MathML-enabled post (click for more details).$

Does it help to reformulate equality as “sharing the same properties”? I don’t think so. Properties is related to “truth value”, but truth is hardly definable. It might even be harder to define what is true than what is equal in a certain sense.

What is a property, before all?

Posted by: sure on March 5, 2015 5:14 PM | Permalink | Reply to this

Re:

$MathML-enabled post (click for more details).$

By the way, if the equivalence between indiscernibles and identicals holds true for all propositions without considering the proposition $P(x=y)$ “ $x$ is equal to $y$ ”, it still holds for the proposition $P(P(x=y))$ = “the proposition “ $x$ is equal to $y$ ” is true”. So you would have to reject all $P^n(x=y)$ for $n \geq 1$ and all their negations if you’re a constructivist… Even worse, you would also have to reject the propositions that decides if $x=y$ , say, $Dec(x=y)$ “there exists a proof of $x = y$ or there exists a proof of $x \neq y$ ”, because this would mean that you can explicitly show by using the equivalence that all propositions hold true, in particular the ones you want to reject. Obviously, also all $Dec^n(x=y)$ and so on. Where do we stop? Is there even an end to this rejection process?

I think that this way of reasoning is actually totally ill defined: one needs to type propositions. A proposition about a proposition is of different type than a proposition about an “object”. There is different layers of logical deduction/reasoning, and mixing them up makes you vulnerable to diagonal arguments and all this kind of infinitary digression. Moreover, the distinction between “being an object” or “being a proposition about an object” is also ill-defined. The $n$ -propositions about $m$ -propositions with $m$ < $n$ takes as objects propositions, not what we would consider naively as “real objects”. There is no such thing as “real objects”, even in ZF(C), what we call integers are nothing else than pure syntax, that is, some true propositions for the formal system.

Posted by: sure on March 5, 2015 5:37 PM | Permalink | Reply to this

Re: Concepts of Sameness (Part 4)

Re: “Furthermore, if we bear in mind the interconnectedness of things, we can say that Alexander’s soul contains for all time traces of everything that did and signs of everything that will happen to him — and even marks of everything that happens in the universe, although it is only God who can recognise them all.”

The n-Category Café

Skip to the Main Content

February 27, 2015