This Week's Finds in Mathematical Physics (Week 281)

October 20, 2009

This Week’s Finds in Mathematical Physics (Week 281)

Posted by John Baez

$MathML-enabled post (click for more details).$

In week281 of This Week’s Finds, learn about the newly discovered ring of Saturn — the Phoebe ring — and how it explains the mystery of Iapetus.

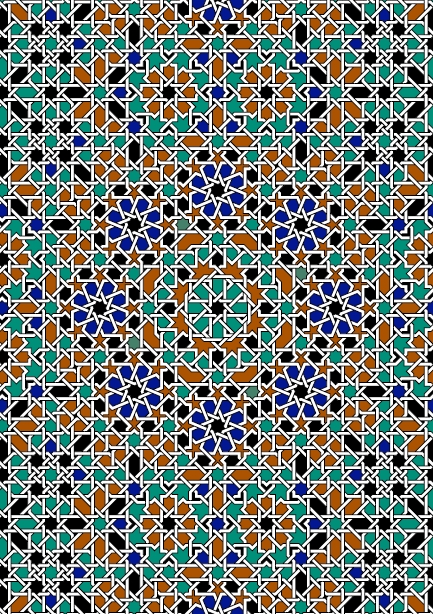
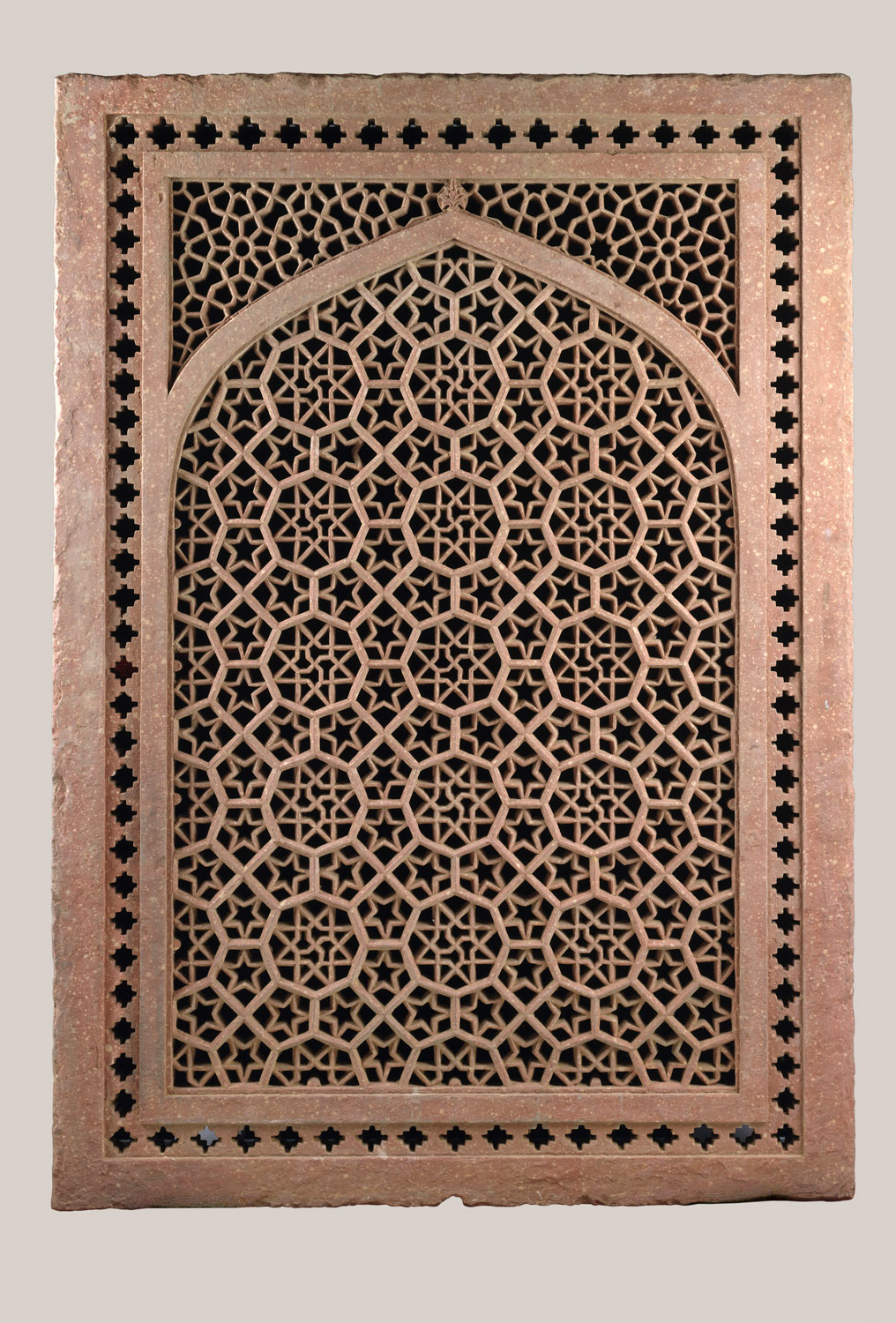
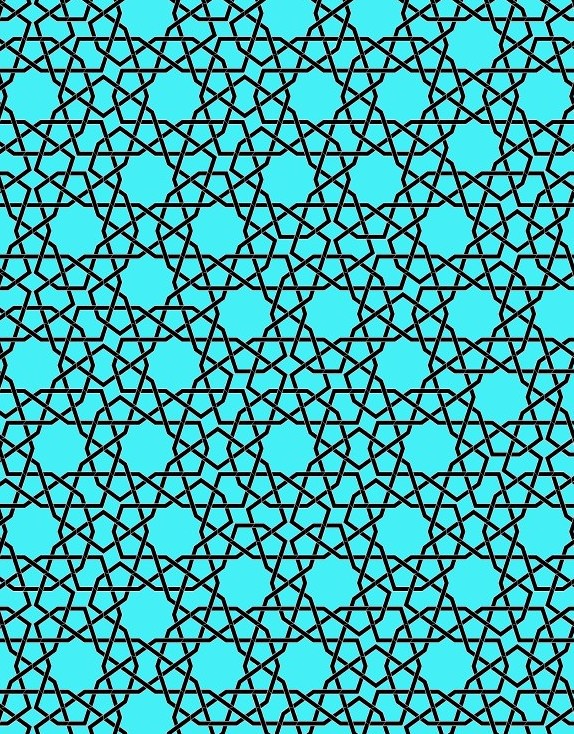
See Egan’s new applet that produces ever-expanding tilings with 10-fold quasisymmetry. Delve deeper into the history of these tilings, which date back to the Timurid dynasty. Go back all the way to the Topkapi Scroll… then go modern and check out tiling patterns in spherical and hyperbolic geometry. Finally, hear about strings in 4d BF theory, and spin foam models based on the representation theory of 2-groups.

$MathML-enabled post (click for more details).$

It’s worth seeing this nice and big:

I’ve given up asking questions here because people don’t answer them very often. But here’s one. In this picture of the dark stuff on Iapetus, is the dark stuff really all on hilltops? And if so, why?

Are the hills made of dark stuff, with the ice only melting to reveal it near the top? Or did dark stuff land only on hilltops for some reason? The second alternative seems implausible… but both alternatives seem to go against this information from the Wikipedia article:

There is dark material filling in low-lying regions, and light material on the pole-facing slopes of craters, but no shades of grey. The material is a very thin layer, only a few tens of centimeters (approx. one foot) thick at least in some areas, according to Cassini radar imaging and the fact that very small meteor impacts have punched through to the ice underneath.

Posted at October 20, 2009 5:57 PM UTC

TrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/2090

52 Comments & 0 Trackbacks

Re: This Week’s Finds in Mathematical Physics (Week 281)

Maybe the picture is an optical illusion, and the parts that appear high up are really the parts that are low down?

Posted by: Toby Bartels on October 20, 2009 8:17 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

That would be a great explanation… but I just can’t shake this ‘illusion’, even by looking at the picture upside-down.

Oh wait, suddenly I got it to flip!

Posted by: John Baez on October 20, 2009 8:31 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Is she spinning clockwise or counter clockwise?

Posted by: Daniel de França MTd2 on October 20, 2009 8:39 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Try turning the picture upside-down.

No, seriously! I haven’t misunderstood you. The problem is that humans tend to read photographs on the assumption that they are lit from above (ie. from the top of your screen). But the is being lit from below. As a result, the shape-from-shading algorithm in our heads inverts the depth.

Posted by: Dan Piponi on October 20, 2009 10:53 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

I knew about this effect, so after reading Toby and Daniel’s comments I looked at the picture while turning my head upside down, but that didn’t work. What worked was looking at it while ‘knowing’ that they were depressions rather than hills.

Does anyone else see them as hills, or is it just me?

Now I can see them either way.

Posted by: John Baez on October 20, 2009 11:35 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

I saw them as hills, and still saw them as hills after turning my computer upside down, and even after turning my computer upside down and telling myself all the bumps were depressions. Eventually I got it to flip by staring very hard at one particular crater, with my computer upside down, for a while, until that changed—and then when I looked at the other craters, they’d flipped too.

Posted by: Tim Silverman on October 21, 2009 12:01 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

Okay, good — so it’s not just some personal cognitive disorder of mine.

Posted by: John Baez on October 21, 2009 12:04 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

What helped me was imagining that the light source is coming from the bottom of the image. Most of the light sources we see in everyday life come from above, so it’s natural to instead see the craters as hills.

Posted by: Owen Biesel on October 21, 2009 4:26 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Well, if you follow the rim of each “hill”, starting from “south” shadow (south here being the down part of the photo), you will see that is not a hill, but a crater… And the black material is indeed deep in crater. I’m sorry if this is not an answer to your question…

Posted by: Daniel de França MTd2 on October 20, 2009 8:25 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

I imagine the black stuff absorbs more solar energy, and causes more sublimation of the underlying ice, hence the appearance of black exclusively in depressions.

Posted by: Scott Carnahan on October 20, 2009 8:53 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Yes, the Wikipedia article hints at this — but now that I’m seeing the picture correctly I actually believe it!

(Strangely, I can’t even see the picture the wrong way anymore. It’s just obvious that the dark stuff is in craters.)

Here’s what the all-knowing Wikipedia says:

Because of its slow rotation of 79 days (equal to its revolution and the longest in the Saturnian system), Iapetus likely had the warmest daytime surface temperature and coldest nighttime temperature in the Saturnian system even before the development of the color contrast; near the equator, heat absorption by the dark material results in a daytime temperatures of 128 K in the dark Cassini Regio compared to 113 K in the bright regions. The difference in temperature means that ice preferentially sublimates from Cassini, and precipitates in the bright areas and especially at the even colder poles. Over geologic time scales, this would further darken Cassini Regio and brighten the rest of Iapetus, with all exposed ice being lost from Cassini, creating a thermal positive feedback for ever greater contrast in albedo. It is estimated that, at current temperatures, over one billion years Cassini would lose about 20 meters of ice to sublimation, while the bright regions would lose only 10 centimeters, not considering the ice transferred from the dark regions. This model explains the distribution of light and dark areas, the absence of shades of grey, and the thinness of the dark material covering Cassini.

However, a separate process of color segregation would be required to get the thermal feedback started. The initial dark material is thought to have been debris blasted by meteors off small outer moons in retrograde orbits and swept up by the leading hemisphere of Iapetus. The core of this model is some 30 years old, and has been revived by the September flyby.

Posted by: John Baez on October 20, 2009 9:54 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

You have some broken links (due to a missing quotation mark), which should be

Check out the nice photo gallery and the lesson on 5-fold symmetry!

There's also a missing quotation mark in the link to arXiv:0908.0953, this time on the other side.

Posted by: Toby Bartels on October 20, 2009 10:50 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Thanks. Fixed!

Posted by: John Baez on October 21, 2009 12:04 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

A couple of typos:
But when you remove [from] a surface from spacetime
Something interesting is going [on] here.

Posted by: Stuart on October 21, 2009 7:30 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

Thanks, Stuart! Fixed!

Posted by: John Baez on October 22, 2009 6:11 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

This is a 2-group I invented…

And there I was thinking you’d emerged from the cave and glimpsed a Form.

Did Mathieu invent the group $M_{24}$ , or Fischer the group $Fi_{24}$ ?

Posted by: David Corfield on October 21, 2009 8:38 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Yes,

Posted by: Eugene Lerman on October 21, 2009 3:28 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

That comma makes me very concerned, Eugene. Did a ninja sneak up and garrote you while you were posting this comment?

Posted by: John Baez on October 22, 2009 6:50 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

yes….

(more like my eyesight failing me in my late middle age)

Posted by: Eugene Lerman on October 22, 2009 6:55 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

David wrote:

And there I was thinking you’d emerged from the cave and glimpsed a Form.

I knew someone would feel funny about me saying I invented this 2-group, instead of discovering it. Even I felt funny about it.

But in this particular case it felt like ‘invention’. I knew that given a Lie group and a representation of it on a vector space, you get a Lie 2-group. I wanted to give a bunch of examples. So, why not try the Lorentz group and its representation on $\mathbb{R}^4$ ?

This gives some sort of 2-group analogue of the Poincaré group, which is built from the same data. So I called it the ‘Poincaré 2-group’ and hoped someone would figure out something to do with it. Amazingly, someone did.

So: this 2-group wasn’t there waiting for me at the end of a long exploration. I didn’t feel that ‘aha!’ sensation upon meeting it. I didn’t and still don’t have any sense of its conceptual significance. And at a certain level, I’m still mystified by why a spin foam model based on this 2-group should describe a quantum version of Minkowski spacetime.

If the words ‘invention’ and ‘discovery’ are too heavy and fraught with significance, you could say I’m talking about the difference between ‘bumping into’ something and ‘cooking it up’.

By the way, I also hopes someone dreams up a use for the ‘Heisenberg 2-group’. Any central extension of groups gives a 2-group. So, take a symplectic vector space and its usual central extension — the one that gives the Heisenberg group. You get a 2-group. What’s it good for?

Posted by: John Baez on October 22, 2009 7:16 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

I wonder if we should have made more of the Schreiber-Forrester-Barker 2-group. Or even ones I might put my name to such as here or here.

Posted by: David Corfield on October 22, 2009 7:35 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

It’s considered hopelessly declassé to name a theorem or mathematical object after yourself. So, what mathematicians do is name things after their friends, and get their friends to name things after them. It helps if you conveniently forget to make up names for things you discover — or make up really stupid names that nobody wants to use.

If you pick a 2-group you invented (or discovered), I’ll officially name it after you.

Posted by: John Baez on October 22, 2009 7:47 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

this 2-group wasn’t there waiting for me at the end of a long exploration. I didn’t feel that ‘aha!’ sensation upon meeting it. I didn’t and still don’t have any sense of its conceptual significance.

We may have talked about the following before, I forget:

one guess would be that the first-order version of the Einstein-Hilbert action

$\epsilon_{a b c d} \int_X R^{a b} \wedge e^c \wedge e^d$

secretly wants to be read as a BF-type action with

$F^{a b} = R^{a b}$

and

$B^{c d} = e^c \wedge e^d$

This could be achieved by using a slight variant of your Poincaré 2-group, with not $\mathbb{R}^4$ in degree 2, but $\mathbb{R}^4 \wedge \mathbb{R}^4$ .

Here I am using that the BF-action in 4d is really the action for a Lie-2-algebra valued connection, with the $B$ -piece in the shifted component.

Posted by: Urs Schreiber on October 26, 2009 12:12 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

I feel like I should rephrase my previous comment in order to better make its point:

of course there is a vast number of articles that investigates the idea that Einstein gravity is a BF-theory the way I indicated.

What none of these articles seems to do say is that naturally we are to think of BF-theory as a nonabelian 2-gauge theory with the $B$ -field being the degree 2 component.

If one takes any of the models discussed in the literature and interprets them in this sense, one is naturally led to the variation of your (John’s) Poincaré 2-group that I just mentioned.

But then another aspect is remarkable: each and every from this large number of articles on BF 2-form gravity tries to fiddle with the action such as to impose constrains that ensure that on-shell we have

$B^{a b} = e^a \wedge e^b \,.$

None seems to try to investigate the idea that maybe an unconstrained $B^{a b}$ may be fundamentally the true degree of freedom (with all the speculation going on in this area, this is kind of remarkable).

There should be a sensible way to regard this $B \in \Omega^2(X, \wedge^2 \mathbb{R}^4)$ as a vielbein for an area metric.

$G : \wedge^2_{C^\infty(X)} T X \to \wedge^2_{C^\infty(X)} T^* X$

given by

$(v,w) \mapsto B(v,w)^{a b} B_{a b} \,.$

Posted by: Urs Schreiber on October 26, 2009 1:37 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Have you seen this?

Posted by: Daniel de França MTd2 on October 26, 2009 4:49 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

A paper on 2-groups – The 2-group of linear auto-equivalences of an abelian category and its Lie 2-algebra – which also discusses Lie 2-algebras more general than yours:

It is easy to see that the Baez-Crans Lie 2-algebras, from our point of view, are just those pseudo Lie 2-algebras such that the constraint $s$ is the identity. (p. 44)

Posted by: David Corfield on October 30, 2009 4:18 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

David wrote that Xinwen Zhu wrote:

It is easy to see that the Baez-Crans Lie 2-algebras, from our point of view, are just those pseudo Lie 2-algebras such that the constraint s is the identity. [p. 44]

It’s worth noting that Zhu uses the phrase ‘Baez-Crans Lie 2-algebra’ in a different sense than Urs uses ‘Baez-Crans Lie $n$ -algebra’. Urs uses ‘Baez-Crans Lie $n$ -algebra’ to mean a semistrict Lie $n$ -algebra built from a Lie algebra and an $(n+1)$ -cocycle. Zhu is using ‘Baez-Crans Lie 2-algebra’ to mean what we call a ‘semistrict Lie 2-algebra’ — one in which the antisymmetry

$[x,y] = -[y,x]$

still holds on these nose. Zhu is considering more general Lie 2-algebras where there’s an isomorphism:

$s_{x,y} : [x,y] \to [y,x]$

Such more general Lie 2-algebras were already studied by Dmitry Roytenberg. Unfortunately, Zhu does not cite Roytenberg’s work! So, I’ll bring it to his attention.

Also, it would be nice to carefully compare Zhu’s axioms to those of Roytenberg, to see if they’ve converged to the same definition.

Posted by: John Baez on October 30, 2009 6:11 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

I’d say they are hills.

If you examine the central hill on the picture you can see that the shadow below it is cast on the surface which looks exactly like the rest of flat surface - it’s not on a slope. Only hills can cast a shadow on the surrounding flat surface.

Posted by: Arrow on October 21, 2009 3:37 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

Only hills can cast a shadow on the surrounding flat surface.

Good point. But I don’t see such shadows — certainly not in an unambiguous way. So I’m now convinced these are valleys… in part because that just makes more sense.

Maybe we should ask NASA.

Posted by: John Baez on October 22, 2009 7:42 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

here is what nasa says:

http://saturn.jpl.nasa.gov/photos/imagedetails/index.cfm?imageId=2761

Posted by: --- on October 22, 2009 10:39 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

http://saturn.jpl.nasa.gov/photos/imagedetails/index.cfm?imageId=2761

Now I can see them as craters!

Posted by: Toby Bartels on October 22, 2009 10:50 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Just because NASA said they were?

Anyway, thanks to the anonymous commenter above, we now know: “The dark material is preferentially found at the bottoms of craters. Bright water ice forms the ‘bed rock’ on Iapetus, while the dark, presumably loose material apparently lies on top of the ice.”

So now we can wonder: did the dark stuff form the craters by impact, by heating-induced sublimation, or did it just blow around or something and collect in craters. Or all three?

I guess there’s not enough atmosphere on Iapetus for significant wind. If we can’t think of any other process that would collect the dark stuff into patches — except for gravity-induced sliding to the bottoms of craters — then maybe it had to land in blobs in the first place. Which seems a bit weird, given that lots of this stuff is micrometer-to-centimeter sized particles.

Posted by: John Baez on October 22, 2009 11:07 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Just because NASA said they were?

No; not only was I already convinced that they were craters based on previous comments, I still can only see the features in your picture as hills. (Even when I turn your picture upside down. Even though that makes it extremely similar to NASA's picture, with large areas of overlap.)

Posted by: Toby Bartels on October 23, 2009 12:22 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

I also immediately see the NASA picture as craters, while the one posted earlier really “wants” to be hills. Weird.

Posted by: Tim Silverman on October 23, 2009 9:20 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Just to be irritatingly pedantic: both pictures are from NASA. Nobody else has ever taken closeup photos of Iapetus.

But anyway, I decided to replace the picture on week281 by a picture that doesn’t cause this particular illusion quite so much… although I can still get it to happen if I want!

The illusion is fun, so I’ll leave it here, but it doesn’t help people understand what’s happening on Iapetus.

Posted by: John Baez on October 23, 2009 7:31 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Just to be irritatingly pedantic: both pictures are from NASA.

I was identifying them by website, not photographer. (–_^)

Posted by: Toby Bartels on October 24, 2009 12:48 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

Brian Wichmann pointed out this online database of his:

A tiling database.

Here’s a sample database entry, based on a tiling in the Alhambra:

Posted by: John Baez on October 22, 2009 6:09 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Should the possible union of string and loops be called “STROOP” theory?

Posted by: Doug on October 22, 2009 5:27 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

It will be called string theory.

Posted by: John Baez on October 22, 2009 6:02 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Robert Schlesinger sent me an email saying:

Regarding Islamic patterns and tilings, there is also a vast literature in Russian and needless to say, Arabic and Farsi. I received, e.g., a large package of reprints on the subject about 25 years ago from a mathematician in the East Germany, and many of the papers were in Russian. Branko Gruenbaum’s book is a beauty and encyclopedic. Many tilings and patterns are arranged to make Kufic inscriptions or Surahs, and are often ingenious. Stylized Islamic calligraphy is also a vast field unto itself and the art is amazing and also with many interesting symmetries. The Arabic alphabet seems especially adapted to generating interesting symmetries. This relates to the cursive nature of the script. Even a linguistics novice such as myself discovered a few calligraphic symmetries using the Arabic calligraphy for “akbar” (literally, “great”). Islamic tilings and patterns appear in many places throughout the Islamic cultures, such as wooden furniture, screens (e.g., joli screens), chess sets, picture frames, even tea sets and plates. I have some beautiful joli screens in my collection. A favorite Islamic plate (not in my collection) is from 10th century Samarkand, and has a Kufic inscription about its rim, which states, as I recall: “The pursuit of knowledge is bitter to the taste at first, but soon is sweeter than honey. Safety. ” [The word safety is used as a filler.] If interested, I’ll make a pdf of the plate photo and send it along.

Posted by: John Baez on October 22, 2009 9:41 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

joli screens

Google suggests that we spell this

jali screens

and then gives lots of hits with good pictures.

Posted by: Toby Bartels on October 22, 2009 10:23 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

From the late-16th-century Mughal Dynasty in India — irregular pentagons, but stars nice enough to please the 5-lovers among us:

Posted by: John Baez on October 22, 2009 11:16 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

Regarind the above joli scrren, here’s a somewhat astounding email that Jim Stasheff and I got from Satyan Devadoss, who let me repost it here:

Hi Jim,

with regards to John’s posting: there is more than art here. If we just consider the pentagons in this picture (and pretend the octagons are punctures in the plane, with antipodal points glued), we end up with a generalization of the real compactified moduli space $M^{0,n}(R)$ extended to the affine Coxeter complex of type $\tilde{C}_2$ .

It has the Dynkin diagram

o-(4)-o-(4)-o ,

a path with 3 nodes, with 2 edges labeled “4”.

It is tiled by associahedra (as seen by the pentagons).

For more info on this, see the paper “Particle configurations and Coxeter operads” by Armstrong et al in Journal of Homotopy and Related Structures. In particular, see Figure 2(c) which shows this tiling (before blowing up certain points which give us the octagons)… and Theorem 6.4 showing it to be tiled by associahedra.

Satyan

Posted by: John Baez on October 28, 2009 4:28 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

Michael D. Hirschhorn emailed me to say that nearly 30 years ago, he and David C. Hunt published a paper in the Journal of Combinatorial Theory classifying all tilings of the plane by identical convex equilateral pentagons. The most famous appears to be the ‘Hirschhorn medallion’. Bob Jenkins used it to tile his bathroom:

Later Hirschhorn and Hunt extended their result to cover all non-convex equilateral tilings, but this has never been published.

Posted by: John Baez on October 23, 2009 2:45 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

Dear John,

David and I did not claim to find all the tilings of equilateral pentagons. We found all the equilateral pentagons that tile, but not necessarily all the possible tilings.

For example the pentagon with angles 100,140,60,160,80 degrees (the `versatile’) tiles in many ways, including both regular and spiral tilings, but we did not attempt to find all its tilings.

Best wishes,

Mike.

Posted by: michael d. hirschhorn on November 1, 2013 5:19 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

I just got an email from Craig Kaplan, whose wonderful work on tilings I mentioned. He found it particularly pleasing to be mentioned in the same breath with Greg Egan, who is one of his favorite authors. And he writes:

Because of the content of your post, I can’t help but offer a few notes about what you said. Feel free to use these any way you want, or file them away for later.

I wouldn’t say that the Timurids set out to tackle fivefold tilings. They looked at a lot of geometry in general – it’s not clear to me that they devoted any more energy to 5 than any other number. But they did produce amazing results!

You should be aware that within the Islamic geometric art community, there’s a fair amount of controversy and resentment surrounding the Lu and Steinhardt paper. First, the paper contains very strong claims that aren’t supported by evidence. Even if the artisans had some understanding of inflation (which is debatable), I don’t think there’s any way they would have had a notion of quasiperiodicity. Second, several researchers perceive that L&S muscled their way into unfamiliar territory without really finding out what had been done before – one could argue that most of the work in their paper was well known to the community. Finally, the paper made its mark not because of the originality of its contribution, but because Science rolled out an enormous publicity machine around the paper’s release. This is something that academics can’t really control for, and which I still find a bit baffling.

Man, I’d also love to get my hands on Necipoglu’s book on The Topkapi Scroll. I knew of the book when it was in print, and didn’t buy it.

Cromwell’s article was in part a response to Lu & Steinhardt’s. You also might be interested in three upcoming articles of his, to appear in the Journal of Mathematics and the Arts (for which I’m an AE):

Islamic geometric designs from the Topkapi Scroll I: Unusual arrangements of stars

Islamic geometric designs from the Topkapi Scroll II A modular design system

Hybrid 1-point and 2-point constructions for some Islamic geometric designs

Hopefully they’ll be out soon.

In the meantime, I might also add that I did a bit of work on understanding the origin of strange tilings like the one you show with decagons, pentagons, and funky hexagons. It’s in this paper, which you didn’t link to:
http://www.cgl.uwaterloo.ca/~csk/papers/gi2005.html

Hope that’s useful to you, and thanks for the mention.

Posted by: John Baez on October 23, 2009 3:13 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

It’s not exactly the same kind of tiling, but here’s a nice Escheresque Fractal sort of pattern I encountered in connection with Higher Order Propositions:

HOP to Table 8

Posted by: Jon Awbrey on October 23, 2009 1:20 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

I wrote a new version of the Girih applet, which scrolls across an infinite quasiperiodic tiling at a single scale. (I start with a Penrose rhombic tiling that I construct by de Bruijn’s method, and then convert into a tiling of decagons, hexagons and bowties.)

This one can be run in full-screen mode.

Posted by: Greg Egan on October 23, 2009 3:13 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

Nice!

Posted by: John Baez on October 23, 2009 7:56 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

How are quantum gravity people taking these results – A limit on the variation of the speed of light arising from quantum gravity effects? Had there been predictions for departures from Lorentz invariance?

Posted by: David Corfield on October 30, 2009 10:19 AM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

There had been papers suggesting violations or modifications of Lorentz-invariance due to quantum gravity effects. None of these papers could ‘derive’ these effects from some well-worked out theory of quantum gravity — except in 3d spacetime, where there’s a beautiful mechanism that leads to the Poincaré group being $q$ -deformed. But physicists are hungry for predictions that can be falsified, even if they don’t emerge from any deep theory! Here’s a sampling of papers:

Joao Magueijo and Lee Smolin, Generalized Lorentz invariance with an invariant energy scale.
Lee Smolin, Falsifiable predictions from semiclassical quantum gravity.
Giovanni Amelino-Camelia and Lee Smolin, Prospects for constraining quantum gravity dispersion with near term observations.

The abstract of the last one, from 2009, conveys the mood of this line of work:

Abstract: We discuss the prospects for bounding and perhaps even measuring quantum gravity effects on the dispersion of light using the highest energy photons produced in gamma ray bursts measured by the Fermi telescope. These prospects are brigher than might have been expected as in the first 10 months of operation Fermi has reported so far eight events with photons over 100 MeV seen by its Large Area Telescope (LAT). We review features of these events which may bear on Planck scale phenomenology and we discuss the possible implications for the alternative scenarios for in-vacua dispersion coming from breaking or deforming of Poincare invariance. Among these are semi-conservative bounds, which rely on some relatively weak assumptions about the sources, on subluminal and superluminal in-vacuo dispersion. We also propose that it may be possible to look for the arrival of still higher energy photons and neutrinos from GRB’s with energies in the range $10^14$ - $10^17$ eV. In some cases the quantum gravity dispersion effect would predict these arrivals to be delayed or advanced by days to months from the GRB, giving a clean separation of astrophysical source and spacetime propagation effects.

Of course, the danger of making falsifiable predictions is that they can be falsified.

Posted by: John Baez on October 30, 2009 5:50 PM | Permalink | Reply to this

Re: This Week’s Finds in Mathematical Physics (Week 281)

$MathML-enabled post (click for more details).$

But physicists are hungry for predictions that can be falsified, even if they don’t emerge from any deep theory!

Indeed, we see the loud criticism of not-yet-testable theories has driven people to the opposite problematic extreme: not-yet-theoretic predictions.

In some circles it is getting to the point that if only you can argue that it could be measured by the next NASA sattelite, then it doesn’t matter where you got your idea from.

But since all observation needs to be interpreted in some framework, quasi-theory-less predictions are just a bad as no predictions. Even if the predicted effect is measured tomorrow, all we are left with is nagging doubts if our would-be theory now really predicted this or if the effect is actually explained by a totally different theory that we hadn’t had time to develop, due to being occupied with making unfounded predictions.

I may be wrong, but my impression is that in the 80s or so there was a healthy activity by people who did care about the precise clean formal structural aspects of the hypothetical theories of nature under discussion since then. Probably largely because progress in that realm is always very slow, these people seem to have entually died out, or quit. In theoretical physics departments, who is thinking concentrated about structural questions these days? Almost nobody. Many are too busy making predictions out of thin air.

Posted by: Urs Schreiber on October 30, 2009 10:47 PM | Permalink | Reply to this

The n-Category Café

Skip to the Main Content

October 20, 2009