November 29, 2022
Last Seminar on This Week’s Finds
Posted by John Baez
On Thursday December 2nd I gave the last of this year’s seminars on This Week’s Finds. You can see videos of all ten here. I will continue in September 2023.
In my last one, I spoke about Dyson’s ‘three-fold way’: the way the real numbers, complex numbers and quaternions interact in representation theory and quantum mechanics. For details, try my paper Division algebras and quantum theory.
One cute fact is how an electron is like a quaternion! More precisely: how quaternions show up in the spin-1/2 representation of SU(2) on ℂ².
Let me say a little about that here.
November 27, 2022
Mathematics for Humanity: a Plan
Posted by John Baez
I’m working with an organization that may eventually fund proposals to fund workshops for research groups working on “mathematics for humanity”. This would include math related to climate change, health, democracy, economics, etc.
I can’t give details unless and until it solidifies.
However, it would help me to know a bunch of possible good proposals. Can you help me imagine some?
A good proposal needs:
a clearly well-defined subject where mathematics is already helping humanity but could help more, together with
a specific group of people who already have a track record of doing good work on this subject, and
some evidence that having a workshop, maybe as long as 3 months, bringing together this group and other people, would help them do good things.
I’m saying this because I don’t want vague ideas like “oh it would be cool if a bunch of category theorists could figure out how to make social media better”.
November 22, 2022
Inner Automorphisms of the Octonions
Posted by John Baez
What are the inner automorphisms of the octonions?
November 16, 2022
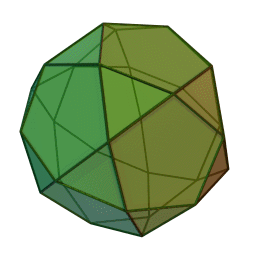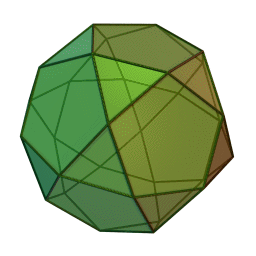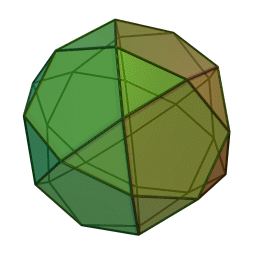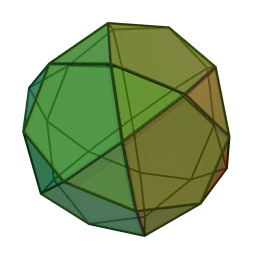
The Icosidodecahedron
Posted by John Baez
The icosidodecahedron can be built by truncating either a regular icosahedron or a regular dodecahedron. It has 30 vertices, one at the center of each edge of the icosahedron—or equivalently, one at the center of each edge of a dodecahedron. It is a beautiful, highly symmetrical shape. But it is just a shadow of a more symmetrical shape with twice as many vertices, which lives in a space with twice as many dimensions! Namely, it is a projection down to 3d space of a 6-dimensional polytope with 60 vertices.
Even better, it is also a slice of a more symmetrical 4d polytope with 120 vertices, which in turn is the projection down to 4d space of an even more symmetrical 8-dimensional polytope with 240 vertices: the so-called ‘E8 root polytope’.
Note how the numbers keep doubling: 30, 60, 120 and 240.
November 1, 2022
Categories and Epidemiology
Posted by John Baez
I gave a talk about my work using category theory to help design software for epidemic modeling:
• Category theory and epidemiology, African Mathematics Seminar, Wednesday November 2, 2022, 3 pm Nairobi time or noon UTC. Organized by Layla Sorkatti and Jared Ongaro.
This talk is a lot less technical than previous ones I’ve given on this subject, which were aimed mainly at category theorists. You can watch it on YouTube.
 Posts with this logo use
Posts with this logo use