December 29, 2009
A Question About the Job Application Process
Posted by Alexander Hoffnung
Since I am currently applying for jobs it probably would have been a good idea for me to keep something here like a journal collecting and possibly offering advice about the application process. Alas, I have not done that, but I am also still submitting applications so maybe there is hope yet.
Let me start by explaining the problem I encountered today and see if anyone else has had this problem or can offer some explanation. Then maybe I can just open up the floor for people to say what problems they are running into or to give advice that they have gathered and want to share.
If there is enough feedback then it would probably be useful to create an nLab page to collect job advice over time.
December 21, 2009
Cobordism and Topological Field Theories Weeks 6 & 7
Posted by Alexander Hoffnung
Welcome back to the cobordism and TFT seminar at UCR! We are posting weeks 6 and 7 of the seminar simultaneously because it flows nicely and the new quarter will start before we know it. So we want to be all caught up by the first week of the second quarter.
Week 6 starts back with the regular lecture series by Julie Bergner. These lectures are especially nice as they begin to introduce -dimensional topological field theories in Lecture 6 and the higher category theory needed to formulate these concepts in Lecture 7.
You can click here for the previous week’s lecture and keep reading below the fold for a link to the notes for Weeks 6 and 7…
December 20, 2009
This Week’s Finds in Mathematical Physics (Week 287)
Posted by John Baez
In week287 of This Week’s Finds, discover Jean-Pierre Marquis and Gonzalo Reyes’ history of categorical logic. Then continue learning about rational homotopy theory! Learn a quick way to construct differential forms starting from any commutative algebra… and see how Sullivan used this to construct “rational differential forms” starting from any topological space.
December 18, 2009
Galois Theory in Two Variables
Posted by David Corfield
I have just returned from attending three days of the Final Workshop of the Newton Institute Non-Abelian Fundamental Groups in Arithmetic Geometry Programme. I was kindly invited by Café visitor Minhyong Kim, whose lecture we discussed a while ago.
While many of talks were well beyond me, I could detect a few glimmers through the mist, and this was greatly helped by some lengthy chats with Minhyong. Out of these discussions I could also see emerge the seeds of a number of philosophy papers – but more of that another time.
After a tricky start, Mihnyong’s own talk I found one of the most approachable. It rises to a crescendo with the idea that we need a Galois theory for polynomials in two variables. Now wouldn’t that make for a wonderful Polymath project for our culture? All kinds of things we hold dear to us at the Café would be involved – nonabelian duality, nonabelian cohomology, the symmetries of pairs of rational solutions in a configuration space linked by a path… And there’s even the hope for higher-dimensional algebraic entities to play a role, as suggested back here.
Could we entice Minhyong to lead an online project?
December 13, 2009
Can Five-Year-Olds Compute Coproducts?
Posted by John Baez
Charlie Clingen points out the following article:
- Steven Phillips, William H. Wilson and Graeme S. Halford, What do transitive inference and class inclusion have in common? Categorical (co)products and cognitive development, PLoS Computational Biology.
December 12, 2009
The Problem With Lax Functors
Posted by Mike Shulman
As many readers probably know, part of categorification involves replacing equalities by coherent isomorphisms or equivalences. This is basically unavoidable, since after replacing sets by categories, they’re now objects of a category, so it would be evil to ask them to be equal instead of isomorphic. However, there’s another side to categorification: once we turn equalities into isomorphisms, we can go one step further and allow them to be arbitrary morphisms, not even invertible.
Over at the nLab we’ve been discussing how to distinguish between these two terminologically. Whatever we call them, they’re both indisputably important. But the second one (“directification” or “laxification”) has some subtleties that can trap the unwary. In particular, the notion of lax functor between 2-categories (or -categories for ) is surprisingly ill-behaved. You can’t make a tricategory out of 2-categories, lax functors, and any of the usual sorts of transformations. In fact, lax functors are not even invariant under equivalence!
(Thanks to Chris Schommer-Pries for bringing up this question at mathoverflow and on SBS. I thought I’d take the liberty of elaborating here, since it gives me the opportunity to proselytize a bit more on one of my favorite topics.)
December 9, 2009
This Week’s Finds in Mathematical Physics (Week 286)
Posted by John Baez
In week286 of This Week’s Finds, begin learning about rational homotopy theory! See how to build a space whose fundamental group is the rational numbers:

Learn how to ‘rationalize’ a space. And see three descriptions of the rational homotopy category.
December 8, 2009
Syntax, Semantics, and Structuralism II
Posted by Mike Shulman
Recently several of us have been making a lot of noise about “structural set theory,” also known as “categorical” or “categorial” set theory. This phrase refers to a general class of theory akin to Lawvere’s ETCS, which describes sets from a purely “structural” point of view. A brief way to say this is that we care only about the category of sets, in which isomorphic sets are indistinguishable, rather than the class of sets equipped with a global membership relation . In structural set theory, which claims to be closer to the way sets are actually used in most mathematics, it doesn’t make sense to ask whether (for instance) , since and are elements of the set but not sets themselves. Rather than coming with an intricate set-membership structure, sets in structural set theory are simply the “raw material” with which we build mathematical structures such as groups, rings, spaces, manifolds—and even “set-membership structures”!
This is sort of a continuation of this post, but it should also stand mostly alone. I’ll also summarize some of the things I learned from this previous discussion.
When Naturality Fails
Posted by David Corfield
I started a page at Lab on the notion of injective hulls. It seems to cover a range of interesting constructions, including algebraic field extensions and Dedekind-MacNeille completion. But it appears that it is not ‘natural’. So I asked at the Forum
Does this tell us something interesting?
Toby replied
This has always been a strange thing to beginning category theorists (and maybe still for the rest of us). The usual example seems to be the algebraic closure of a field (although that’s an injective hull only in a slightly unobvious category, now noted on our page). Any two algebraic closures are isomorphic, but not canonically so, and this applies to injective hulls in general.
It should tell us something, but I don’t know what.
Can anyone help us?
December 7, 2009
Cobordism and Topological Field Theories Week 5
Posted by Alexander Hoffnung
Welcome to week 5 of the cobordism and TFT seminar at UCR! Time has apparently sped up a great deal as week 4 was posted only a few days ago. Since the quarter is coming to an end here at Riverside the lecture notes should start appearing rather quickly now. This week is special since you will be getting nicely polished lecture notes. John Huerta has prepared a detailed story based on his lecture “A Short History of the Interaction Between QFT and Topology”.
You can click here for the previous week’s lecture and keep reading below the fold for the abstract and link to this week’s lecture notes…
December 6, 2009
This Week’s Finds in Mathematical Physics (Week 285)
Posted by John Baez
In week285 of This Week’s Finds, discover the beauty of roots:
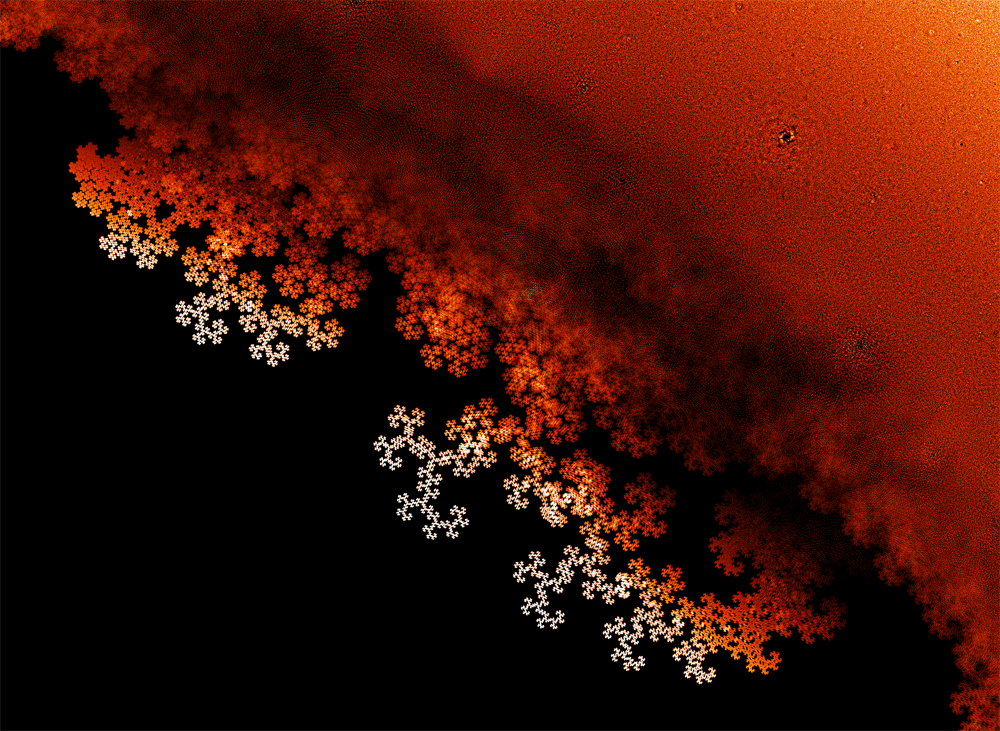
Then hear about the second day’s talks on homotopy theory and higher algebraic structures at the AMS conference at U.C. Riverside. Learn about string topology, string connections, 2-gerbes, orbifolds, twisted K-theory, monoids of moduli spaces of manifolds, categorified quandles, long knots and Vassiliev theory, 3-categories for the working mathematician… and the blob complex!
Cobordism and Topological Field Theories Week 4
Posted by Alexander Hoffnung
Welcome to week 4 of the cobordism and TFT seminar at UCR by Chris Carlson. The previous lecture was put up a few weeks ago here. Sorry for the long break, but the next few should be coming in a more timely manner. So keep an eye out. This week’s lecture introduces -dimensional topological quantum field theories and commutative Frobenius algebras.
December 2, 2009
Size, Yoneda, and Limits of Algebras
Posted by Mike Shulman
Let be a monad on a category , and its category of Eilenberg-Moore algebras with forgetful functor . Consider the following two (true) statements.
- For all and , if is any diagram such that has a limit in , then has a limit in which is preserved by .
- For all and , if is complete, then so is , and is continuous.
Obviously the first implies the second. Interestingly, the second also implies the first, by a clever Yoneda-lemma argument. However, the argument applies a priori only to small categories, so it provides one convenient testing-ground to compare different ways of dealing with size.
December 1, 2009
Dangerous Knowledge
Posted by John Baez
It’s been around a while, so maybe you’ve already seen it… but I just heard about the BBC documentary called Dangerous Knowledge. According to the summary, it’s about “four brilliant mathematicians — Georg Cantor, Ludwig Boltzmann, Kurt Gödel and Alan Turing — whose genius has profoundly affected us, but which tragically drove them insane and eventually led to them all committing suicide”. And now you can see it on YouTube.
It sounds fun, or at least fun to complain about. Did Cantor really commit suicide? I thought he died of a heart attack. Was Ludwig Boltzmann a mathematician? I thought he was a physicist. And did Alan Turing commit suicide because his genius drove him insane? I thought it had something to do with the British government convicting him for homosexuality and punishing him by forcing him to take estrogen, which made him grow breasts.
But the documentary is more fun than the summary. I’m watching it right now. It’s charmingly dark and edgy, featuring lines like:
Cantor is wonderful because… it’s so crazy! It’s the equivalent of being on drugs.
What could the greatest mathematician of his century have seen that could have driven him insane?
If you have a friend who’s a goth, and you’re trying to explain to them why you like math, have them watch this.
 Posts with this logo use
Posts with this logo use 














