The n-Category Café

Main results

Our working model for all concrete computations in the following is $2\mathrm{Cat}$, the Gray category whose objects are strict 2-categories, whose morphisms are strict 2-functors, whose 2-morphisms are pseudonatural transformations and whose 3-morphisms are modifications of these. It is clear that all our statements ought to have analogs for weaker, more general and higher $n$ versions of $n$-categories. But with a good general theory of higher $n$-categories still being somewhat elusive, we won’t bother to try to go beyond our model $2\mathrm{Cat}$.

So we shall now set $n = 2$ once and for all and take the liberty of using $n$ instead of 2 in our statements, to make them look more suggestive of the general picture which ought to exist.

Tangent $(n+1)$-bundle

We define for any $n$-category $C$ an $n$-category $TC$ which is an $(n+1)$-bundle $p : TC \to \mathrm{Obj}(C)$ over the space of objects of $C$. This we address as the tangent bundle of $C$.

The definition of this tangent bundle is morally similar to but in detail somewhat different form the way tangent bundles are defined in synthetic differential geometry and in supergeometry:

we consider the category $$\mathbf{pt} := \{ \bullet \stackrel{\sim}{\to} \circ \}$$ as an arrow-theoretic model for the “infinitesimal interval” or the “superpoint” in that it is a puffed-up version of the mere point $$\mathrm{pt} := \{ \bullet \}$$ to which it is equivalent, by way of the injection $$\mathrm{pt} \hookrightarrow \mathbf{pt} \,,$$ but not isomorphic. This suble difference, rooted deeply in the very notion of category theory, we claim usefully models the notion of tangency as “extension which hardly differs from no extension”. Concretely, we consider $$TC \subset \mathrm{Hom}_{n\mathrm{Cat}}(\mathbf{pt}, C)$$ to be that subcategory of morphisms from the fat point into $C$ which collapses to a 0-category after pulled back to the point $\mathrm{pt}$.

The characteristic property of the tangent $(n+1)$-bundle is that it sits inside the short exact sequence $$\mathrm{Mor}(C) \to T C \to C \,.$$

Finally, for later use notice that dual to its realization as a projection $$T C \to \mathrm{Obj}(C)$$ the tangent bundle may be thought of as an $n$-functor $$T C : C^{\mathrm{op}} \to n\mathrm{Cat}$$ which sends objects $a$ to the tangent categories $T_a C$ over them and sends morphisms the the pullback of these along them $$T C \; : \; ( a \stackrel{f}{\leftarrow} b ) \; \mapsto \; ( T_a C \stackrel{T_f C}{\to} T_b C ) \,.$$

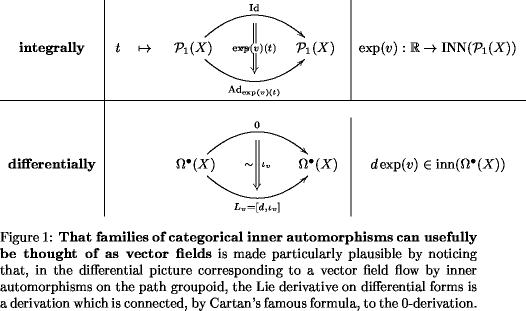
Vector fields and Lie derivatives

Let $X$ be a smooth manifold and let $P_1(X)$ be the groupoid of thin homotopy classes of paths in $X$.

Then ordinary vector fields $v \in \Gamma(TX)$ on $X$ are in canonical bijection with smooth 1-parameter families of categorical tangent vectors to the identity map on $P_1(X)$: $$\Gamma(TX) \; \stackrel{\sim}{\to} \; \{ \Sigma \mathbb{R} \to \Sigma T_{\mathrm{Id}_{\mathcal{P}_1(X)}}(\mathrm{Cat}) \} \,.$$

On a general category $C$, it may be useful to consider generalizations of this where $\mathbb{R}$ is replaced by some other group $G$. We speak of $G$-flow on a category, in this general case.

The “arrow fields” on a category $C$, considered by Isham in [Isham], are $\mathbb{Z}$-flows $$\{ \Sigma \mathbb{Z} \to \Sigma T_{\mathrm{Id}_{C}}(\mathrm{Cat}) \}$$ on $C$.

On the other hand, the identitfication of $T C$ itself (as opposed to $T_{\mathrm{Id}_C}{\mathrm{Cat}}$) with ordinary vectors for suitable choice of $C$ is both more subtle and more interesting than the above. This will be discussed elsewhere, once fully worked out.

Inner automorphism $n$-groups

Of particular importance are the tangent bundles, in our sense, to $n$-categories which are 1-object $(n-1)$-groupoids $\Sigma G_{(n)}$, hence $n$-groups $G_{(n)}$. In our context these $(n-1)$-groupoids must be thought of as 1-point orbifolds. Accordingly, they have just a single “tangent space” (tangent $n$-category) $$T_\bullet \Sigma G_{(n)} \,.$$

This turns out to have interesting properties [Roberts-S]:

$\bullet$

For $G$ an ordinary group, one finds that $$T_\bullet \Sigma G \simeq T_{\mathrm{Id}_{\Sigma G}}(\mathrm{Cat})$$ is a 2-group, which we call $\mathrm{INN}(G)$. It sits inside the exact sequence $$Z(G) \to \mathrm{INN}(G) \to \mathrm{AUT})(G) \to \mathrm{OUT}(G)$$ of 1-groupoids. Here $Z(G)$ is the categorical center of $\Sigma G$ (which coincides with the ordinary center of $G$), regarded as a 1-object groupoid. This identifies $\mathrm{INN}(G)$ as the 2-group of inner automorphisms of $G$. But $\mathrm{INN}(G)$ also sits inside the exact sequence $$\mathrm{Disc}(G) \to \mathrm{INN}(G) \to \Sigma G \,.$$ Moreover, it is equivalent to the trivial 2-group, hence “contractible”. This identifies $\mathrm{INN}(G)$ as the categorical version of the universal $G$-bundle.

$\bullet$

For $G_{(2)}$ a strict 2-group, one finds that $$T_\bullet \Sigma G_{(2)} \subset T_{\mathrm{Id}_{\Sigma G_{(2)}}}(2\mathrm{Cat})$$ is a 3-group, which we call $\mathrm{INN}(G_{(2)})$. It sits inside the exact sequence $$Z(G_{(2)}) \to \mathrm{INN}(G_{(2)}) \to \mathrm{AUT})(G_{(2)}) \to \mathrm{OUT}(G_{(2)})$$ of 2-groupoids. Here $Z(G_{(2)})$ is the 2-categorical center of $\Sigma G$, regarded as a 1-object 2-groupoid. This identifies $\mathrm{INN}(G_{(2)})$ as the 3-group of inner automorphisms of $G$. But $\mathrm{INN}(G_{(2)})$ also sits inside the exact sequence $$\mathrm{Disc}(G_{(2)}) \to \mathrm{INN}(G_{(2)}) \to \Sigma G_{(2)} \,.$$ Moreover, it is equivalent to the trivial 3-group, hence “contractible”. This identifies $\mathrm{INN}(G_{(2)})$ as the categorical version of the universal $G_{(2)}$-2-bundle.

Curvature and Bianchi Identity for functors

General functors

Using the functorial incarnation $TC : C^{\mathrm{op}} \to n\mathrm{Cat}$ of the tangent bundle, we may push forward any $n$-functor $$F : C \to D$$ to a connection on the tangent bundle of $C$, simply by postcomposing $$d F : C \stackrel{F}{\to} D \stackrel{T D}{\to} n\mathrm{Cat} \,.$$ The crucial point of this construction is that it extends uniquely (up to equivalence) to an $(n+1)$-functor $$d F : C_{(n+1)} \to n\mathrm{Cat}$$ on the $(n+1)$-category $$C_{(n+1)} := \mathrm{Codisc}(C)$$ which is obtained from $C$ by replacing all Hom-($n-1$)-categories by the corresponding codiscrete $n$-groupoids over them.

By introducing the terminology

$\bullet$ $d F$ is the curvature of $F$
$\bullet$ $F$ is flat if $d F$ is degenerate (sends all $(n+1)$-morphisms to identities)

we obtain the technically easy but conceptually important generalization of the Bianchi identity: for any functor $F$

$\bullet$ $d F$ is flat

or equivalently

$\bullet$ $d d F$ is degenerate.

Parallel transport functors and differential forms

When $F : C \to D$ is the smooth parallel transport functor \cite{transport} in an $n$-bundle with connection [Bartels,Baez-S,S3], the arrow-theoretic notion of curvature described above does reproduce the theory of curvature forms of connection forms. The general Bianchi identity we have discussed then reduces to the ordinary Bianchi identity familiar from differential geometry.

More precisely, let $C := \mathcal{P}_3(X)$ be the strict 3-groupoid of thin homotopy classes of $k$-paths in a smooth manifold $X$. And let $G_{(2)}$ be a strict Lie 2-group coming from the Lie crossed module $H \stackrel{t}{\to} G \stackrel{\alpha}{\to} \mathrm{Aut}(H)$.

Then, according to [S-Waldorf,Baez-S,S3,Roberts-S] we have the following bijections of smooth $n$-functors with differential forms

$\bullet$ $\left\lbrace \mathrm{smooth}\;1-\mathrm{functors} \mathcal{P}_1(X) \to \Sigma G \right\rbrace \stackrel{\sim}{\to} \left\lbrace A \in \Omega^1(X,\mathrm{Lie}(G)) \right\rbrace$

$\bullet$

$\left\lbrace \mathrm{smooth}\;2-\mathrm{functors} \mathcal{P}_2(X) \to \Sigma G_{(2)} \right\rbrace \stackrel{\sim}{\to} \left\lbrace (A,B) \;\; F_A + t_*\circ B = 0 \right\rbrace$

$\bullet$

$\left\lbrace \mathrm{smooth}\;3-\mathrm{functors} \mathcal{P}_3(X) \to \Sigma \mathrm{INN}(G_{(2))} \right\rbrace \stackrel{\sim}{\to} \left\lbrace (A,B,C) \;\; C = d_A B \right\rbrace$

Now let $$\mathrm{tra} : P_1(X) \to \Sigma G$$ be a smooth 1-functor with values in the Lie group $G$. Then, under these bijections, we find that its curvatures correspond to the following differential forms at top level $$\begin{aligned} \mathrm{tra} &\mapsto A \\ \mathrm{curv} = d \mathrm{tra} &\mapsto F_A := d A + A \wedge A \\ d \mathrm{curv} = d d \mathrm{tra} &\mapsto d_A F_A = 0 \end{aligned} \,.$$

This way the ordinary Bianchi identity for the curvature 2-form $F_A$ of $A$ is reproduced. Notice that for this result come out the way it does, just by turning our abstract crank for differential arrow-theory, the result of \ref{Inner automorphism $n$-groups} is crucial, which says that the curvature $(n+1)$-functor of a $G_{(n)}$-transport is itself an $\mathrm{INN}(G_{(n)})$-transport.

Sections and covariant derivatives

The curvature $\mathrm{curv} = d \mathrm{tra}$ of a parallel transport $n$-functor is typically trivializable, in that it admits morphisms $$e : I \stackrel{\sim}{\to} \mathrm{curv}$$ for $I$ some “trivial” $(n+1)$-transport. As with the inner automorphism $(n+1)$-groups $\mathrm{INN}(G_{(n)})$, this trivializability, far from making these objects uninteresting, turns out to control the entire theory.

(Compare this to the contractibility of the universal $G$-bundle: while equivalent to a point, it is far from being an uninteresting object, due to the morphisms which go into and out of it. According to the above this comparison is far more than an mere analogy.)

A basic fact of $n$-category theory has major implications here:

recall that for $F$ and $G$ $n$-functors, a transformation $$G \to F$$ is given in components itself by an $(n-1)$-functor. Now if $G$ is trivial in some sense to be made precise, and if the transformation is an equivalence $$I \stackrel{f_\sim}{\to} F \,,$$ then this implies that the $n$-functor $F$ is entirely encoded in the $(n-1)$-functor $f$.

We show that for $F = \mathrm{curv} = d\mathrm{tra}$ the curvature $(n+1)$-functor of a transport $n$-functor $\mathrm{tra}$, the latter essentially encodes the component map of the transformation $$I \stackrel{\mathrm{tra}_\sim}{\to} \mathrm{curv} \,.$$ In components this is nothing but a generalization of Stokes’ law $$\int_X d \omega = \int_{\partial X} \omega \,.$$

Moreover, it turns out that there may be other trivializations of $\mathrm{curv}$, not by isomorphisms but by mere equivalences. On objects, the component functions of these correspond to sections of the original bundle. On morphisms it corresponds, under the identification of smooth functors and differential forms mentioned above to the covariant derivative of these sections.

In [S1] it is indicated how all these statements have a quantum analogue as we push our $n$-functors forward. There it is indicated how the fact that transport $n-functors$ have sectins which are themselves transport $(n-1)$-functors translates in the context of extended functorial quantum field theory to essentially what is known in physics as the holographic principle. This needs to be discussed elsewhere, clearly.