The n-Category Café

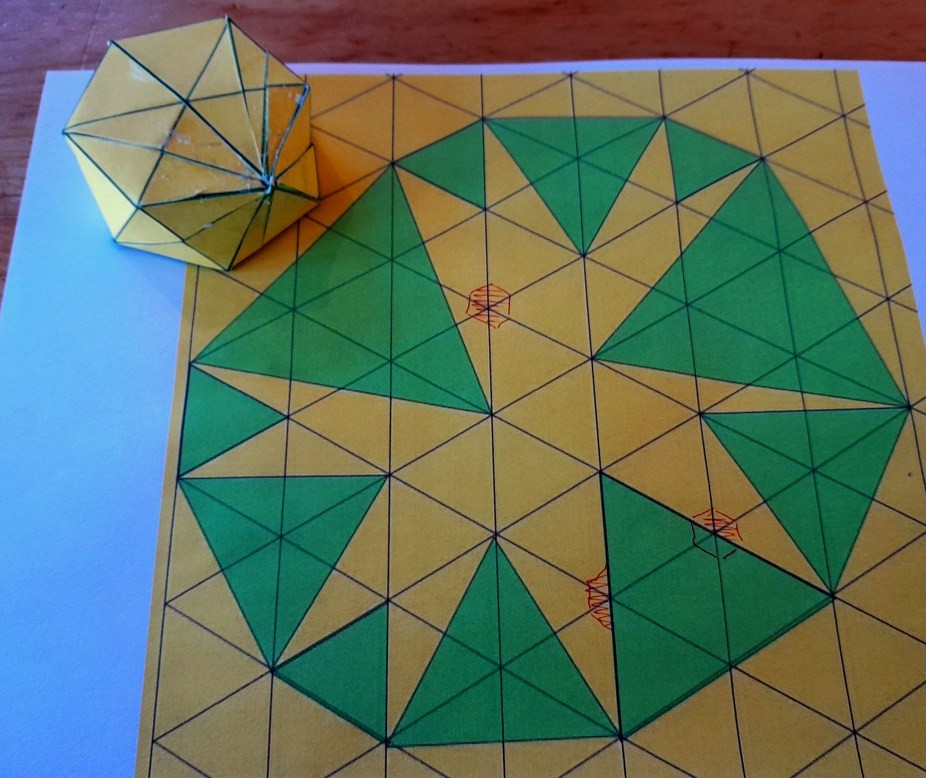
Here is Thurston’s procedure. First draw the lattice of Eisenstein integers in the complex plane:

$$\mathbb{E} = \{ a + b \omega \; \vert \; a, b \in \mathbb{Z} \}$$

where $\omega = \exp(2 \pi i / 3)$. Then draw an 11-sided polygon $P$ whose vertices are Eisenstein integers. Along each edge of $P$, draw an equilateral triangle pointing inward. Make sure to choose the polygon $P$ so that these triangles touch each other only at its corners.

If you remove these triangles, you are left with an 11-pointed star. You can always fold up this star so all its tips meet at one point, creating a convex polyhedron. This in turn gives a triangulation of the 2-sphere, where the triangles are those coming from the lattice of Eisenstein integers. 5 or 6 triangles will meet at each vertex.

Thurston proved a remarkable fact: using this method, you can get all triangulations of the 2-sphere where 5 or 6 triangles meet at each vertex. (By Euler’s formula or the Gauss–Bonnet theorem, there will always be 12 vertices where 5 triangles.)

In the above example, Gerard Westendorp got a polyhedron called the hexagonal antiprism.

But in my AMS Notices column, I pose the following puzzle: how can you get the regular icosahedron?

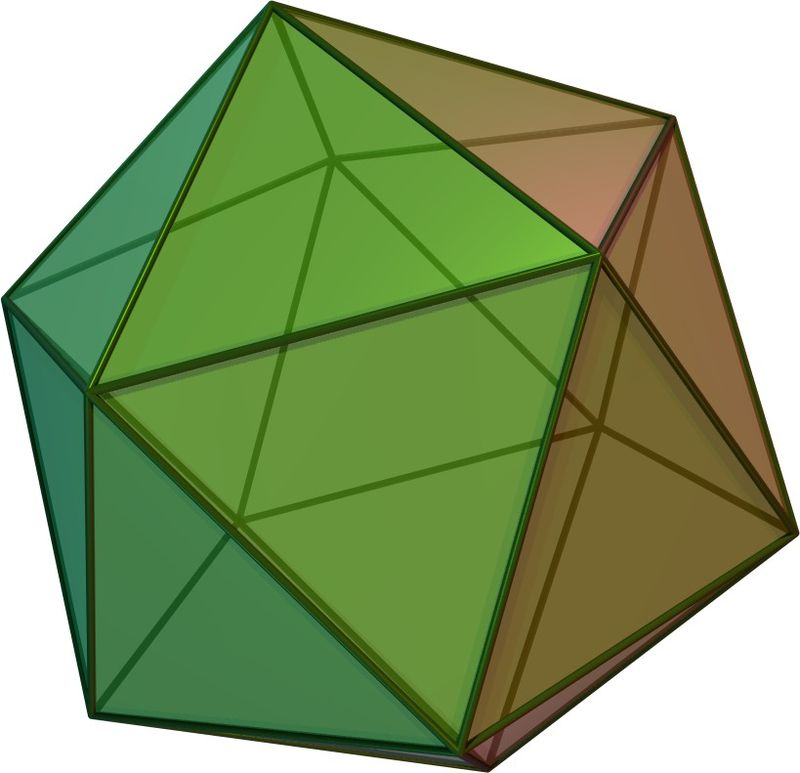
Here is Westendorp’s answer:

Want to check it? Print it out, cut it out and fold it up!