The n-Category Café

There was a kind of pre-event yesterday, a colloquium for the Osaka University mathematics department which I gave:

The many faces of magnitude

If you’ve seen me give a general talk on magnitude then many of the slides will be familiar to you. But I want to draw your attention to a new one (page 27), which is a summary of recent and current activity in magnitude homology and is meant to whet your appetite for some of the talks this week.

Here’s that slide in plain text, shorn of the photos of the people involved:

What’s happening in magnitude homology?

• There is a relationship between magnitude homology and persistent homology — but they detect different information (Nina Otter; Simon Cho)

• Applications of magnitude homology to the analysis of networks (Giuliamaria Menara)

• A theory of magnitude cohomology (Richard Hepworth)

• Connections between magnitude homology and path homology (Yasuhiko Asao)

• A comprehensive spectral sequence approach that encompasses both magnitude homology and path homology (Yasuhiko Asao; Richard Hepworth and Emily Roff; also relevant is work of Kiyonori Gomi)

• A concept of magnitude homotopy (Yu Tajima and Masahiko Yoshinaga)

• New results on magnitude homology equivalence of subsets of $\mathbb{R}^n$, involving convex geometry (joint with Adrián Doña Mateo)

• And lots more… find out this week!

The first talk is by Emily Roff, based on her paper Iterated magnitude homology.

Emily began by reviewing some of the foundations.

The setup for magnitude is this:

• a monoidal category $V$
• a ring $K$
• a “size” homomorphism $|\cdot|: (ob(V),\otimes) \to (K, \cdot)$

and with some linear algebra, we get a notion of the magnitude of a finite $V$-category.

Categorifying, the setup for magnitude homology is this:

• a semicartesian monoidal category $V$
• a monoidal abelian category $A$
• a strong symmetric monoidal functor $\Sigma: V \to A$ (a “size functor”)

and with some homological algebra, we get a notion of the magnitude homology of a $V$-category (not necessarily finite).

The magnitude homology functor $V Cat \to A^{\mathbb{N}}$ is the composite of three functors:

$$V Cat \stackrel{M B^\Sigma}{\to} [\Delta^{op}, A] \stackrel{C}{\to} Ch(A) \stackrel{H_\bullet}{\to} A^{\mathbb{N}}$$

Here $C$ and $H_\bullet$ are standard, off-the-shelf functors: chain complexes and homology. The first functor, $M B^\Sigma$, gives the magnitude nerve construction, which takes a $V$-category $X$ as input and produces as output the simplicial object with $n$th object

$$\bigoplus_{x_0, \ldots, x_n \in X} \Sigma(X(x_0, x_1) \otimes \cdots \otimes X(x_{n - 1}, x_n)).$$

Its simplicial object structure is given in the usual nervy way, but here’s where we need the restriction that the monoidal category $V$ is semicartesian: the unit object is terminal. That’s needed in order to define the first and last face maps.

Emily then told us about partially ordered groups (which include Coxeter groups with the Bruhat order) and norms on groups (e.g. any generating set on a group determines a word-length norm), which induce metrics in the usual kind of way.

For a symmetric monoidal category $V$, a $V$-group is a one-object $V$-category whose underlying ordinary category is a group. E.g. if $V$ is $Cat$, $Poset$ or $Met$ then a $V$-group is a monoid $G$ in $V$ together with a function $(-)^{-1}: U(G) \to U(G)$ making the usual diagrams commute. (Here $U$ means the underlying set of the $V$-object $G$ and $Met$ is metric spaces with the $\ell^1$ product.) This is something more general than an internal group.

• Every partially ordered group is a group enriched in $Poset$. (The inversion map is basically never monotone.)

• Every normed group with conjugation-invariant norm gives a group enriched in $Met$. (The inversion map is 1-Lipschitz if the metric is symmetric.)

• A strict 2-group, i.e. a group object in $Cat$, is a special kind of group enriched in $Cat$. Strict 2-groups classify homotopy 2-types. For instance, any normal subgroup $N$ of a group $G$ gives a strict 2-group $G_N$ whose objects are the elements of $G$ and with arrows $(k, g) : g \to kg$ for $k \in N$ and $g \in G$. Mac Lane and Whitehead proved that $\pi_1(B G_N) = G/N$.

Now onto iterated magnitude homology.

In all the cases above, $G$ has a “second-order” enrichment, i.e. it’s enriched in $V$-$Cat$ where $V$ is one of $Set$, $(0 \to 1)$ and $[0, \infty]$. Iterated magnitude homology is a homology theory of categories enriched in $V$-$Cat$.

A short digression. Any 2-category has a classifying space. There are a couple of approaches to this: Duskin’s and Street’s on the one hand, and Segal’s on the other. Bullejos and Cegarra proved that the two classifying space constructions are equivalent.

Now let $V$ be a semicartesian monoidal category and $\Sigma: V \to A$ a strong symmetric monoidal functor.

Proposition: the magnitude nerve defines a strong sym mon functor $$MB^\Sigma: VCat \to [\Delta^{op}, A],$$

which means we can use it as a size functor!

The double magnitude nerve of a $(V Cat)$-category $X$ $$MB^{MB^\Sigma}(X) \in [\Delta^op \times Delta^op, A].$$ The iterated magnitude nerve of $X$ is the diagonal part of this bisimplicial object, and its homology is called the iterated magnitude homology $IMH_\bullet(X)$. (The role of the diagonal here recalls the Segal construction mentioned above.)

Examples/theorems:

• For a $Cat$-group $G$, we have $IMH_1(G) = \pi(G)_{ab}$.

• For a normal subgroup of a group $G$, we have $IMH_1(G_N) = (G/N)_{ab}$

• For a preordered group $G$, we have $IMH_1(G) = (G/P_\sim)_{ab}$, where $P_\sim$ is the subgroup of elements $g$ such that $g \leq e \leq g$.

• Take a $Met$-group $G = (G, d)$. An element $g$ of $G$ is primitive if there is nothing strictly between it and the identity $e$ (“between” in the sense of the triangle identity). In length grading $0$, we have $$IMH^0_\bullet(G) \cong H_\bullet(G)$$ (the ordinary group homology), and in length gradings $\ell \gt 0$, the abelian group $IMH^\ell_2(G)$ is freely generated by the conjugacy classes of primitive elements of norm $\ell$.

Summary: iterated magnitude homlogy captures “the homology of a classifying space”.

And for a group with a conjugacy-invariant norm, the iterated magnitude homology is sensitive to both the the topology of the classifying space and the geometry of the group under the norm.

From homological algebra to analysis, with Café regular Mark Meckes.

Mark began with some classical geometric inequalities…

• The isoperimetric inequality: for nice $A \subseteq \mathbb{R}^n$, the ratio $$vol_n(A)/(vol_{n-1}(\partial A))^{n/(n-1)}$$ is maximized when $A$ is a Euclidean ball.

• The Brunn-Minkowski inequality: for nice $A, B \subseteq \mathbb{R}^n$, $$vol_n(A + B)^{1/n} \geq vol_n(A)^{1/n} + vol_n(B)^{1/n}$$ or equivalently $$vol_n(\lambda A + (1 - \lambda) B) \geq vol_n(A)^\lambda vol_n(B)^{1 - \lambda}.$$

Notation: $A$ is a nonempty compact metric space, $M(A)$ is the set of signed Borel measures on $A$, and $P(A)$ is the set of Borel probability measures on $A$. For a signed measure $\mu$ and $x \in A$, write $$Z\mu(x) = \int_X e^{-d(x, y)} d\mu(y).$$

We’ll use two mutually consistent definitions of magnitude:

• If there exists $\mu \in M(A)$ such that $Z\mu$ has constant value 1 (a weight measure) then $Mag(A) = \mu(A)$.

• If $A$ is of “negative type” then $$Mag(A) \sup_{\mu \in M(A)} \mu(A)^2 /\int Z\mu d\mu.$$ This is also equal to $$\inf \|f\|^2_H$$ where the inf is over functions $f$ that have constant value 1 on A, $H$ is a particular function space on $X \supseteq A$, and details are omitted.

The maximum diversity of $A$ is $$Div(A) = \sup_{\mu \in P(A)} \Biggl( \int_X (Z\mu)^{q - 1} d\mu \Biggr)^{1/(1 - q)}$$ where $q \in [0, \infty]$ and the right-hand side turns out to be independent of $q$ (a theorem of Emily Roff and myself; the finite case is due to Mark and myself).

Basic, easy inequalities:

• If $A \subseteq B$ then $1 \leq Div(A) \leq Div(B) \lt e^{diam(B)}$.

• If $A \subseteq B$ and $B$ is of negative type then $1 \leq Mag(A) \leq Mag(B)$.

• The fundamental inequality: if $A$ is of negative type then $Div(A) \leq Mag(A)$.

• Magnitude is lower semicontinuous on spaces of negative type: if $A_k \to A$ in the Gromov-Hausdorff topology then $$Mag(A) \leq liminf Mag(A_k).$$ (There are examples where this inequality is strict.)

Lower bounds for maximum diversity

• It’s pretty straightforward to show that $$Div(A) \geq \frac{vol(A)}{n!vol(B)}$$ where $A$ is a subset of an $n$-dimensional normed space, whose unit ball is called $B$.

• The packing number $P(A, \varepsilon)$ is a notion of the “effective number of points” at a particular scale: the largest number of points at distance $\geq \varepsilon$ from each other. Then $$Div(A) \geq \frac{P(A, \varepsilon)}{1 + P(A, \varepsilon)e^{-\varepsilon}}.$$

Upper bounds for maximum diversity

• Aishwarya, Li, Madiman and Meckes (in prepartion): if $A = \bigcup_i A_i$ then $Div(A) \leq \sum_i Div(A_i)$.

• The covering number $N(A, \varepsilon)$ is the smallest cardinality of an $\varepsilon$-net. The previous bullet point implies that $$Div(A) \leq N(A, \varepsilon)e^{2\varepsilon}.$$

• Packing and covering numbers are basically the same: $P(A, 2\varepsilon) \leq N(A, \varepsilon) \leq P(A, \varepsilon)$. From this we get the growth rate of $Div(t A)$ as $t \to \infty$ is the Minkowski dimension of $A$. We also get an expression in terms of maximum diversity for $$\sup_{\varepsilon \geq 0} \varepsilon\sqrt{\log(N(A, \varepsilon))}$$

Lower bounds for magnitude

Assume negative type now. Magnitude has been bounded below in two broad ways:

• $Mag(A) \geq Div(A)$, combined with lower bounds for $Div(A)$.

• Use monotonicity wrt inclusion, combined with exact computation via weight measures. E.g. in $\ell_1^n$, $$Mag \Biggl( \bigcup_{i = 1}^n [0, a_i] e_i \Biggr) = 1 + 2\sum a_i,$$ so if $\sum_i a_i = \infty$ then in $\ell_1$, $$Mag \Biggl( \bigcup_{i = 1}^\infty [0, a_i] e_i \Biggr) = \infty$$ (a simple example of a compact negative type space with infinite magnitude).

Upper bounds for magnitude

In principle, we have the bound $Mag(A) \leq \|f\|_H^2$ for any $f \in H$ with $f|_A \equiv 1$. But this is hard to implement in practice; the norm is a complicated Sobolev-type norm. Mostly, it has been used for asymptotic results such as those of Barceló and Carbery, of Leinster and Meckes, and (sharpest of all) of Gimperlein, Goffeng and Louca.

The major exception: for a subset $A$ of Euclidean space $\mathbb{R}^n$, $$Div(A) \leq Mag(A) \leq c_n Div(A)$$ where $c_n$ is a dimensional constant. This is a deep result in potential theory about the relationship between two different families of capacities. Meckes deduced that the growth rate of the magnitude of $t A$ as $t \to \infty$ is also the Minkowski dimension of $A$.

The most powerful way of bounding magnitude above ahs been to approximate $A$ by spaces $A_k$ with weight measures, then use lower semicontinuity. And can iterate this idea, approximating $A_k$ in the same way too.

To say more, need the idea of the intrinsic volumes on an $n$-dimensional normed space of negative type. There’s a Hadwiger-type theorem in this generality, giving well-defined intrinsic volumes $\mu_0, \ldots, \mu_n$ called the Holmes-Thompson intrinsic volumes. (There are some details here regarding choice of normalization that I’m not attempting to keep track of.)

• Take the normed space $\ell_1^n$. For compact, $\ell_1$-convex sets $K$, $$Mag(K) \leq \sum_{i = 0}^n 4^{-i} \mu_i(K) = vol([0, 1]^n + \tfrac{1}{2}K).$$

• Next approximate an $n$-dimensional subspace of $L_1 = L_1[0, 1]$ by subspaces of $\ell_1^N$. For an $n$-dimensional normed space $E$ of negative type and compact convex $K \subseteq E$, $$Mag(K) \leq \sum_{i = 0}^n (1/4)^i \mu_i(K) \leq \exp(\mu_1(K)/4)$$ and there’s a slightly sharper estimate in the special case of $K \subseteq \ell_2^n$, using the Alexandrov-Fenchel inequalities.

Applications:

Let $E$ be a fin-dim normed space of negative type.

• If $A$ is a compact subset of $E$ then $Mag(t A)$ is always finite and $Mag(t A) \to 1$ as $t \to 0$ (the one-point property).

• If $K$ is a compact convex subset of $E$ then $Mag(t K)$ is differentiable at $0$, and in fact $$lim sup_{t \to 0} \frac{Mag(t K) - 1}{t} \leq \tfrac{1}{4} \mu_1(K).$$ There are some known cases where the limit exists and is $\mu_1(K)$, in which case that’s the derivative at $t = 0$ of $Mag(t K)$.

• There is another application to Sudakov minoration. Using the bound on $\varepsilon \sqrt{\log N(K, \varepsilon)}$ mentioned earlier, Meckes gets a new proof of Sudakov’s minoration inequality.

• He also gets a short new proof of a special case of Mahler’s conjecture, making the assumption of negative type: $$vol(B) vol(B^\circ) \geq 4^n/n!$$ for the unit ball $B$ in an $n$-dimensional normed space of negative type. (Here $B^\circ$ is the polar set of $B$.)

There is also a Brunn-Minkowski-type inequality for maximum diversity, at least in one-dimensional space $\mathbb{R}^1$. In higher dimensions, there are only partial results known now. E.g. for subsets $A$ and $B$ of Euclidean space and $0 \leq \lambda \leq 1$, $$Div((1 - \lambda)A + \lambda B) \geq Div(A/\sqrt{2})^{1 - \lambda} Div(B/\sqrt{2})^\lambda.$$

Open questions

Mark finished with a bunch of open questions. There are some really basic unknowns here!

• Consider spaces $A$ of negative type. If $t \gt 1$, is $Mag(t A) \geq Mag(A)$? Is $Mag(A) \leq \# A$? If $t \lt 1$, is $Mag(t A) \geq Mag(A)^t$ (cf. Brunn-Minkowski)?

• In a normed space of negative type, is magnitude continuous of compact convex sets? We know it’s continuous in full dimension and at a point. But Mark suspects it’s false in general.

• Does $Mag(tK)$ have derivative $\mu_1(K)/4$ at $t = 0$, for compact $K$ in a finite-dimensional linear subspace $E \subseteq L_1$?

• What about a maximum diversity approach to the full Mahler conjecture?

• What about Brunn-Minkowski in arbitrary normed spaces, for either magnitude or maximum diversity?

• Can we get any inequalities from magnitude homology? This could be a powerful method!

The first talk not on magnitude is by Asuka Takatsu, in an area where optimal transport meets metric geometry. It represents joint work with J. Kitagawa.

Notation: $P(X)$ is the set of probability measures on a complete, separable metric space $X$.

The Monge-Kantorovich metric is also known as the Wasserstein metric. Data scientists are interested in it, but it’s expensive to compute. To fix this, one possibility is to use “sliced” M-K metrics.

For $1 \leq p \lt \infty$ and $1 \leq q \leq \infty$, we’ll define the M-K distance $MK_{p, q}$ on the space $P_p(\mathbb{R}^n)$ of measures with finite $p$th moment (i.e. $\int |x|^p d\mu(x)$ is finite). With this metric, $P_p(\mathbb{R}^n)$ is complete and separable, and it’s geodesic iff either $n = 1$ or $p = 1$.

We’re also going to consider the space $P(S^{n - 1} \times \mathbb{R})$, and the embedding of $\mathbb{R}^n$ into $S^{n - 1} \times \mathbb{R}$. For a probability measure $\sigma$ on $S^{n - 1} \times \mathbb{R}$, there’s an M-K metric $MK_{p, q}^\sigma$ on $P_{p, q}^\sigma(S^{n - 1} \times \mathbb{R})$, which is complete, separable (if $q \lt \infty$), and always geodesic.

The classical M-K distance $MK_p$ first arose in optimal transport. For probability measures $\mu$ and $\nu$ on $X$, we have the set of couplings between them, $$\Pi(\mu, \nu) = \{ \pi \in P(X \times X): \pi(A \times X) = \mu(A)  \text{and}  \pi(X \times A) = \nu(A)  \text{for all measurable} A\}.$$ For a cost function $c: X \times X \to \mathbb{R}$, the total cost of moving from $\mu$ to $\nu$ according to $\pi$ is $\int_{X \times X} c(x, y) d\pi(x, y)$, and the optimal transport problem is to minimize the total cost over $\pi \in \Pi(\mu, \nu)$. We put $$MK_p(\mu, \nu) = \inf_{\pi \in \Pi(\mu, \nu)} \biggl( \int_{X \times X} d(x, y)^p d\pi(x, y) \biggr)^{1/p}.$$ This is a metric on $P_p(X)$. The inf is achieved. Also, the metric space $(P_p(X), MK_p)$ is complete and separable, and it’s geodesic if $X$ is.

(Categorical point: the “gluing lemma” says there’s a canonical map $$\Pi(\mu, \nu) \times \Pi(\nu, \lambda) \to \Pi(\mu, \lambda).$$ It’s associative… and indeed, we have a category here. There may be some analytic/measure-theoretic requirements to get this to work. Mark Meckes tells me that Tobias Fritz calls this the “category of couplings”, and that Simon Willerton has pondered it too.)

Examples

• If $\mu = \tfrac{1}{n} \sum_{i = 1}^n \delta_{x_i}$ and $\nu = \tfrac{1}{n} \sum_i \delta_{y_i}$ then the minimizer is $\tfrac{1}{n} \delta_{(x_i, y_{\sigma(i)})}$ for some permutation $\sigma$.

• Let $\mu, \nu \in P(\mathbb{R})$. Then we transport $x$ to $y$ whenever $\mu(-\infty, x] = \nu(-\infty, y]$. (I guess this assumes $\mu$ and $\nu$ always give nonzero measures to nonempty open sets, otherwise that doesn’t make sense.)

This was just the warmup before Asuka got onto the main topic, sliced MK-metrics. For $\omega \in S^{n - 1}$, define $$R^\omega = \langle -, \omega \rangle: \mathbb{R}^n \to \mathbb{R}.$$ Then for $\mu \in P(\mathbb{R}^n)$, we get the pushforward measure $R^w_\#\mu \in P(\mathbb{R})$. (Asuka drew a picture here justifying the word “sliced”.) And now put $$MK_{p, q}(\mu, \nu) = \|MK_p(R_\#^\bullet \mu, R_\#^\bullet \nu)\|_{L^q(\sigma)}$$ where $\sigma$ is the uniform probability measure on $S^{n - 1}$. That is, unwinding the notation, $$MK_{p, q}(\mu, \nu) = \biggl( \int_{S^{n -1}} MK_p(R_\#^\omega \mu, R_\#^\omega \nu)^q d\sigma(\omega) \biggr)^{1/q}.$$ So if $q = 1$, then $MK_{p, q}(\mu, \nu)$ is the expected $MK_p$ distance between $\mu$ and $\nu$ after you project them down to a random line in $\mathbb{R}^n$. (This reminds me of mean width.)

$MK_{p,q}$ is a complete, separable metric on $P_p(\mathbb{R}^n)$. It’s topologically equivalent to $P_p(\mathbb{R}^n)$ with the metric $MK_p$, but not usually metrically equivalent. Typically it’s not geodesic, but it is if $n = 1$ or $p = 1$.

At this point I stopped being able to take notes, as this is new stuff to me and it took all my concentration to follow.

The second non-magnitude talk is on a topic that seems very closely linked to magnitude homology: persistent homology. The speaker is Emerson Gaw Escolar, and let me quote the beginning of his abstract:

In topological data analysis, one-parameter persistent homology can be used to describe the topological features of data in a multiscale way via the persistence barcode, which can be formalized as a decomposition of a persistence module into interval representations. Multi-parameter persistence modules, however, do not necessarily decompose into intervals and thus (together with other reasons) are complicated to describe.

(All the abstracts are on the programme page.)

Emerson’s talk will give general results on “interval approximation” and “interval global dimension” of finite posets, and he’ll also try to relate his observations to magnitude.

Setting: $P$ is a finite poset, $A = k P$ is its incidence algebra (i.e. the category algebra), and we consider the category $[P, vect_k]$ of finite-dimensional $k$-representations of $P$, also called persistence modules over $P$. The goal is to approximate them by simpler ones called interval-decomposable ones.

For example, if we consider a point cloud and increasing radii $r_1 \lt r_2 \lt \cdots \lt r_n$ of balls around the points, then our poset $P$ is $\bullet \to \bullet \to \cdots \to \bullet$. In this 1-dimensional situation, we can decompose into interval modules. That’s the standard structure theorem in persistent homology (the “bar code” theorem, I think it’s sometimes called).

(Emerson’s talk has lots of nice diagrams that I can’t reproduce here. The conference website has copies of slides from speakers willing to share them; maybe Emerson’s will appear there.)

Now for multiple parameters. For instance, you might have spatial parameters (e.g. radius) and a time parameter (data changing through time). Or there might be clustering depending on multiple parameters that govern how you’re doing your clustering.

In that first example, the spatiotemporal one, you have a different persistence diagram for each time point. So you need to think about how the birth/death events at one time point connect up to those at other time points. There’s no analogue of the decomposition into interval modules; we have a “wild representation type”.

Often, the poset $P$ for multiparameter situations is the grid $[m] \times [n]$ for some natural numbers $m, n$. (Here $[n] = \{0 \lt \cdots \lt n\}$.) We have at our disposal the adjointness relation $$Fun([m] \times [n], vect) \cong Fun([m], Fun([n], vect)).$$ But we’ll think about all finite posets $P$.

A full subposet $I$ of $P$ is an interval if it is connected and convex, in the obvious senses. (But “interval” in the poset literature means something different!) For example, there are intervals in $[m] \times [n]$ that aren’t the product of two posets of the form $[k]$. For an interval $I$, the interval representation $V_I: P \to vect_k$ is defined by $V_I(p) = k$ for all $p \in I$ and $V_I(p) = 0$ when $p \not\in I$; it’s defined on maps in the simplest possible way.

Now for approximation. Let $M$ be a persistence module. An interval-decomposable approximation of $M$ is a weakly terminal map from an interval-decomposable module to $M$. (So it’s something a bit like a projective cover.)

You can then consider “interval resolutions”: diagrams

$$\cdots \to X_2 \stackrel{f_2}{\to} X_1 \stackrel{f_1}{\to} X_0 \stackrel{f_0}{\to} M \to 0$$

such that $f_0$ is an interval approximation of $M$ and $f_i$ is an interval approximation of $ker f_{i - 1}$. And then you can talk about minimal interval resolutions, projective dimension of $M$, and global dimension of $k P$. I’ll skip some stuff here, but the path leads us deeper into representation theory and, in particular, the theory of dimensions of various types.

Relative homological algebra plays an increasing role in persistence, which Emerson mentioned briefly before skipping forward to interval global dimension of finite posets. He and his collaborators have computed the interval global dimension of $[m] \times [n]$ for small $m$ and $n$, and there are some interesting patterns, conjectures and theorems about monotonicity and stabilization.

Monotonicity theorem: if $Q$ is a full subposet of $P$ then $intgldim(Q) \leq intgldim(P)$. The proof uses the “intermediate extension” construction of Kuhn, Beilinson, Bernstein and Deligne, which is some analogue of convex hull and is a map from $vect^Q$ to $vect^P$ (not induction or coinduction).

Now, what about magnitude?

Is magnitude a measure of complexity, from the point of view of intervals?

Let $P$ be a finite poset. Form the full subcategory of interval representations of $P$. This is a finite category. What is its magnitude? Here we’re talking about the magnitude of the linear category $Intervals(P)$ of interval representations of $P$, i.e. the Vect-enriched category. What about the category of indecomposable projectives?

A paper by Joe Chuang, Alastair King and myself helps to answer the second question, with a fomrula for the magnitude of the linear category of indecomposable projectives over a suitably finite algebra. Also, for a finite poset $P$, $$Mag_\#(P) = Mag(IndecProj(k P))$$ where the LHS is the magnitude/Euler characteristic as a category, and the RHS treats $IndecProj(k P)$ as a linear category. This answers the question.

For the first question, I fell behind in taking notes, unfortunately.

It looks like the magnitude of $Intervals(0 \to \cdots \to n)$ is $n$, tested numerically for $n \leq 10$.

The last talk of the day is by me, on joint work with Adrián Doña Mateo.

Since I can’t very well live-blog my own talk, I’ll just drop a link to my slides and copy my abstract:

I will present a theorem describing when two closed subsets $X$ and $Y$ of Euclidean space have the same magnitude homology in the following strong sense: there are distance-decreasing maps $X \to Y$ and $Y \to X$ inducing mutually inverse isomorphisms in positive-degree magnitude homology. The theorem states that this is equivalent to a certain concrete geometric condition. This condition involves the notion of the “inner boundary” of a metric space, which consists of those points adjacent to some other point. The proof of the theorem builds on Kaneta and Yoshinaga’s structure theorem for magnitude homology.

Wow, much food for thought.

One perhaps exotic-sounding application that I just put on arXiv last week (so too late to be noticed) is to help find inputs that reach particular parts of a program’s control flow graph in cases that typical “fuzzing” tools are poorly equipped to handle: https://arxiv.org/abs/2311.17200

The first talk on Wednesday is by our generous host Masahiko Yoshinaga, and it’s one I’ve been especially looking forward to: the notion of magnitude homotopy. It’s based on a paper of his with Yu Tajima, and the full title is “Magnitude homotopy type: a combinatorial-topological approach to magnitude homology”.

The idea is to study magnitude homology groups via combinatorial topology, and more particularly, discrete Morse theory.

Starting from a poset $P$, we get its order complex, the simplicial complex $\Delta = \{(p_0 \lt \cdots \lt p_n): n \geq 0, p_i \in P\}$, hence a space $|\Delta|$.

Short review of discrete Morse theory. Take a set $V$ of vertices and a set $S \subseteq 2^V$. Then take $$M \subseteq \{(\sigma, \tau) \in S \times S: \sigma \subset \tau, |\tau \setminus \sigma| = 1\}.$$ We call $M$ an acyclic matching if:

• $\sigma \in S$ appears at most once
• there is no cycle $\sigma_1 \vdash \tau_1 \succ \sigma_2 \vdash \tau_2 \succ \cdots \vdash \tau_n \succ \sigma_1$, where $\sigma_i \vdash \tau_i$ means $(\sigma_i, \tau_i) \in M$ and $\tau_i \succ \sigma_{i + 1}$ means that $\tau_i \supset \sigma_{i + 1}$ and $|\tau_i \setminus \sigma_{i + 1}| = 1$.

We write $$C = \{\sigma \in S: \sigma  \text{does not appear in}   M\}$$ and this is called the set of critical cells.

The fundamental theorem of discrete Morse theory says that $|S|$ is equivalent to a CW complex constructed from the critical cells.

Example Take a triangle (empty) with vertices called 1, 2, 3. Its face poset $P$ has elements 1, 2, 3, 12, 13, 23 with the evident inequalities. Take $1 \vdash 12$ and $2 \vdash 23$. Then $3$ and $13$ are critical, and $P$ is homotopy equivalent to a circle with a point marked on it; the point is $3$ and the rest of the circle is $13$. (Masahiko drew some diagrams here, and this is my poor effort to put them into text.)

That’s the review of discrete Morse theory.

Now take a quasi-metric space $(X, d)$, i.e. a non-symmetric metric space. A directed graph is a typical example. We sometimes allow $\infty$ as a distance.

We’ll construct a poset suitable for the study of magnitude homology, the “causal order”. Masahiko wrote the words “potential on space-time” and then, as a motivating example, drew a very charming diagram.

There are some similar diagrams in his paper with Tajima; they’re space-time diagrams showing causal relationships and “cones of influence” or light-cones.

The causal order on $X \times \mathbb{R}$ is defined by $$(x_1, t_1) \lt (x_2, t_2) \iff t_1 \lt t_2  \text{and}  d(x_1, x_2) \leq t_2 - t_1.$$ Magnitude homology can be defined purely in terms of this poset.

Now we define causal intervals. Let $a, b \in X$ and $\ell \geq 0$. Write $$Cau^\ell(X: a, b) = [(a, 0), (b, \ell)]$$ where the square brackets mean the interval in the causal order.

(Masahiko also made some remarks on the physics angle, e.g. time-like and light-like relations in Minkowski geometry. The notion of causal relation goes back to a 1967 paper of Kronheimer and Penrose. There’s also a relevant 2018 paper of Kunzinger and Sämann on causal spaces, I guess this one.)

Next, magnitude homotopy type!

For $a, b \in X$, we have the order complex $\Delta Cau^\ell(a, b)$, i.e. the set of chains in $Cau^\ell(a, b)$. It has a subcomplex $\Delta'$ consisting of the chains $$(x_0, t_0) \lt \cdots \lt (x_n, t_n)$$ such that $$d(x_0, \ldots, x_n) \lt \ell.$$ (Here $d(x_0, \ldots, x_n)$ means $\sum d(x_{i-1}, x_i)$.) The magnitude homotopy type is the quotient simplicial complex. $$M^\ell(X: a, b) = \Delta Cau^\ell(X: a, b)/\Delta',$$ i.e. we collapse $\Delta'$ to a single point. Or we can consider everything as a space and take $$|\Delta Cau^\ell(X: a, b)|/|\Delta'|.$$ (Alternative point of view: consider the pair $(\Delta, \Delta')$.)

Theorem (Tajima and Yoshinaga)

• $\tilde{H}_n(M^\ell(X: a, b)) \cong MH_{n, \ell}(X: a, b)$, where $tilde{H}$ means reduced homology and the right-hand side is defined in the obvious way so that $MH_{n, \ell}(X) = \bigoplus_{a, b \in X} MH_{n, \ell}(X: a, b)$.
• $M^\ell(X: a, b)$ is a double suspension of $$\bigl|\Delta(Cau^\ell(a, b)\setminus\{(a,0), (b, ell)\})/\Delta'\cap\cdots\bigr|$$ (an object originally considered for graphs by Asao and Izumihara).

Example Let $X = \mathbb{Z}$, with points labelled as $p_n$ ($n \in \mathbb{Z}$). What is $M^4(X: p_0, p_0)$? Here the explanation absolutely needs the diagrams that Masahiko drew:

I’m afraid my notes can’t convey the dynamic live explanation here. The conclusion is that $M^4(X: p_0, p_0)$ is homotopy equivalent to $S^4 \vee S^4$, and $MH_{4, 4} \cong \mathbb{Z}^2$.

Now put $$M^\ell(X) = \bigvee_{a, b \in X} M^\ell(X: a, b)$$ where the wedge is a one-point sum. (The definition of $M^\ell(X: a, b)$ as a quotient $\Delta/\Delta'$ gives it a basepoint.) Then we have a functor $$M^\ell: Met \to Top_\ast.$$

Theorem If $X$ is finite and its similarity matrix $Z = (e^{-d(a, b)})$ is invertible then the family of reduced Euler characteristics $$\Bigl( \tilde{\chi}(M^\ell(X: a, b)) \Bigr)_{a, b \in X, \ell \geq 0}$$ determines the metric space $(X, d)$.

Sketch proof The inverse $Z^{-1}$ of the similarity matrix of $X$ has $(a, b)$-entry $$\sum_{\ell \geq 0} \tilde{\chi}(M^\ell(a, b))e^{-\ell}.$$

There’s also an inclusion-exclusion result: under conditions, $$M^\ell(X \cup Y) \vee M^\ell(X \cap Y) \simeq M^\ell(X) \vee M^\ell(Y).$$ And there’s a K¼nneth theorem too!

Next we have another non-magnitude talk on a topic that magnitudey people should probably be interested in: Jun O’Hara (web page) from Chiba University on residues of manifolds.

Let $X = (X, d, \mu)$ be either a closed submanifold of $\mathbb{R}^n$ or a compact body in $\mathbb{R}^n$ (“body” meaning of dimension $n$). (I think $d$ and $\mu$ are the distance function and metric on $X$.) We’re interested in the function $$z \mapsto B_X(z) = \int_{X \times X} d(x, y)^z d\mu_x d\mu_y \in \mathbb{C}$$ on $\mathbb{C}$. (This reminded me of capacities in potential theory, but that’s just a single integral with $x$ or $y$ fixed, and typically $z$ is real there. Here we’re integrating the capacity over the whole space.)

The function $B_X$ is holomorphic on the $z$ with real part $\gt - \dim X$, and can be analytically continued to $\mathbb{C}$ with only simple poles at some negative integers. It’s called a Brylinski beta function or the meromorphic (Riesz) energy function of $X$, and has been studied especially closely for knots.

I’m going to be briefer in these notes because the subject is less familiar to me and the talk slides are on the speaker’s web page.

We’re also interested, for a knot $K$, in the integrals $$\int_{K \times K} \frac{d x d y}{|x - y|}, \qquad E(K) = \int_{K \times K} \frac{d x d y}{|x - y|^2}$$ or rather their regularizations. The motivating problem: find a functional $e$ on the space of knots so that each knot type has an “optimal” representative minimizing $e$ within the knot type.

The meromorphic function $B_X$ produces two important quantities:

• Residues: $R_X(z_0)$, the residue at a pole $z_0$ of $B_X$. Examples: volume, total squared curvature, Willmore functional.

• Energy: $E_X(z)$ is $B_X(z)$ if $z$ is not a pole of $B_X$, and $$lim_{w \to z} \Bigl( B_X(w) - \frac{R_X(z)}{w - z}\Bigr)$$ if $z$ is a pole. Examples: knot energies, generalized Riesz energies.

For a compact manifold $(X, d)$ and $R \in \mathbb{C} \setminus \{0\}$, we have the magnitude operator $Z_X(R)$: $$u \mapsto \biggl( x \mapsto \frac{1}{R} \int_X e^{-R d(x, y)} u(y) d y \biggr).$$ Notice that the “scale factor” $R$ is a complex number! This idea was crucial to the work of Gimperlein, Goffeng and Louca on magnitude, in which (for example) they discovered that the magnitude function of a suitably nice space determines its surface area and Willmore energy.

There followed a summary of how to compute residues using the coarea formula, and an explanation of a relation with integral geometry (including work with Gil Solanes in Barcelona).

Theorem (Brylinski; Fuller and Vemuri) Take a closed $m$-manifold $M$ in $\mathbb{R}^n$, with $d(x, y) = |x - y|$. Then $B_M$ has poles at $-m, -m - 2, \ldots$, the first residue at $-m$ is essentially the volume of $M$, the second residue is essentially the Willmore energy for $m = 2$ and $n = 3$ (and more generally we get something to do with mean curvature).

If $n = 2, 3$ then the intrinsic volumes (including the Euler characteristic) of a compact body are given by a linear combination of residues and relative residues. For all $n$, the inclusion-exclusion property holds for residues of compact bodies if the boundary is regular enough.

Now consider geodesic distance on a closed submanifold of $\mathbb{R}^n$ (and can generalize to Riemannian manifold). The first residue is volume, the second residue is total scalar curvature, and the third residue is something more complicated involving the Ricci tensor and total scalar curvature.

Gimperlein, Goffeng and Louca showed that the magnitude function of such a manifold has an expansion whose coefficients involve volume and total scalar curvature, among other things. There’s more about this in a guest post they wrote last year.

A point to watch out for: different manifolds $X$ can have the same beta function $B_X$.

There are pairs of spaces distinguished by their magnitude functions, but not by their beta functions. E.g. consider $\ell_1 \gt \cdots \gt \ell_6$ such that all triples satisfy the triangle inequalities to make them the edge-lengths of a tetrahedron. A numerical experiment: there are 30 tetrahedra with the same beta functions, but different magnitudes.

On the other hand, there’s a pair of graphs distinguished by their beta functions, but both with magnitude function $$\frac{4 - 2q}{1 + q}$$ They’re $\bullet-\bullet-\bullet-\bullet$ and the 4-vertex graph that looks like $\mathsf{Y}$.

There’s a more general, interesting, question of comparing the residue method and the magnitude method to see which is more sensitive (in different situations) in detecting differences between spaces.

This afternoon’s first talk is by Masaki Tsukamoto, who’s giving an introduction to Gromov’s notion of mean dimension. I’ll paste in the abstract:

Mean dimension is a topological invariant of dynamical systems introduced by Gromov in 1999. It is a dynamical version of topological dimension. It evaluates the number of parameters per unit time for describing a given dynamical system. Gromov introduced this notion for the purpose of exploring a new direction of geometric analysis. Independently of this original motivation, Elon Lindenstrauss and Benjamin Weiss found deep applications of mean dimension in topological dynamics. I plan to survey some highlights of the mean dimension theory.

Tsukamoto works in dynamical systems and ergodic theory, and explained to us the idea of “dynamicalization”: take concepts and theorems in geometry, and find analogues in dynamical systems.

For example:

• number of points $\mapsto$ topological entropy

• pigeonhole principle $\mapsto$ symbolic coding

• New example by Gromov: topological dimension $\mapsto$ mean dimension.

Slogan:

Mean dimension is the number of parameters per unit time for describing a dynamical system.

Plan for the talk:

• Topological entropy and symbolic dynamics
• Mean dimension and topological dynamics
• Mean dimension and geometric analysis

Topological entropy and symbolic dynamics

For now, a dynamical system is a compact metrizable space $X$ with a homeomorphism $T: X \to X$, or equivalently, a continuous $\mathbb{Z}$-action. So then we can consider generalizing from $\mathbb{Z}$ to other groups.

For $\varepsilon \gt 0$, define $\#(X, d, \varepsilon)$ as the minimum number of open sets of diameter $\lt \varepsilon$ needed to cover $X$. Now for $N \geq 1$, put $$d_N(x, y) = \max_{0 \leq n \lt N} d(T^n x, T^n y).$$ This is a metric. The topological entropy of $(X, T)$ is $$h_{top}(X, T) = \lim_{\varepsilon \to 0} \lim_{N \to \infty} \frac{\log \#(X, d_N, \varepsilon)}{N}.$$

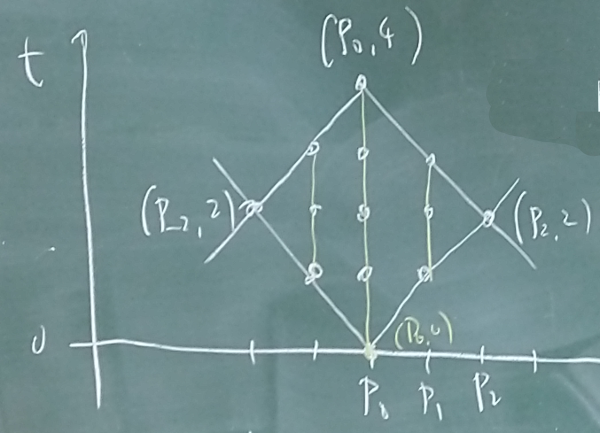
Example Let $A$ be a finite set, and consider the full shift on the alphabet $A$, which is the dynamical system $(A^\mathbb{Z}, \sigma)$ defined by $$\sigma((x_n)_{n \in \mathbb{Z}}) = (x_{n + 1})_{n \in \mathbb{Z}}.$$ Then we get $$h_{top}(\sigma) = \log|A|.$$

Generally, here’s a recipe for dynamicalization: given an invariant $Q(K)$ of spaces $K$, define a dynamical version $Q_{dyn}$ so that $$Q_{dyn}(K^\mathbb{Z}, \sigma) = Q(K).$$

We just did this for cardinality. What about the pigeonhole principle?

Some terminology. An embedding $(X, T) \to (Y, S)$ of dynamical systems is a topological embedding that commutes with $T$ and $S$.

What obstructions are there to existence of dynamical embeddings? Write $P_n(X, T)$ for the set of periodic points of period dividing $n$. If $(X, T)$ embeds in $(Y, S)$ then:

• $h_{top}(X, T) \leq h_{top}(Y, S)$
• $|P_n(X, T)| \leq |P_n(Y, S)|$ for all $n \geq 1$.

A partial converse holds! A closed subset $X$ of $A^\mathbb{Z}$ is called a subshift if $\sigma(X) = X$. The Krieger embedding theorem says that if $A$ and $B$ are finite sets and $X \subseteq A^\mathbb{Z}$ satisfies:

• $h_{top}(X, \sigma) \lt log|B|$,
• $|P_n(X, \sigma)| \leq |B|^n$ for all $n \geq 1$,

then $X$ embeds in $B^\mathbb{Z}$. Note that $A$ can be much larger than $B$!

This is the dynamical version of the pigeonhole principle, in the following version: a finite set $A$ embeds in a finite set $B$ iff $|A| \leq |B|$. We’ve just changed the appropriate notion of size.

Mean dimension and topological dynamics

A continuous map of metric spaces is called an $\varepsilon$-embedding if each fibre has diameter $\lt \varepsilon$. For compact $X$, define $Widim_\varepsilon(X, d)$ (width dimension) as the minimum integer $n$ for which $X$ can be $\varepsilon$-embedded into an $n$-dimensional simplicial complex.

The topological dimension of $X$ is $$\dim X = \lim_{\varepsilon \to 0} Widim_\varepsilon(X, d) \in \mathbb{N}.$$ This is a topological invariant!

Theorem (Menger and Nöbeling) If $\dim X \lt N/2$ then $X$ topologically embeds in $[0, 1]^N$.

Short digression: every compact metric space of topological dimension 1 can be topologically embedded into the Menger sponge (itself a subspace of $[0, 1]^3$).

The dynamical embedding problem Consider the shift map $\sigma$ on the Hilbert cube $[0, 1]^\mathbb{Z}$. When does a given dynamical system $(X, T)$ embed in $([0, 1]^\mathbb{Z}, \sigma)$?

Periodic points again provide an obstruction. If $(X, T)$ does embed in $([0, 1]^\mathbb{Z}, \sigma)$ then $P_n(X, T)$ embeds in $P_n([0, 1]^\mathbb{Z}, \sigma) \cong [0, 1]^n$.

Allan Jaworski proved in his 1974 PhD thesis that if $(X, T)$ is a finite-dimensional system (i.e. $\dim X \lt \infty$) and has no periodic point then $(X, T)$ embeds in $([0, 1]^\mathbb{Z}, \sigma)$. Then he left mathematics and became an artist.

What about infinite-dimensional systems? Joseph Auslander asked in 1988: can we embed every minimal dynamical system in $([0, 1]^\mathbb{Z}, \sigma)$ in the shift of the Hilbert cube? Minimal means that every orbit is dense, e.g. an irrational rotation of the circle. (This is a topological analogue of ergodicity.) Minimality clearly precludes the existence of periodic points: it’s a strong version of there being no periodic points.

The answer came about a decade later. In 1999, Mikhail Gromov introduced mean dimension, defined by

$$mdim(X, T) = \lim_{\varepsilon \to 0} \lim_{N \to \infty} \frac{Widim_\varepsilon(X, d_N)}{N}$$

Again, this is a topological invariant!

If $K$ is a nice space then

$$mdim(K^\mathbb{Z}, shift) = \dim K.$$

In this sense, mean dimension is the dynamicalization of topological dimension.

Earlier we had the slogan:

Mean dimension is the number of parameters per unit time for describing a dynamical system.

Here’s an informal (or maybe I mean formal?) explanation of the “per unit time” bit:

$$mdim(K^Z, \sigma) = \frac{dim K^\mathbb{Z}}{|\mathbb{Z}|} = \frac{|\mathbb{Z}| \times \dim K}{|\mathbb{Z}|} = \dim K.$$

In particular,

$$mdim([0,1]^\mathbb{Z}, \sigma) = 1.$$

But if $(X, T)$ embeds in $(Y, S)$ then one easily shows that

$$mdim(X, T) \leq mdim(Y, S)!$$

So if $mdim(X, T) \gt 1$ then it doesn’t embed in the shift of the Hilbert cube! Lindenstrauss and Weiss found in 2000 that for any $c \geq 0$, there is some minimal dynamical system of mean dimension. IN particular, there is some minimal system not embeddable in the shift of the Hilbert cube.

Lindenstrauss proved a further result that if a minimal dynamical system has mean dimension smaller than $N/36$ then it embeds in the shift of $([0, 1]^N)^\mathbb{Z}$. And, with the speaker Tsukamoto, he proved that this constnt 36 can’t be improved to 2 or less. Finally, Tsukamoto and Gutman proved that it can be improved to 2. So that completely settles that question.

Mean dimension and geometric analysis

The talk then went into more advanced material on Gromov’s original motivation, which was from geometry rather than symbolic dynamics. Gromov was interested in groups acting on manifolds $M$, with a PDE on M and its space $X$ of solutions. I decided to concentrate on listening to this part rather than taking notes.

This was a really wonderful talk, with beautiful conceptual structure. I now think of dynamicalization as a cousin of categorification and feel irresistibly drawn to try to find something new to dynamicalize.

Back to magnitude, with Luigi Caputi from Torino/Turin.

The talks here have ranged from analysis to homological algebra to dynamical systems to geometry to category theory, but the theme for this one is representation theory.

Plan:

• Recap, problems, questions
• Representation theory of categories
• Applications

Recap, problems, questions

$G$ denotes an undirected graph, usually connected, and not simple (i.e. may have loops and multiple edges). But can generalize most things to directed graphs.

Such a graph is a metric space under the path metric.

Maps: there are the ordinary morphisms of graphs that send vertices to vertices and edges to edges. But we’ll concentrate on minor morphisms $\phi: G' \to G$. Such a thing is a function

$$V(G') \coprod E(G') \coprod \{\ast\} \to V(G) \coprod E(G) \coprod \{\ast\}$$

such that

• vertices to go vertices and $\phi(\ast) = \ast$

• if $e \in E(G')$ is incident to $v, w$ then:

  • $\phi(e) = \ast$, or
  • $\phi(e) = \phi(v) = \phi(w)$, or
  • $\phi(e) \in E(G)$ and it is incident to $\phi(v), \phi(w)$

• $\phi^{-1}(E(G))$ maps bijectively to $E(G)$

• $\phi^{-1}(v)$ is a tree for all $v$.

It is a contraction if $\phi^{-1}\{\ast\} = \{\ast\}$. Write $Graph$ for the category of graph and minor morphisms, and $CGraph$ for graphs and contractions.