On the Magnitude Function of Domains in Euclidean Space, II
Posted by Simon Willerton
joint post with Heiko Gimperlein and Magnus Goffeng.
In the previous post, On the Magnitude Function of Domains in Euclidean Space, I, Heiko and Magnus explained the main theorem in their paper
(Remember that here a domain in means a subset equal to the closure of its interior.)
The main theorem involves the asymptoic behaviour of the magnitude function as and also the continuation of the magnitude function to a meromorphic function on the complex numbers.
In this post we have tried to tease out some of the analytical ideas that Heiko and Magnus use in the proof of their main theorem.
Heiko and Magnus build on the work of Mark Meckes, Juan Antonio Barceló and Tony Carbery and give a recipe of calculating the magnitude function of a compact domain (for an odd integer) by finding a solution to a differential equation subject to boundary conditions which involve certain derivatives of the function at the boundary and then integrating over the boundary certain other derivatives of the solution.
In this context, switching from one set of derivatives at the boundary to another set of derivatives involves what analysts call a Dirichlet to Neumann operator. In order to understand the magnitude function it turns out that it suffices to consider this Dirichlet to Neumann operator (which is actually parametrized by the scale factor in the magnitude function). Heavy machinary of semiclassical analysis can then be employed to prove properties of this parameter-dependent operator and hence of the magntiude function.
We hope that some of this is explained below!
[Remember that throughout this post we have is an odd positive integer.]
The work of Meckes and Barceló-Carbery
As a reader of this blog you might well know that magnitude of finite metric spaces is usually defined using weightings. Mark Meckes showed that the natural extension of magnitude to infinite subsets of Euclidean space can be defined using potential functions.
Before going anywhere, however, recall that the Laplacian operator is the differential operator on functions on given by .
Now, for a compact subset of with smooth boundary, a potential function for is a function with properties including the following:
- on ;
- (weakly) on ;
- is times differentiable on , and the th derivative exists in an -sense;
- as .
You can see an example below in the next section.
Barceló and Carbery built on the results of Meckes to show that for a compact convex domain in with smooth boundary the following recipe can be used to calculate the magnitude of .
First define to be the order differential operator on the boundary by
where means the derivative in the normal direction to the boundary.
The Barceló-Carbery Recipe for Magnitude. Suppose is a compact domain with smooth boundary.
Find a solution with as of the differential equation subject to the boundary conditions
The magnitude is then calculated by
Barcelo and Carbery actually stated their result for convex domains, but if we assume smoothness of the boundary then we can drop the convexity assumption.
The potential function of is related to the in the recipe by extending it to all of by taking it to be on .
Let’s have a look at a simple example that we’ll return to through this post.
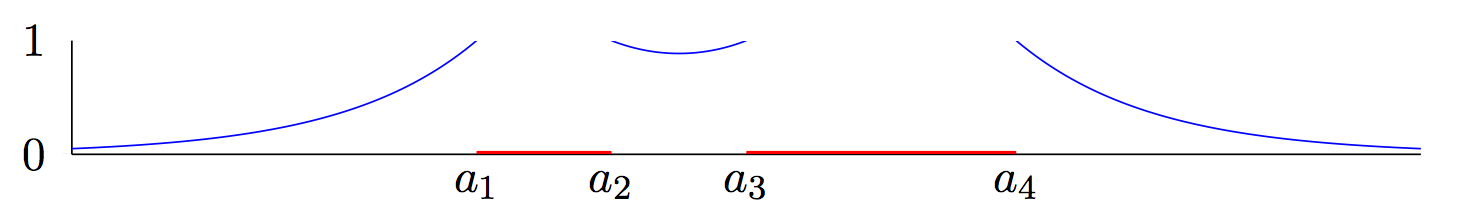
A one-dimensional example
Consider the union of two intervals in the line: for . The differential equation to solve in the Barceló-Carbery recipe is then
and the boundary conditions are
This is easy to solve by hand and you find the solution
Here is the graph of the potential function.
Now according to Barceló and Carbery’s recipe we can calculate the magnitude as
But it is easy to compute from the formula for above that
and so
which we can write as
A key point to note here is that to calculate the magnitude we don’t actually need to know the whole potential function , we only need to know certain of its derivatives at the boundary. So we start with a differential equation, specify sufficiently many derivatives at the boundary to give a unique solution and then find values of other derivatives at the boundary. This is a process which is well studied in the area of boundary value problems and is embedded in the notion of the Dirichlet to Neummann operator which we now look at.
The Dirichlet to Neumann operator
As you surely know, when solving a differential equation, you impose boundary conditions in order to pin down the solution. You might impose different boundary conditions in different situations. For instance, the classical Dirichlet boundary conditions for a problem of second order fix the value of the function on the boundary, whereas the classical Neumann boundary conditions fix the normal derivative of the function on the boundary.
For the calculating magnitude, the boundary value problem is of order , not of order . We think of the boundary conditions , which involves derivatives of order up to , as analogues of Dirichlet boundary conditions. To compute the magnitude we need to determine the derivatives of the solution of order up to , which we think of as analogues of Neumann boundary conditions.
Given Dirichlet boundary conditions we want to determine the corresponding Neumann boundary conditions. Let’s think what this means.
If you have a differential equation on a domain then specifying the boundary condition means imposing a set of equations of the form
where each is a differential operator on the boundary and each belongs to a suitable space of functions on the boundary. (We will avoid technicalities and complicated notation by using to stand for some space of functions, which might vary depending on context.)
When the boundary conditions give a unique solution to the differential equation – such as in the Barceló-Carbery Recipe – then for any other set of differential operators on the boundary there is a map, the Dirichlet to Neumann operator, between tuples of function on the boundary:
where is the unique solution to subject to the boundary conditions , .
This Dirichlet to Neumann operator will be a key ingredient in our approach to the parameter-dependent boundary problem for the magnitude function below.
In our toy one-dimensional example we have a single differential operator and , so this is a classical Dirichlet boundary condition; and we have being the normal derivative to the boundary and therefore this is a classical Neumann boundary condition.
Let’s see what this operator is in this example.
The Dirichlet to Neumann operator in our toy example
The boundary of consists of the four points , and we can identify the space of functions on the boundary with . We define the linear map , ie. as
where the function solves the boundary value problem
It is not difficult to compute that
Using the Barceló-Carbery recipe we have that the magnitude can be obtained from the sum of the entries of the matrix of : writing ,
as we had before.
Of course, in this case we did calculate the potential function in order to calculate . For domains in higher dimensions it is rarely possible to compute the potential function. This is the reason to introduce heavier guns from global analysis allowing us to study the operator without explicitly solving the problem.
What we are really interested is the magnitude function, its meromorphicity and asymptotic behaviour, so we need to study the above boundary value problems with a parameter which will represent the scale factor.
Introducing a parameter
Remember that the magnitude function, , is defined in terms of the magnitude of the dilates of , ie. for , where is the same space but with the metric scaled up by a factor of .
The Barceló-Carbery recipe for the magnitude from above can be generalized to include the scale factor and in such a way so that it is on an equal footing with the derivatives, essentially by replacing with . This approach is well studied in the literature on parameter-dependent pseudo-differential operators.
First define to be the order differential operator on the boundary given by
The Gimperlein-Goffeng Recipe for the Magnitude Function. Suppose that is a compact domain with smooth boundary.
Find a solution with as of the differential equation subject to the boundary conditions on :
The magnitude is then calculated by
The eagle-eyed amongst you will notice that setting does not immediately recover the Barceló-Carbery recipe. However, you can recover that with some algebraic manipulation and binomial identities.
Again we can use the Dirichlet to Neumann operator, but note that this will depend on a parameter . We think of as an operator valued function of the scaling parameter . If we start with the values of the differential operators on the boundary it should return the values of the operators , where if is odd and is is even. By the formula above we can use this operator to calculate the magnitude function.
Let’s look at the case of our toy example again.
The parameter-dependent operator in the toy example
In our running example of two disjoint intervals on the real line, , you can calculate to find
Again, writing , we compute the magnitude, using the Gimperlein-Goffeng recipe, as the sum of all the entries:
It is worth noting that you can see that the operator depends meromorphically on , rather than just being defined for , and an asymptotic expansion as . Therefore, the same holds for .
Proving the main theorem!
As described in the previous post, the main theorem of the paper is about a meromorphic extension of the magnitude function and about the asymptotic behaviour of the magnitude function as . As we’ve seen above, the magnitude function can be calculated from the parameter-dependent Dirichlet to Neumann operator . Now heavy machinery from geometric and semiclassical analysis – such as meromorphic Fredholm theorem and parameter-dependent pseudo-differential operators – can be used to extend to a meromorphic operator valued function and study its asymptotic expansion as . The properties of the magnitude function then follow.
That is probably enough for now, but in the next post, there should be a slightly less trivial example and some thoughts and comments of a more general nature.

Re: On the Magnitude Function of Domains in Euclidean Space, II
Thanks for the post!
Would someone like to explain — very roughly! — what semiclassical analysis is? It’s a funny name: you don’t hear people talking about semiclassical music. But it seems to have a particular meaning.