The n-Category Café

BV-Formalism, Part X: Symplectic Structures

Posted by Urs Schreiber

Last time in part IX (part I, II, III, IV, V, VI, VII, VIII) I finally started moving from discussion of the purely differential graded $\infty$-algebraic structure underlying BV-quantization towards those ingredients which make BV theory into BV theory: the BV-Laplacian, the antibracket, the master equation.

In the last installment I had reviewed Witten’s old (but not at all particularly wide spread, it seems) nice observation which indicated that all this new structure is just old familiar structure in unusual guise.

This time I want to add yet another facet to that. I had complained before at various places in our BV-discussions here that I am not entirely fond of the currently very popular perspective on BV-formalism in terms of supermanifolds. I said: if we are really talking about Lie $\infty$-algebroids, then it seems awkward to model all our internal imagery on supergeometry, just because the Chevalley-Eilenberg algebra of any Lie $\infty$-algebra happens to be that: a graded algebra. Instead, we should use Lie-algebraic imagery.

To add substance to this vague idea, I’ll here go through the standard constructions of the antifields- and antighosts- and anti-ghosts-of-ghosts-, etc.-parts, which is usually thought of as forming the cotangent bundle $T^* X$ of the supermanifold $X$ of physical configurations, by using instead the Lie $\infty$-algebraic point of view which we invoke in Lie $\infty$-connections and applications to String- and Chern-Simons $n$-transport, combined with the Clifford-algebraic point of view that Witten highlighted.

In this spirit I will

- identify the configuration space $X$ as the action Lie $n$-algebroid $(g,V)$ (here $g$ denotes an $L_\infty$-algebra and $V$ a module for it) obtained from the $L_\infty$-algebra $g$ of physical symmetries, symmetries of symmetries, etc., acting on the space $V$ of fields, whose dual algebra is the Chevalley-Eilenberg algebra $CE(g,V)$ (definition 2);

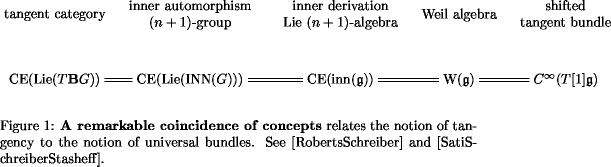
- identify the shifted tangent bundle $T X$ with the inner automorphism Lie $(n+1)$-algebroid $inn(gg,V)$, corresponding to the tangent category of the groupoid integrating $(g,V)$, whose dual algebra is the Weil algebra $$W(g,V) = CE(inn(g,V))$$ (definition 5, section 4.1.1)

- identify the shifted cotangent bundle, dually with the Clifford algebra generated by $CE(gg,V)$, which is like differential forms on $X$ together with the horizontal inner derivations on $\mathrm{W}(g)$.

Here “horizontal” is with respect to the universal $(g,V)$-bundle which dually reads $$\array{ CE(g,V) \\ \uparrow \\ \mathrm{W}(g,V) \\ \uparrow \\ inv(g,V) }$$ (table 1)

- identify the inner pairing (often addressed as the graded symplectic pairing in the supermanifold imagery) of these (pairing of fields with anti-fields, ghosts with anti-ghosts, ghosts-of-ghosts with anti-ghosts-of-ghost, etc) with the co-adjoint action of horizontal vector fields on vertical vertor fields in the universal $(g,V)$-bundle, which means in symbols that $$(\iota_X, \omega) := L_{\iota_X} \omega = [[d_{\mathrm{W}(g,V)}, \iota_X], \omega] \,.$$

If you are an expert on BV-formalism in supermanifold language, you’ll find nothing new here after you unwrap my ideosyncratic terminology. Still I think this is worthwhile. The main change in perspective is:

instead of thinking of BV-formalism as living in the cotangent bundle of a supermanifold of physical configurations, we realize it as living in the horizontal derivations on the universal groupoid $n$-bundle of the action $n$-groupoid of gauge transformations acting on physical fields.

It seems that after this introduction I am too tired to spell out the details today. Maybe tomorrow… I could leave this as an exercise to the reader. Most everything one needs to know (apart from what was discussed in previous BV-installments here) is in Lie $\infty$-connections.

Sorry.