On Hess and Lack on Bundles of Categories
Posted by Urs Schreiber
Kathryn Hess and Steve Lack are working on a Bundle Theory for Categories, various aspects of which are very close to the things we like to talk about here at the -Café.
John Baez kindly pointed out to me the very nice set of slides
Kathryn Hess
Bundle Theory for Categories
(slides) .
These slides discuss definitions and examples of this framework from 0-categories over 1-categories to 2-categories.
Here I shall walk through the material of that talk concerning 1-categories by exemplifying every step in terms of the example where the bundle of categories in question is the Atiyah groupoid of an (ordinary!) principal -bundle.
I believe this is helpful for putting these constructions in perspective.
I shall make use of the discussion of the Atiyah groupoid as given in
-Transport and Higher Schreier Theory
and
Curvature, the Atiyah Sequence and Inner Automorphisms,
but I try to make the discussion self-contained and elementary.
First: a fancy way to think of preimages
The entire construction which we shall be concerned with is maybe best understood as a categorification of the notion of preimages.
From Kindergarten we know that for and any two sets (let’s, following Hess and Lack, always make the least troublesome of all technical assumptions, which here means: assume these sets are finite) and for a surjective map from one to the other, the preimage operation is a map from the set to the set of all finite sets, which sends each element of to the set of all elements of which maps to .
Okay, you knew that before. So let’s be a little more fancy and put it this way:
Fact. There is a canonical bijection between surjective maps of sets and maps
Notice that this involves a funny shift in dimension: Above we regard as a mere 0-category (a set) itself. But of course is itself really a 1-category.
We can make that more explicit by refining the above statement slightly:
Let be the category of sets over . Objects are surjective maps as above, and morphisms are commuting triangles
Moreover, let be the standard category of functors, whose objects are functors from , regarded as a category with only identity morphisms, to the category of finite sets, which here I am calling just for fun. A functor from such a discrete category to an honest category is what deserves to be called a pseudo 0-functor.
Then, clearly, we have the
Fact. The category of 0-categories fibered over is canonically equivalent to that of pseudo 0-functors from to :
Of course I am stating this simple fact in such fancy language just in order to make it look more suggestive of what comes next.
Fibered Categories or 2-Preimages
The way we have stated our Kindergarten fact about pre-images above immediately makes us want to categorify it (that’s how it works: you know you understand something really when it categorifies seamlessly).
So we’d hope that the following is true, for any (1-)category:
Fact. The category of 1-categories fibered over is canonically equivalent to that of pseudo (1-)functors from to :
This is indeed true, when we impose the right condition for what it means for a category to be “fibered” over another. It turns out that the right condition is that the projection functor admits what are called cartesian lifts of morphisms in to morphisms in .
“Cartesian lift” is a bad (but standard) name for the following good idea:
Given any morphism down in , suppose we find some object sitting above That makes us want to find a lift of the entire morphism to . Even with its source fixed to be there may still be many choices. A cartesian lift is one which is universal among these choices:
suppose is a lift of the entire morphism . Clearly it deserves to be called universal among all such lifts with source given by , if any other one, say uniquely factors through it,
Warning: I am being sloppy, on purpose: this is the op-version of the usual definition. So I am not describing fibrations here, but op-fibrations, following Hess and Lack.
In any case, Hess and Lack use a slightly stronger version of this condition, where the above unique morphisms exist not just for lifts of morphisms with the same endpoint, but also for morphisms with different endpoints. Compare their slide 14.
As far as I understand, this extra condition is what makes the opfibration split and this is what makes them equivalent not just to pseudofunctors, but to strict (ordinary) functors . But experts should please correct me here.
Okay, before going on, I’ll look at a (simple) example for such a split opfibration of categories.
The example which I will apply all this to
We all know that a principal -bundle is a “bundle of sets” . In order to understand how passing to “bundles of categories” brings a connection on bundles into the game, it is helpful – I think – to consider the Atiyah groupoid of of . Here is how that is defined.
Let be some Lie group, and let be some smooth principal -bundle over a smooth space . From this data, we canonically get the following groupoids
Here
- is the pair groupoid of (the codiscrete groupoid over) : objects are points in , morphisms are pairs of points in .
- is the fundamental groupoid of : objects are points in , morphisms are pairs of points in , moprhisms are homotopy classes of paths between these points.
- is the smooth path groupoid of : objects are points of , morphisms are thin-homotopy classes of paths in
- is the Atiyah groupoid of : objects are the fibers of , morphisms are all maps between fibers which respect the -action on .
- is the groupoid corresponding to the adjoint bundle of groups of : objects are the fibers of , morphisms are all -equivariant fiber automorphisms.
The Atiyah groupoid as a split opfibrations
The Atiyah groupoid canonically comes with a projection down to the pair groupoid: this simply forgets the details of the morphism between two fibers of and simply remembers the points over wich the source and target fibers live.
This is a (simple) example for a split opfibration:
i) it is clear that for each morphism in at least one lift does exist: choose any morphism you like between the fibers of over the given endpoints (for instance simply by choosing one point in each fiber, that already fixes a -equivarint morphism between them!)
ii) since is a groupoid, where every morphism is invertible, every lift is already universal.
Notice that in this example each object has a unique lift: the point needs to be lifted to the fiber over it, which is one object (not a collection of objects as one might maybe trick oneself into thinking) of . So it’s really a very simple example only. But supposedly illustrative.
As John Baez teaches in his lectures (last time in Quantization and Cohomology (Week 23)) a functor which lifts each “path” (morphism) in to a morphism of the fibers over the endpoints is nothing but a choice of connection on . What kind of connection it is is determined by the nature of !
If is the pair groupoid of , then functoriality of implies that no matter which intermediate steps one makes to get from to , the composite of the corresponding fiber morphisms has to depend just on the endpoints and .
This means that we have a flat connection. And in fact, since is so puny, it really means that we have a flat connection on a trivial(izable) bundle .
We can allow slightly more freedom by using not just the pair groupoid, but the fundamental groupoid of . We may simply pull back the Atiyah groupoid along the canonical projection The resulting, slightly refined Atiyah groupoid, now has morphism which are pairs consisting of a choice of homotopy class of path between two points of , and a morphism between the fibers over these endpoints.
Now, a functor is still a flat connection, but possibly on a nontrivial bundle . (There may be nontrivial monodromies around non-contractible loops.)
You might still find this disappointing. No problem. Just keep pulling back the Atiyah groupoid along the chain of projections As we pull back the Atiyah groupoid to the full path groupoid we find its version where each morphism is a (thin-homotopy) class of a path between any two points, and a fiber morphism between the endpoints. Now functors are arbitrary connection on . You may require everything in sight to be smooth and indeed obtain a general theory of smooth bundles with smooth connection this way, all using functors: that’s described in full detail in Parallel Transport and Functor (and constitutes the first edge of The Cube).
Shifting everything in dimension
The above description of connections follows this slogan:
A connection is something which sends each path to an isomorphism of the fibers above its endpoints. Hence it transports the elements of the fibers along the path.
But this actually means that other things get transported along the paths as well. Most importantly, it is not just the fibers itself which thus get transported – we may also think of the automorphism groups of the fibers being transported.
For if here is a fiber and here an automorphism of it and if here is the parallel transport along a path to some other fiber , then here is an automorphism of the fiber : This automorphism of deserves to be called It arises from by conjugating with the parallel transport. You see, it is an inner morphism of the automorphism group of the fiber.
But recall that we are just looking at a very simple example here. In general there could be more than one object in sitting over any object down in . In that case we’d have not just an automorphism group of each fiber of an object downstairs, but an entire groupoid. Or even just any arbitrary (small, maybe) category.
So this means we ought be be looking at this adjoint action as actually being a functor which acts on fibers that we regard as categories. It is here that that curious shift in dimension appears in one of its many guises.
As a result, we get for every choice of “cartesian lift” of our (split op)fibered category which in my example was a functor with values not just in a 1-category, but in the 2-category of categories In my example this guy sends
– points in to the automorphism group of the fiber over , regarded as a one-object groupoid
– morphism, namely paths in to the functor which acts on these one-object groupoids by conjugating with the corresponding fiber isomorphism obtained from that connection
(If you think about it, this is, while different in detail, very closely related to the construction of the “differential” of the functor which I describe in section 3.2 of what I ideosyncratically call Arrow-Theoretic Differential Theory (I, II, III, IV). And of course that’s why John Baez emphasized Hess and Lack’s work.)
In more general situations, where things are not quite as well-behaved as in my little example, when the categories in question are not groupoids, for instance, and when they have more than one object over each object of the base, one needs to formulate the above slightly differently, though it essentially comes down to the same idea.
Hess and Lack give the general description of the functor corresponding to
- a (split opfibered) category
- and a choice of lifts
on their slide 24. If you think of everything there being invertible, you immediately see the Ad-action which I mentioned above.
There would be more to say. But since this has already become a rather lenghty entry, maybe I should stop here for the moment.

Re: On Hess and Lack on Bundles of Categories
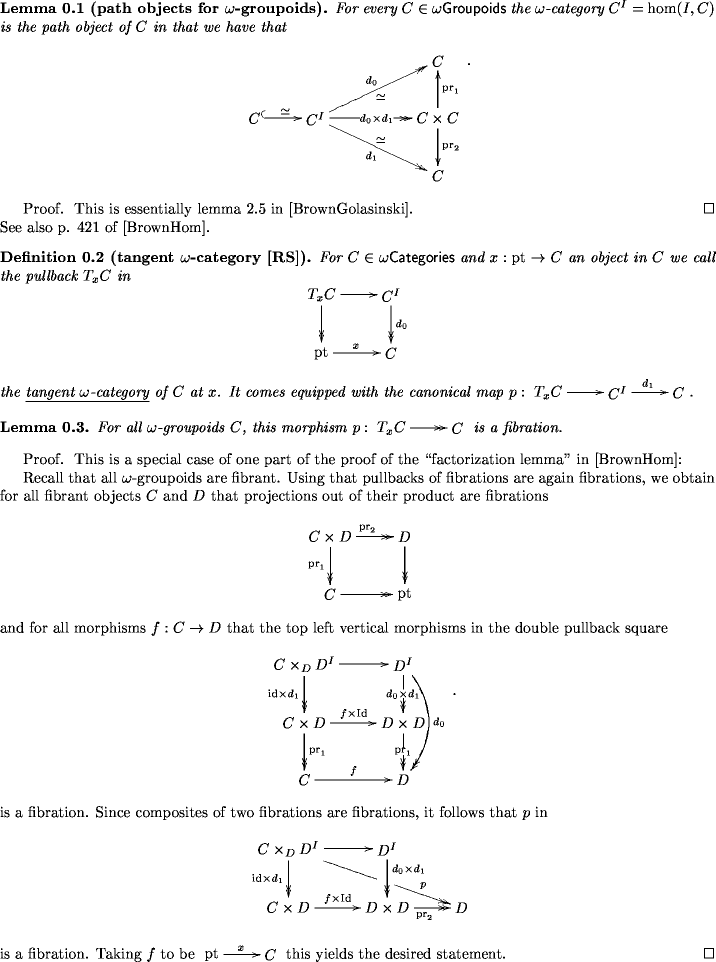
It was David Roberts who apparently first explicitly noticed the obvious: that the tautological or universal category bundle considered on slide 19 in Kathryn Hess’s talk is the tangent category bundle
Recall that for any -category the -category over the object of is defined to be the (strict) pullback and that it is known (section 7 here) at least up to that for every -group with corresponding one-object -groupoid the map is in fact the universal principal -bundle in that every principal -bundle over a space arises from this as the pullback along an -anafunctor
(In that section 7 this is just stated without detailed proof, but that can be seen here)
The theorem on Hess’s slide 21 says that the universal -bundle classifies (split op)-fibred categories:
(As Richard Lewis pointed out this statement connects to the more familiar formulation in terms of the Grothendieck construction in that is the result of applying the Grothendieck construction to ).
I would like to better understand the relation
Since the tangent category construction is obvious and natural for all , I am wondering if the following might be true in with respect to its standard model structure # (generalizing Lack’s model structure on 2Cat):
a) For every , is a fibration. For every object is a fibration. (?)
b) All fibrations in arise from pullbacks of fibrations of the form . (?)
I have some ideas about a), but not complete yet. About b) I am a bit clueless currently how to go about approaching the question.
One subtlety to expect for b) is that the pattern suggests that instead the correct statement rather wants to be something like that every fibration arises as a pullback of . Which would need further qualification as exists not in but in , I suppose.