Curvature, the Atiyah Sequence and Inner Automorphisms
Posted by Urs Schreiber
I am still trying to better understand -curvature and its relation to inner automorphism -groups.
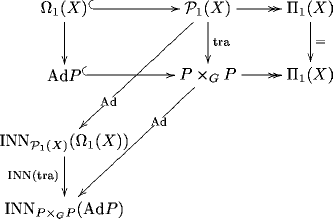
A while ago David Roberts emphasized that the parallel transport functor of a principal -bundle with connection can be thought of as inducing a morphism of short exact sequences of groupoids: from the sequence of path groupoids of to the integrated Atiyah sequence of .
Here I take a fresh look at the curvature 2-functor of a parallel transport 1-functor from this point of view, emphasizing the role played by inner automorphisms in this construction.
Curvature 2-Transport, the Atiyah Sequence and Inner Automorphisms
Let be the groupoid of thin homotopy classes of paths and is the fundamental groupoid.
Then we have a short exact sequence of path groupoids is the groupoid of closed paths (loops).
On the other hand, the integrated Atiyah sequence of the principal bundle is the short exact sequence (Here I am for simplicitly assuming that is simply connected, so that is the codiscrete grupoid over . If is not simply connected there is a relatively straightforward generalization of everything in sight.)
For any connection on , its parallel transport is a functor
This extends to a morphism of exact sequences of groupoids
(A)
One crucial point is that the groupoids in the middle of the sequences act by conjugation – hence by “inner” automorphisms – on those on the left.
This allows us to turn the parallel transport 1-functor into a functor This functor tells us how the monodromy groups of the original functor get transformed as we conjugate loops by paths.
The 2-groupoid is codiscrete at top level (all its Hom-categories are codiscrete). This is the 2-groupoid that the curvature 2-functor of takes values in. Codiscreteness of then induces the Bianchi identity on curvature.
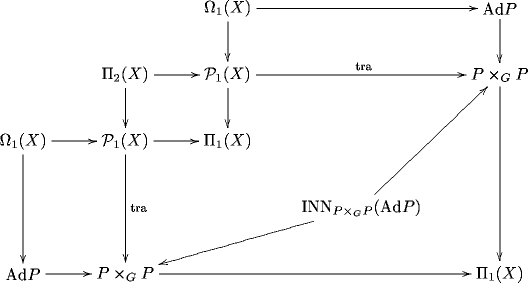
To see this – and that’s the point of this discussion here – we pull back the sequences (A) along themselves to obtain
Here the fundamental 2-groupoid appears in its incarnation where 1-morphisms are taken to be thin homotopy classes of paths. (And now I am even assuming that is 2-connected, for simplicity. Otherwise there is a more or less obvious way to adapt the entire construction.)
Then the two nested pullbacks give us a unique canonical factorization morphism. That’s our curvature 2-functor
So by regarding the connection as a morphism from the path sequence of the base to the Atiyah sequence of the bundle, also the discussion of curvature becomes a little more compelling.
More details in the above mentioned notes.


Re: Curvature, the Atiyah Sequence and Inner Automorphisms
Here is yet another way to see the same. This is closely analogous the the discussion of (twisted) vector bundles as morphisms into 2-vector transport 2-functors which I gave here.
Let be the 2-category whose
- objects are groups
- morphisms are left - right -spaces
- 2-morphisms are maps of --spaces, commuting with both actions.
This plays the analogous role for principal 1- and 2-bundles as does for vector 1- and 2-bundles.
Curvature of a principal 2-bundles with connection is a 2-functor which is such that it trivializes in the sense that there is a morphism into it.
Here is the 2-functor which sends everything to the identity on the trivial group.
The component map of – a functor on – gives the parallel transport .
To see this, notice that will send any surface
to
(Here a group with an automorphism in the subscript, denotes as a - space with the obvious left and the -twisted right action.)
Here , are the fibers of a principal -bundle over and is their automorphism group in the category of -spaces. This is canonically isomorphic to the group which appeared a lot in the above entry.
Notice how the fact that the 2-functor admits a “2-section” forces it to takes values in a sub-2-category of which in the above entry I denoted
This is equivalent to and hence, in particular, trivializable.
While is hence trivializable, it is the choice of the trivialization, , which encodes the interesting information:
one and the same curvature 2-functor may be trivialized by several non-equivalent morphisms and .
I am emphasizing this point since David Corfield was wondering about this here. The above is one example for the answer which I gave here.