Jacob Biamonte on Tensor Networks
Posted by John Baez
One of the unexpected pleasures of starting work at the Centre for Quantum Technologies was realizing that the math I learned in loop quantum gravity and category theory can also be useful in quantum computation and condensed matter physics!
In loop quantum gravity I learned a lot about “spin networks”. When I sailed up to the abstract heights of category theory, I discovered that these were a special case of “string diagrams”. And now, going back down to earth, I see they have a special case called “tensor networks”.
Jacob Biamonte is a postdoc who splits his time between Oxford and the CQT, and he’s just finished a paper on tensor networks:
- Jacob Biamonte, Algebra and coalgebra on categorical tensor network states.
He’s eager to get your comments on this paper, since while it’s aimed at people who already know about tensor networks, it uses a lot of things we talk about here: for example, algebras and coalgebras in symmetric monoidal categories! So, if you folks can’t understand this paper, or find it insufficiently precise, he’d like to hear from you.
Heck, he’d also like to hear from you if you love the paper! But as usual, the most helpful feedback is not just a pat on the back, but a suggestion for how to make things better.
Let me just get you warmed up here…
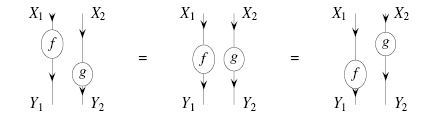
There’s a general theory of string diagrams, which are graphs with edges labelled by objects and vertices labelled by morphisms in some symmetric monoidal category with duals. Any string diagram determines a morphism in that category. If you hang out here, there’s a good chance you already know and love this stuff. I’ll assume you do.
To get examples, we can take any compact Lie group, and look at its category of finite-dimensional unitary representations. Then the string diagrams are called spin networks. When the group is , we get back the original spin networks considered by Penrose. These are important in loop quantum gravity.
But when the group is the trivial group, it turns out that our string diagrams are important in quantum computation and condensed matter physics! And now they go by a different name: tensor networks!
The idea, in a nutshell, is that you can draw a string diagram with all its edges labelled by some Hilbert space , and vertices labelled by linear operators. Suppose you have a diagram with no edges coming in and edges coming out. Then this diagram describes a linear operator
or in other words, a state
This is called a tensor network state. Similarly, if we have a diagram with edges coming in and coming out, it describes a linear operator
And the nice thing is, we can apply this operator to our tensor network state and get a new tensor network state . Even better, we can do this all using pictures!
Tensor networks have led to new algorithms in quantum computation, and new ways of describing time evolution operators in condensed matter physics. But most of this work makes no explicit reference to category theory. Jacob’s paper is, among other things, an attempt to bridge this cultural gap. It also tries to bridge the gap between tensor networks and Yves Lafont’s string diagrams for Boolean logic.
Here’s the abstract of Jacob’s paper:
We present a set of new tools which extend the problem solving techniques and range of applicability of network theory as currently applied to quantum many-body physics. We use this new framework to give a solution to the quantum decomposition problem. Specifically, given a quantum state S with k terms, we are now able to construct a tensor network with poly(k) rank three tensors that describes the state S. This solution became possible by synthesizing and tailoring several powerful modern techniques from higher mathematics: category theory, algebra and coalgebra and applicable results from classical network theory and graphical calculus. We present several examples (such as categorical MERA networks etc.) which illustrate how the established methods surrounding tensor network states arise as a special instance of this more general framework, which we call Categorical Tensor Network States.
Take a look and say what you think! Jacob will be watching this blog, and will respond to any questions you have.











Re: Jacob Biamonte on Tensor Networks
Seems to be some problem with the link to the paper.