This Week’s Finds in Mathematical Physics (Week 291)
Posted by John Baez
In week291 of This Week’s Finds, learn about Carlos Simpson’s new book. Listen to a crab canon on a Möbius strip. See how the Mandelbrot set mimics infinitely many Julia sets:
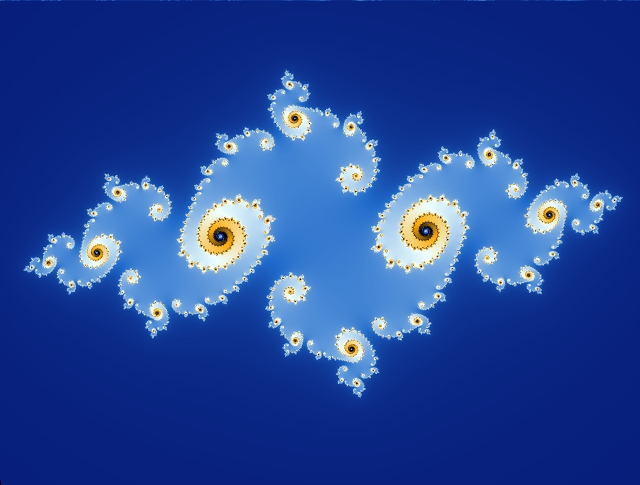

Then, continue our exploration of analogies between electrical circuits and other physical systems. This time we’ll meet the most important 2-ports and 3-ports — and get a tiny taste of Poincaré duality for electrical circuits.
Posted at January 23, 2010 4:51 AM UTC

Re: This Week’s Finds in Mathematical Physics (Week 291)
Did you see this question at Math Overflow, which asks for an interpretation of the claim that Mandelbrot sets are initial objects in the category of bifurcations?
If nothing else, I learned about the notion of multilimit from Mike Shulman’s comment as people there tried to interpret the claim.