Weakly Globular Double Categories: a Model for Bicategories

September 27, 2021

Weakly Globular Double Categories: a Model for Bicategories

Posted by Emily Riehl

$MathML-enabled post (click for more details).$

guest post by Claire Ott and Emma Phillips as part of the Adjoint School for Applied Category Theory 2021.

As anyone who has worked with bicategories can tell you, checking coherence diagrams can hold up the completion of a proof for weeks. Paoli and Pronk have defined weakly globular double categories, a simplicial model of bicategories which is a sub-2-category of the 2-category of double categories. Today, we’ll introduce weakly globular double categories and briefly talk about the advantage of this model. We’ll also take a look at an application of this model: the SIRS model of infectious disease.

$MathML-enabled post (click for more details).$

What is a weakly globular double category?

We’d like to find a sub-2-category of double categories that is biequivalent to the 2-category of bicategories. There are several reasons to want this model of bicategories, which we’ll talk about below. To compare bicategories and double categories, we’re going to consider them as objects in $[\Delta^{op}, \mathsf{Cat}]$ . This will enable us to develop a model of bicategories that lives between strict 2-categories and double categories. Two facts are going to help us develop this model:

Fact 1. $X \in [\Delta^{op}, \mathsf{Cat}]$ is the horizontal nerve of a double category iff the Segal maps $\eta_k: X_k \to X_1 \times_{X_0} \overset{k}{\cdots} \times_{X_0} X_1$ are isomorphisms.

Fact 2. $X \in [\Delta^{op}, \mathsf{Cat}]$ is the nerve of a strict 2-category iff $X_0$ is discrete and the Segal maps $\eta_k: X_k \to X_1 \times_{X_0} \overset{k}{\cdots} \times_{X_0} X_1$ are isomorphisms.

Since bicategories are weak 2-categories, it seems that we should be able to weaken the conditions of Fact 2 to get a model of bicategories in $[\Delta^{op}, \mathsf{Cat}]$ . By Fact 1, if we want this model to land in double categories, we should keep the requirement that the Segal maps are isomorphisms. Hence we want a property that’s a little less strong than $X_0$ being discrete.

We start with a weak gloublarity condition: we require that there is an equivalence of categories $\gamma: X_0 \to X^d_0$ , where $X^d_0$ is the discrete category of the path components of $X_0$ . In the corresponding double category, the weak globularity condition amounts to $X_0$ , the category of objects and vertical arrows, being a posetal groupoid; that is, every pair of objects has at most one arrow between them, which is an isomorphism.

When we define the fundamental bicategory of a weakly globular double category $X$ , meaning the bicategory associated to $X$ (see 8.1 here), we will define the objects to be the connected components of $X_0$ and the arrows to be the horizontal morphisms of $X$ . However, this creates a potential problem - how do we compose two morphisms whose codomain and domain are in the same component but don’t agree?

To solve this, we define an induced Segal maps condition: we require that for all $k \geq 2$ , $\gamma: X_0 \to X^d_0$ induces an equivalence of categories $X_1 \times_{X_0} \overset{k}{\cdots} \times_{X_0} X_1 \simeq X_1 \times_{X^d_0} \overset{k}{\cdots} \times_{X^d_0} X_1$ . This condition answers the composition problem because it allows us to fill in staircases, meaning paths of alternating horizontal and vertical arrows, with invertible 2-cells:

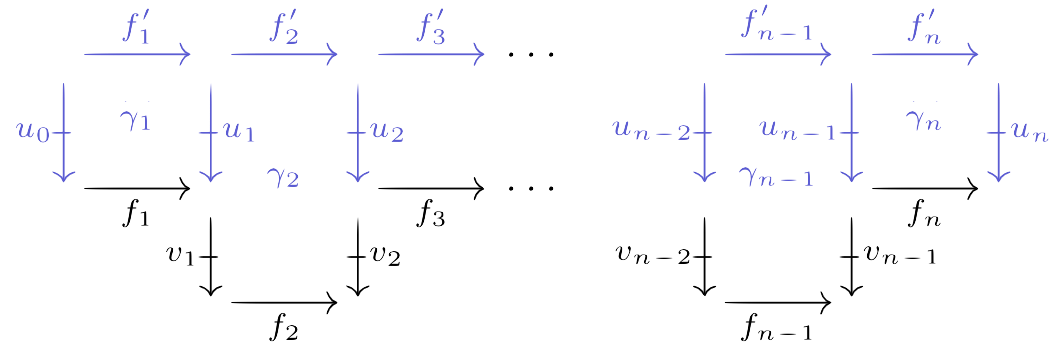
a filled-in staircase

This lift of the horizontal maps will give us our composite in the fundamental bicategory.

Finally we have our definition of a weakly globular double category: $X \in [\Delta^{op}, \mathsf{Cat}]$ is a weakly gloublar double category if and only if

the Segal maps are isomorphisms (i.e., $X$ is a double category);
(weak globularity condition) there is an equivalence of categories $\gamma: X_0 \to X^d_0$ , where $X^d_0$ is the discrete category of the path components of $X_0$ ;
(induced Segal maps condition) for all $k \geq 2$ , $\gamma$ induces an equivalence of categories $X_1 \times_{X_0} \overset{k}{\cdots} \times_{X_0} X_1 \simeq X_1 \times_{X^d_0} \overset{k}{\cdots} \times_{X^d_0} X_1$ .

We then have the following theorem, which you can read more about here:

Theorem 1. There is a biequivalence of 2-categories between the 2-category of weakly globular double categories with (double) pseudofunctors and vertical transformations and the 2-category of bicategories with normal homomorphisms¹ and icons :

$\mathbf{Bic}: \mathsf{WGDbl_{ps,v}} \simeq \mathsf{Bicat_{icon}} : \mathbf{Dbl}.$

Why study weakly globular double categories?

We’ll now try to convince you of why you should be interested in this model of bicategories. Between strict 2-categories, bicategories, and double categories, bicategories seem to show up most often in the wild. Even in pure mathematics, requiring that composition be strictly associative is often too strong of a condition. However, bicategories are also the most difficult to work with, since we have to check several coherence diagrams.

When we use weakly globular double categories, however, the coherence data is encoded in the combinatorial data of the simplicial structure. Hence when defining a bicategory, we can simply give a double category that adheres to the weak globularity and induced Segal maps conditions.

Even so, you may be thinking that while dealing with bicategory coherence diagrams can be unpleasant, they’re not so terrible that it’s worth learning a new model. The real power of this model, however, comes when we extend this notion to higher categories. Checking the coherence diagrams for tricategories is rather terrible, and there’s not even a clear notion of what the coherence diagrams should be for $n$ -categories for $n\geq 4$ . In her book, Paoli proves that weakly globular n-fold categories model weak n-categories. As in the case when $n=2$ , the weak globularity weakens the higher categorical structures of strict $n$ -categories. For $0 \leq k \leq n$ , instead of the $k$ -cells forming a set as they do in a strict $n$ -category, they form a $(n-k-1)$ -fold category which is suitably equivalent to a discrete one.

How do weakly globular double categories show up in applications?

To get a better understanding of what these weakly globular double categories can look like and what they might be used for, we want to introduce an example of marked paths of token placements in Petri nets. In these categories, morphisms only exist between objects with the same underlying Petri net, so we will focus on a category built on one specific net for this example: the SIRS model of infectious disease. We will call this weakly globular double category $\mathsf{Sirs^2}$ . Though we have not explicitly constructed this double category using the functor $\mathbf{Dbl}$ , we have drawn inspiration from the double category of marked paths in Paoli and Pronk, 8.2.

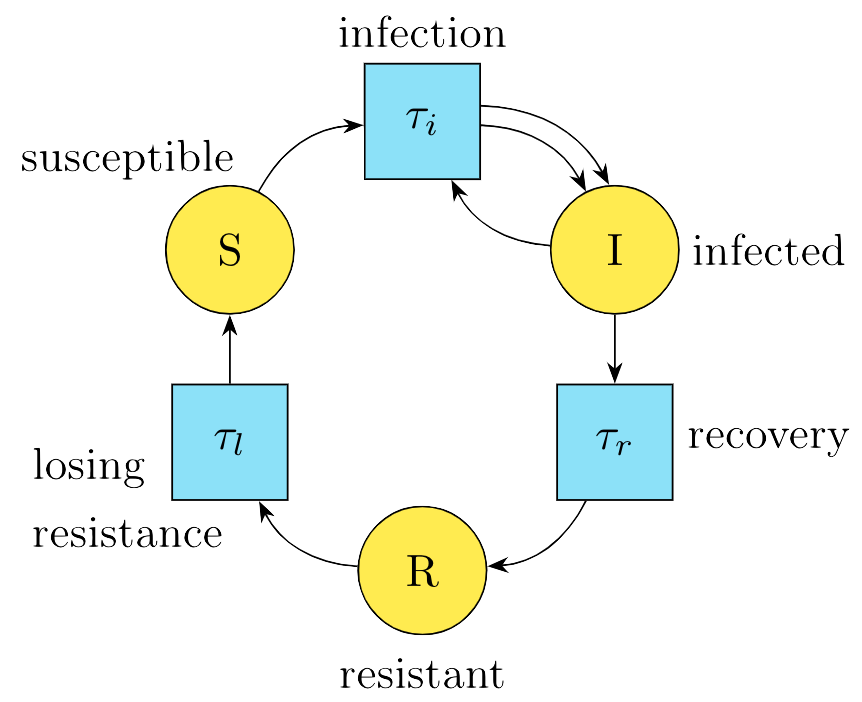
SIRS model

We would like to use token placements to model a group of three people which can be susceptible ( $S$ ), infected ( $I$ ) or resistant ( $R$ ). A token placement is a linear combination of places, i.e the placement $2S + I$ signifies two susceptible and one infected persons. A firing can happen at a transition, which are in our example infection ( $\tau_i$ ), recovery ( $\tau_r$ ) and loosing resistance ( $\tau_l$ ). Each transition has a source and a target which are again linear combinations of places and the condition for a firing is that enough tokens are placed at the transition’s source. In our example there could initially occur a firing at $\tau_i$ leading to the placement $S+2I$ or at $\tau_r$ , but not at $\tau_l$ .

Firings can be combined if the state after one firing is compatible with the next firing. This combination will have its own source and target which does not necessarily have to be the same as the first firing’s source and the last firing’s target. For example $\tau_l\tau_i\tau_r$ has source and target $I + R$ . The order of the firings is also significant.

We want to construct $\mathsf{Sirs^2}$ to capture paths of token placements which can occur through consecutive firings. In our example, one such path could be $2S+I,S+2I,S+I+R,S+2R$ , meaning we start with two susceptible and one infected person, which infects one of those two. Then one of the two infected people recovers, and next the other one recovers. One point to note is that we cannot distinguish tokens by means other than their placement, and so the scenario where the first infected person recovers first looks the same as the second infected person recovering first.

The objects of $\mathsf{Sirs^2}$ are marked paths, marking one token placement of the path. For our example, the marked paths $\begin{aligned} &\mathbf{2S+I},S+2I,S+I+R,S+2R\\ &2S+I,\mathbf{S+2I},S+I+R,S+2R\\ &\dots \end{aligned}$ are all unique objects in $\mathsf{Sirs^2}$ . Each object can be interpreted as a path of events that led to and follow from a chosen state of susceptible, infected, and resistant people.

Therefore we might be interested in all objects with the same fixed state, i.e. token placement. The vertical morphisms in $\mathsf{Sirs^2}$ are unique and exist between any two objects with the same marked token placement. For example, if we want to know the possible history and future for the state $2S+I$ of two susceptible people and one infected person, we could look at all vertical morphisms to or from the object $\mathbf{2S+I},S+2I,S+I+R,S+2R$ . Our potential vertical morphisms would include the following:

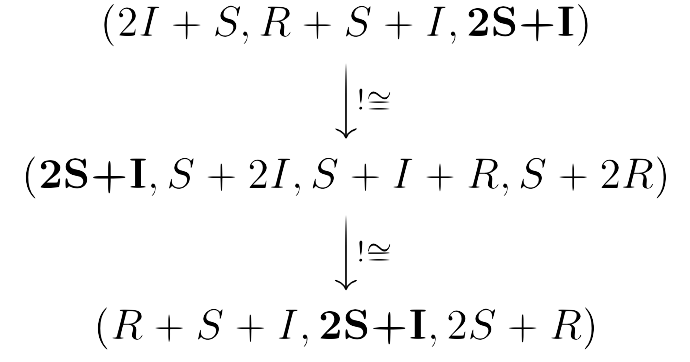
vertical morphisms

Horizontal morphisms only exist between pairs of objects with the same underlying path. There is exactly one horizontal morphism between each of these pairs, with the direction of the morphisms the same as the path’s direction. Horizontal morphisms are labeled by paths with two placements marked. For example $\mathbf{S+I},2I,\mathbf{I+R},S+I$ refers to the following morphism:

$(\mathbf{S+I},2I,I+R,S+I) \xrightarrow{(\mathbf{S+I},2I,\mathbf{I+R},S+I)} (S+I,2I,\mathbf{I+R},S+I)$

Note that there is no horizontal morphism in the opposite direction even though there is a path from $I+R$ to $S+I$ .

Using vertical and horizontal morphisms we get 2-cells of the following form:

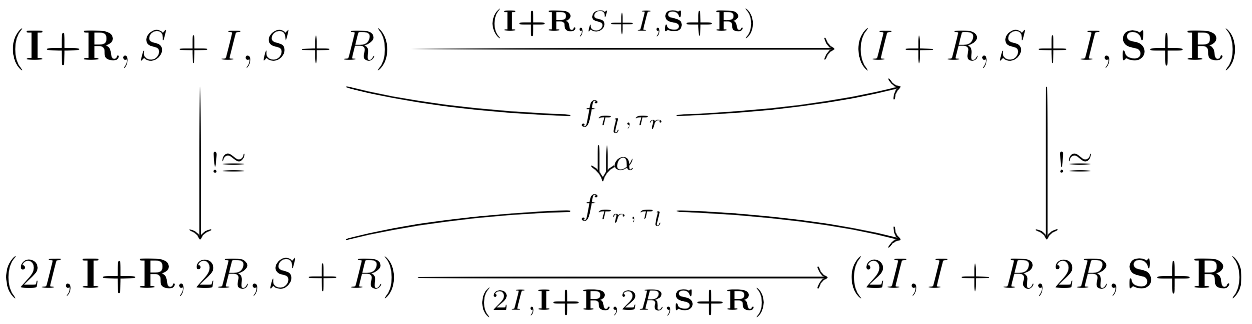
2-cells

In order to make our horizontal composition strictly associative, we have a chosen way to compose a string of horizontal maps which is encoded in the $f$ maps in the center of the diagram. The 2-cell witnesses the existence of alternate paths between two fixed states. In $\mathsf{Sirs^2}$ , all 2-cells are invertible.

We can see that $\mathsf{Sirs^2}$ is indeed a weakly globular double-category. The weak globularity condition is immediate, since the only vertical morphisms are isomorphisms and every pair of objects has at most one vertical morphism between them. In order to see that the induced Segal maps condition holds, consider an example staircase:

SIRS staircase

We fill in the top row of the staircase by creating a path of events built from the sequences encoded in the horizontal morphisms.

1 These are pseudofunctors of bicategories which preserve identities strictly. ↩

Posted at September 27, 2021 11:32 PM UTC

TrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/3355

25 Comments & 0 Trackbacks

Re: Weakly globular double categories: a model for bicategories

$MathML-enabled post (click for more details).$

Cool! You say “horizontal morphisms only exist between pairs of objects with the same underlying path” but I don’t get what the horizontal morphisms actually are in this example. You give an example of a horizontal morphism and maybe you even quickly say what they are in general, but I’m not getting it.

Posted by: John Baez on October 4, 2021 2:50 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

There are at least 4 ways to regard a 2-category as a double category:

vertically trivially
horizontally trivially
diagonally, via the double category of lax squares
diagonally, via the double category of oplax squares

In weak higher category theory, there is a tradition of using methods (1) and (2). For instance, a 2-fold complete Segal space is a double category which is vertically (or horizontally? depends on your conventions) trivial. Paoli and Pronk’s definition seems to be in a similar vein.

I only recently learned, though, that at least for strict 2-categories and strict double categories, the “diagonal” embedding via the double category of squares is also a fully faithful functor from $2Cat$ to $DoubleCat$ ! So it “ought” to be “just as good” for thinking about 2-categories in a double-categorical framework.

Not only that, but the “double category of squares” functor has both a left and a right adjoint, both of which are important:

The left adjoint is kind of related to the lax Gray tensor product – at any rate, when you apply the left adjoint to the double category given by “the walking square”, what you get is a lax square, just like taking the lax Gray tensor product of the arrow category with itself.
The right adjoint carries a double category $D$ to the “2-category of companions” $Com(D)$ . The 2-category $Com(D)$ has the same objects as $D$ . A 1-morphism in $Com(D)$ is companion / conjoint pair of a vertical morphism and a horizontal morphism in $D$ , with squares exhibiting them as a companion pair. The 2-morphisms are “the natural thing”.

I would really like to see the category of squares developed in a weak setting similarly to the way the vertically-trivial and horizontally-trivial embeddings have been. For one thing, this would give yet another model of weak $n$ -categories. For another, it would give us the language to talk about an important construction of double categories!

Posted by: Tim Campion on October 18, 2021 12:48 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

At one point I believed that the lax Gray tensor product of two 2-categories $C$ and $D$ is $L(H C \times V D)$ , where $H$ and $V$ are the horizontally- and vertically-trivial embeddings of 2-categories in double categories and $L$ is the left adjoint to quintets.

When you say that quintets are a fully faithful embedding, at what categorical dimension are you working? Do you mean a fully faithful embedding of 1-categories? Does it include higher structure?

Posted by: Mike Shulman on October 20, 2021 1:54 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

I learned this from Ehresmann and Ehresmann’s Multiple functors III. They prove that this functor is fully faithful in the strict setting for all $n$ . I would assume that that continues to be true $\infty$ -categorically, but I don’t think anybody has worked it out.

Posted by: Tim Campion on October 23, 2021 6:25 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

I don’t think the quintets functor is fully faithful (as a functor between 1-categories). It’s been a long time since I’ve thought about this, but my recollection is that if $A$ and $B$ are 2-categories, a double functor $Q(A) \to Q(B)$ amounts to the same thing as a pair of 2-functors $A \to B$ with an invertible icon between them.

Posted by: Alexander Campbell on October 24, 2021 9:18 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Yes, what Alexander says seems right to me. Since the category of such pairs of isomorphic 2-functors is equivalent to the category of 2-functors, one might hope for a biequivalence of 2-categories between, say, the 2-category of 2-categories, 2-functors, and icons, and a suitable 2-category of double categories — but how exactly to define the latter? Generalizing to the weak setting then seems rather fraught as one would have to deal with arbitrary (pseudo)natural transformations, not just icons.

Posted by: Mike Shulman on October 25, 2021 8:31 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

If one’s double categories have connections, one can always replace an arbitrary square with one with trivial vertical arrows. Then one does have a fully faithful functor (in fact an equivalence of categories to edge-symmetric double-categories), since the functors between double-categories have to preserve the connections.

Extra structure such as connections (one can also/instead add other stuff) are essential in my experience for working well with edge-symmetric double categories (and I was implicitly assuming some such extra structure was there in my first comment).

Posted by: Richard Williamson on October 25, 2021 10:39 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Ok, I’m trying to figure out where I got the notion that the “quintets” construction was fully faithful… this does not appear to be stated in any of the references I thought I might have gotten it from.

Is the distinction that Alex and Mike are drawing a distinction of strictness? I think not, right – icons differ from general cells by more than just strictness, right?

I think what has me confused is that the right adjoint to the “quintets” construction $Quint$ – the construction $Com$ sending a double category to its 2-category of companion-conjoint pairs – has the feel of a colocalization. But if the “quintets” construction is not fully faithful, then this can’t be the case.

So: What’s an example of an edge-symmetric double category $D$ such that $Quintet(Com(D))$ is not equivalent to $D$ ?

Posted by: Tim Campion on October 28, 2021 6:00 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Upon reflection, I’m feeling better about my mistake. Alexander’s description of double functors $Q(A) \to Q(B)$ appears to indicate that although I was wrong to assert we have a fully faithful adjunction between 1-categories, we nevertheless do have a fully faithful adjunction in an appropriately weak sense. And Mike’s objection seems to be that it would be difficult to prove this, not that it’s incorrect.

Obviously I have not through this carefully, but my naive guess would be that if you work with $(\infty,2)$ -categories and $\infty$ double categories in some non-algebraic model, then proving that $Quintets$ is a fully faithful adjunction would be no worse than anything else one does in higher category theory.

Posted by: Tim Campion on October 29, 2021 12:06 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

I would state my “objection” as that it’s difficult to formulate it, which comes before proving it. But I agree that since there is an “obvious” category of icon-isomorphic-pairs-of-2-functors (even if it’s not obvious how to obtain that as a hom-category) that’s equivalent to the category of single 2-functors, it “feels” like there should be some kind of full-faithfulness, if one found a correct way to formulate it. The problem is deciding exactly what the higher morphisms should be in your higher category of double categories.

Posted by: Mike Shulman on October 29, 2021 3:51 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Where by ‘identity square’ I mean, possibly confusingly (apologies for that), a square coming from an identity 2-globe, not (necessarily) a square which is a horizontal identity or a vertical identity. Similarly where I wrote that the squares of $Q(A')$ consist exactly of $\sigma$ plus ‘identities’: I mean $\sigma$ plus squares coming from identity 2-globes.

Posted by: Richard Williamson on December 2, 2021 12:02 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

I think that there in fact does not exist a right adjoint to the quintents construction if one works with plain edge-symmetric double categories. To see this, let $A$ be the 1-category which is the free-standing commutative square. Then the quintets construction applied to $A$ (viewed as a 2-category with only identity 2-arrows) gives a double category $Q(A)$ with a non-identity square, namely the one whose boundary is the commutative square and for which the 2-arrow of the quintets construction is the identity. Then for an edge-symmetric double category $B$ , there are as many functors $Q(A) \rightarrow B$ (I’ll use ‘functor’ for ‘morphism of double categories’) as there are squares in $B$ whose boundaries are commutative squares of 1-arrows. There might be many of these for a given $B$ .

But no matter how one tries to define a functor $R$ from edge-symmetric double categories to 2-categories, it is impossible for functors $A \rightarrow R(B)$ to pick out any non-identity 2-arrow of $R(B)$ . Hence if one is to have an adjunction between $Q$ and $R$ , $R$ must do something like take squares of $B$ whose boundaries are commutative squares of 1-arrows and throw them all away, as well as freely creating a new commutative square of 1-arrows for each. Intuitively I think it obvious at this point that such a construction cannot work. To be concrete, if one instead of $A$ takes some 2-category $A'$ which has a commutative square of 1-arrows but also a square with boundary this commutative square of 1-arrows but a non-trivial 2-arrow inside, it is impossible in general that functors $Q(A') \rightarrow B$ can be in bijective correspondence with functors $A' \rightarrow R(B)$ if $R$ has the convoluted description of the last but one sentence.

To get a right adjoint, again, one needs connections, or similar. Connections rescue the above situation, because with connections the non-identity square of $Q(A)$ can in fact be constructed from connections and identities, and functors $Q(A) \rightarrow B$ reduce to functors on the truncations to 1-categories.

One can see that connections or similar are needed in another way. I don’t exactly how you intended to construct your right adjoint, but the way I would describe it is that $R(B)$ should take a square and make it into a globe whose upper arrow is the composition of the north and east faces of the square, and whose lower arrow is the composition of the west and south faces. With this description, it is immediately clear that there is no way to define horizontal or vertical composition without connections.

Posted by: Richard Williamson on October 28, 2021 8:38 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

I hope I’m not mistaken in thinking that the Quintet construction

$Q : 2Cat \to DoubleCat$

sending a 2-category to its double category of squares, is left adjoint to the Companion construction

$2Cat \leftarrow DoubleCat : Com$

This is referenced (under “3. Applications” with some frustrating weasel words) on the nlab page for quintets and asserted straightforwardly on the nlab page for companions (last bullet under “4. Properties”). I was pretty convinced of this much when I read it.

Posted by: Tim Campion on October 29, 2021 12:00 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Yes, I still believe that $Quin \dashv Com$ between 2-categories and double categories, although I don’t know if I’ve seen it written out explicitly.

Richard is talking about something different (1-categories and edge-symmetric double categories), and I’m not convinced by his argument anyway. If $A$ is the walking commutative square, then $Q(A)$ has a lot of nonidentity squares, together with companion data, and I think that data is sufficient to witness the fact that “the” commutative square in it “is” in fact an identity (more precisely, that its transpose across companion pairs is an identity) in a way that’s preserved under a functor to any other double category $B$ .

Posted by: Mike Shulman on October 29, 2021 3:58 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Richard is talking about something different

Hmm, I didn’t realise I was! 1-categories are a full subcategory of 2-categories, and edge-symmetric double categories are a full subcategory of double categories. Therefore, if there is a right adjoint $R$ to the quintets functor $Q$ from 2-categories to double categories, functors (i.e. morphisms of double categories) from $Q(A)$ to $B$ have to be in (natural) bijective correspondence with functors (i.e. morphisms of 2-categories) from $A$ to $R(B)$ , for any 1-category $A$ and any edge-symmetric double category $B$ . I gave an example where it seems to me that this does not hold.

If A is the walking commutative square, then Q(A) has a lot of nonidentity squares

I think a lot is a bit of an exaggeration! It has some, yes, and I didn’t intend to suggest otherwise.

I think that data is sufficient to witness the fact that “the” commutative square in it “is” in fact an identity

That is indeed the question. I’d be happy to resolve this either way, I just brought the example for the purposes of discussion.

What is clear to me, as I wrote, is that if one has connections, some of the non-identity squares you refer to are in fact connections, and using only these plus identities, one can definitely express the square $s$ whose boundary is the commutative square as a composition. The point though is not really the fact alone that we can obtain $s$ as some composition; rather the point is that when working with connections, one’s morphisms between double categories have to preserve the connections, so if one can express $s$ as a composition of connections and identities, then one has no freedom to choose what $s$ is sent to when constructing a functor out of $Q(A)$ . As of now I don’t see that this reasoning can be carried out for plain double categories; but if I am proved to be wrong about this, I would no doubt be happy with that too, as the argument will probably be enlightening!

I don’t know if I’ve seen it written out explicitly

I believe the nLab took this from this paper of Paré and Grandis, Theorem 1.7. There is no actual proof in the paper. For reasons that are not appropriate to make public, I would definitely not take this result on faith without carefully checking it. I’ll try to carefully work through it myself when I get a chance. Companions are obviously not so dissimilar to connections, but with the important difference that they are not part of the structure.

If the result of Paré and Grandis is true and my example above is wrong, then I think it must be that the description of the right adjoint that I gave when one has connections, which to me is the obvious and natural one, and which I’m pretty sure is correct (it is basically also the same as the obvious functor from edge-symmetric double categories to strict 2-categories which picks out squares whose vertical arrows are identities, which is proven in the literature to be an equivalence of categories, hence a right adjoint), must be able to shown to be equivalent to it. That seems unlikely to me at first glance, but I could be missing something.

Posted by: Richard Williamson on October 30, 2021 7:56 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

edge-symmetric double categories are a full subcategory of double categories

I suppose you could define a category of edge-symmetric double categories that way, but it seems pretty weird. I would expect a functor between edge-symmetric double categories to have to act the same way on the two kinds of morphism. Otherwise, an “edge-symmetric double category” wouldn’t really have “the same” horizontal and vertical category, rather it would be “such that there exists an (unspecified) identity-on-objects isomorphism between the horizontal and vertical categories”.

rather the point is that when working with connections, one’s morphisms between double categories have to preserve the connections… As of now I don’t see that this reasoning can be carried out for plain double categories

I think it can. Any functor between double categories preserves companion pairs, since they are just diagrammatically defined.

If the result of Paré and Grandis is true and my example above is wrong, then I think it must be that the description of the right adjoint that I gave when one has connections… must be able to shown to be equivalent to it.

Yes, if I understand correctly then I think this is for instance Proposition 5.13 here.

Posted by: Mike Shulman on November 4, 2021 6:47 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Apologies for the slow reply. I have finally had time to take a look at the construction of Grandis and Paré, and I still think that their definition of $R$ does not handle my suggested example. I’ll try to go through a specific case in full detail.

Let $R$ be as defined in Grandis and Paré. The construction of $R$ does actually try to do something of the kind I suggested was inevitable in my earlier comment, namely ‘convert some squares to 1-arrows’. But it seems to me that it does not work for the reason I suggested in that earlier comment.

Indeed, let $A'$ be as in that comment: the unique 2-category whose 1-truncation is the walking commutative square, with arrows $f_{3} \circ f_{2} = f_{1} \circ f_{0}$ , say, and which has a single non-identity 2-globe, the source of which is $f_{1} \circ f_{0}$ , and the target of which is $f_{3} \circ f_{2}$ . Let $B$ , still in the notation of my earlier comment, be $Q(A')$ (though almost anything would do).

There are strictly more functors (i.e. morphisms of double categories) $Q(A') \rightarrow Q(A')$ than there are functors from the walking commutative square to itself: every morphism of 1-truncations (there are not that many, one either reflects the square in its diagonal or sends it to an ‘identity’ or a ‘connection’ square) extends to a functor $Q(A') \rightarrow Q(A')$ , whereas if one has the identity on 1-truncations, one has, in addition to the identity on squares, the freedom to send the square coming from the non-identity globe of $A'$ to the square coming from the identity 2-globe with the same boundary.

But the only companion pairs of $Q(A')$ are those coming from the ‘connection’ squares, and, there are no non-identity 2-globes between these companion pairs, i.e., up to isomorphism of 2-categories, one can take $R(Q(A'))$ to be the walking commutative square, viewed as a 2-category with only trivial 2-globes. Hence there are exactly as many functors (i.e. morphisms of 2-categories) $A' \rightarrow R(Q(A'))$ as there are functors from the walking commutative square to itself.

Hence there cannot be a bijection between the set of functors $Q(A') \rightarrow Q(A')$ and the set of functors $A' \rightarrow R(Q(A'))$ .

Of course it’s always possible there’s a mistake there somewhere, but that’s the argument I have in mind.

Yes, if I understand correctly then I think this is for instance…

As far as I see at the moment, this is something more akin to the kind of things that are needed to convert between a) my description of the right adjoint to the quintets functor when one has connections and b) the right adjoint to the inclusion of 2-categories into double categories by throwing in vertical identities.

The key way in which my description differs from Grandis and Paré’s is what is happening on 1-arrows. Mine is just the identity on 1-arrows, which, to me, feels like the only natural thing to do, and in category theory what is natural is usually what is possible! The idea to use companion pairs is a clever trick and insightful (it builds upon the way in which connections appear in the quintets construction), but this kind of cleverness doesn’t usually work in category theory!

I suppose you could define a category of edge-symmetric double categories that way, but it seems pretty weird. I would expect a functor between edge-symmetric double categories to have to act the same way on the two kinds of morphism.

I was just viewing edge-symmetric double categories as a full sub-category of double categories for the purposes of this discussion; I agree with you in general.

Posted by: Richard Williamson on November 25, 2021 10:28 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Just to forestall any confusion, the following was slightly imprecise…

the unique 2-category [with a certain description]

…as I did not specify how to define vertical composition of the single non-identity 2-globe (I’ll denote it $\sigma$ ) with itself. One has in fact two possible choices, namely $\sigma^{2} = \sigma$ or $\sigma^{2} = id$ , and it doesn’t matter which of the two one makes. One could equally well take the 2-globes to be freely generated by $\sigma$ , etc. Or one could begin with the walking non-commutative square instead of the walking commutative square, and then, in addition to having a single 2-globe from $f_{1} \circ f_{0}$ to $f_{3} \circ f_{2}$ , require a single 2-globe in the other direction (this addition is necessary if one wishes to be able to extend to $Q(A')$ the endo-functor of the walking non-commutative square coming from reflection in the diagonal).

Posted by: Richard Williamson on November 28, 2021 9:56 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

The issue is here:

there are no non-identity 2-globes between these companion pairs

The 2-globe $\sigma$ from the diagonal of the commutative square (which I’ll call $g = f_3 \circ f_2 = f_1 \circ f_0$ ) to itself also induces a square in $Q(A')$ with horizontal source and target $g$ and vertical source and target identities. This square then induces a 2-cell in $R(Q(A'))$ from the companion pair $(g,g,id)$ to itself.

Posted by: Mike Shulman on December 1, 2021 2:38 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Thanks for the reply. Could you elaborate on what you have in mind in the following sentence?

The 2-globe $\sigma$ from the diagonal of the commutative square (which I’ll call $g=f_3 \circ f_2=f_1 \circ f_0$ ) to itself also induces a square in $Q(A')$ with horizontal source and target $g$ and vertical source and target identities.

I (probably; it might be dependent upon what you mean) have some other comments, but I’d like first to clarify this.

What I am taking to be the definition of $Q(A')$ is that its horizontal and vertical 1-truncations are both the 1-truncation of $A'$ , and the squares of $Q(A')$ with boundary $(n, e, s, w)$ are exactly those 2-globes in $A'$ with source $e \circ n$ and target $s \circ w$ . The only non-identity 2-globe of $A'$ is $\sigma$ , and thus the squares of $Q(A')$ consist exactly of $\sigma$ along with identities (30 of them by my count: for each of the five 1-arrows of $A$ , four identity squares corresponding to choosing one of the north and east faces to be that arrow, and one of the west and south faces to be that arrow; two identity squares with north face $f_0$ and east face $f_1$ , with $g$ either on the west face or the south face; two identity squares which are the reflection of the latter two in the diagonal; two identity squares with west face $f_2$ and south face $f_3$ , with $g$ either on the north face or the east face; two identity squares which are the reflection of the latter two in the diagonal; the identity square whose boundary is the commutative square; and the reflection of the latter in the diagonal). The only one of these with $g$ on the north and south faces and identity west and east faces is the one coming from the identity 2-globe with source and target $g$ , and this one becomes an identity in $R(Q(A'))$ from the companion pair $(g,g,id)$ to itself.

It’s easy to become blind to something with this kind of thing, so maybe I’m overlooking something obvious; if you can clarify what you mean, I’ll proceed to what I expect to be my other comments.

Posted by: Richard Williamson on December 1, 2021 11:54 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Where by ‘identity square’ I mean, possibly confusingly (apologies for that), a square coming from an identity 2-globe, not (necessarily) a square which is a horizontal identity or a vertical identity. Similarly where I wrote that the squares of $Q(A')$ consist exactly of $\sigma$ plus ‘identities’, I mean $\sigma$ plus squares coming from identity 2-globes.

(I contrived to first post this in the wrong place further up the thread; perhaps if a moderator sees this, they will be kind enough to delete that misplaced comment).

Posted by: Richard Williamson on December 2, 2021 12:07 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

the squares of $Q(A')$ with boundary $(n, e, s, w)$ are exactly those 2-globes in $A'$ with source $e \circ n$ and target $s \circ w$ .

Yes, that’s right. The point is that a given 2-globe in $A'$ can give rise to many different squares in $Q(A')$ according to all the possible way of factoring its domain and codomain as $e\circ n$ and $s\circ w$ . You already observed this with the identities: there are only 9 identity 2-globes in $A'$ (I think), but they give rise to some larger number (I didn’t verify your count) of squares in $Q(A')$ .

Similarly, the single 2-globe $\sigma$ gives rise to many different squares in $Q(A')$ . There’s the one you seem to be thinking of, that arises by factoring the codomain $g$ as $f_3 \circ f_2$ and the domain $g$ as $f_1\circ f_0$ . But there’s another one that arises by factoring the domain $g$ as $g\circ id$ and the codomain $g$ as $id\circ g$ , and another one that arises from the factorizations $g\circ id$ and $g\circ id$ , and another one arising from the factorizations $g\circ id$ and $f_3\circ f_2$ , and another one… you get the picture.

Posted by: Mike Shulman on December 2, 2021 3:33 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

By the way, Ehresmann and Ehresmann’s work seems to only be known to the cognoscenti. I’ve only just started to skim a little bit. It seems like there’s a lot of good stuff there, and it probably deserves to be better-known.

Posted by: Tim Campion on October 23, 2021 6:28 PM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

In case you were not already aware, these kind of double categories are known as ‘edge-symmetric’. I tend to think of/refer to them as ‘cubical 2-categories’ (and, as you again may already know, there is a generalisation to any $n$ ).

In the same way that globes are not a test category but cubes are, I actually think that strict edge-symmetric $n$ -categories can model a lot of weak phenomena. Of course at $n=2$ one cannot really check this. For a considerable period of time from about 2009 to 2015 I worked a lot on viewing (strict) edge-symmetric $n$ -categories as models of weak $n$ -categories, but alas published nothing; but I’ll encourage anybody interested to pursue this, they are very rich models.

As I think you hint in another language, edge-symmetric $n$ -categories have a natural monoidal structure coming from the one on cubical sets, and this monoidal structure is closely related to the Gray tensor product.

Posted by: Richard Williamson on October 20, 2021 9:28 AM | Permalink | Reply to this

Re: Weakly Globular Double Categories: a Model for Bicategories

$MathML-enabled post (click for more details).$

Great reading! I found it pretty neat that you first described strict 2-categories (discrete $X_0$ ) and double categories (any $X_0$ ), only to put weakly globular double categories (homotopically discrete $X_0$ ) exactly between them.

I wonder if the absence of coherence diagrams makes this presentation of weak 2-categories any more suitable for computer implementations; thus far I have only seen strict structures (e.g. symmetric monoidal categories at CatLab). I am, however, too illiterate in these matters to tell.

Posted by: Daniel Teixeira on October 26, 2021 10:36 PM | Permalink | Reply to this

The n-Category Café

Skip to the Main Content

September 27, 2021