p-Local Group Theory
Posted by John Baez
I’ve been trying to learn a bit of the theory of finite groups. As you may know, Sylow’s theorems say that if you have a finite group , and is the largest power of a prime that divides the order of , then has a subgroup of order , which is unique up to conjugation. This is called a Sylow -subgroup of .
Sylow’s theorems also say a lot about how many Sylow -subgroups has. They also say that any subgroup of whose order is a power of is contained in a Sylow -subgroup.
I didn’t like these theorems as an undergrad. The course I took whizzed through them in a desultory way. And I didn’t go after them myself: I was into group theory for its applications to physics, and the detailed structure of finite groups doesn’t look important when you’re first learning physics: what stands out are continuous symmetries, so I was busy studying Lie groups.
Since I didn’t really master Sylow’s theorems, and had no strong motive to do so, I didn’t like them — the usual sad story of youthful mathematical distastes.
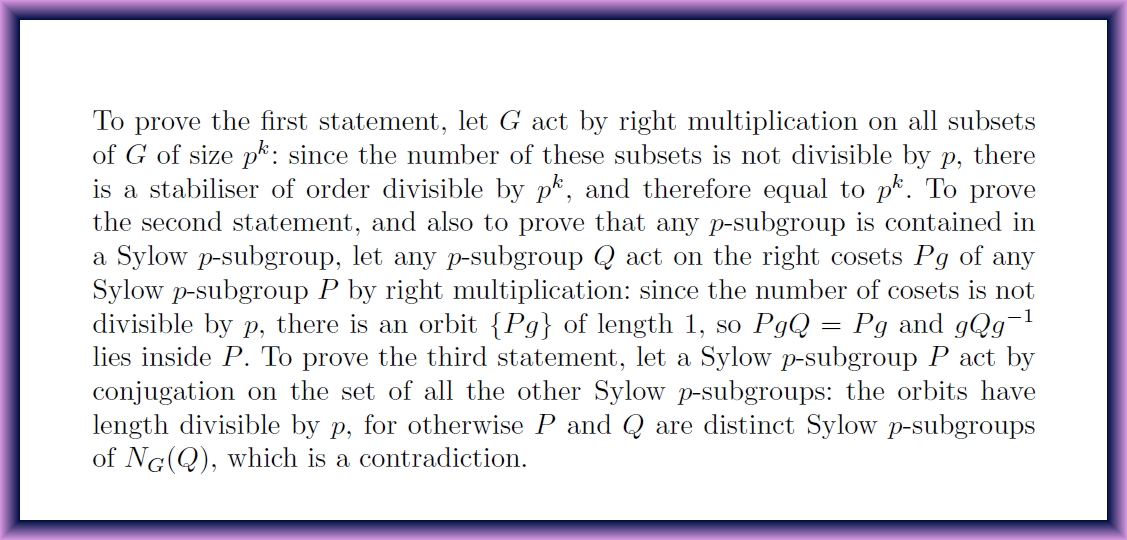
But now I’m thinking about Sylow’s theorems again, especially pleased by Robert A. Wilson’s one-paragraph proof of all three of these theorems in his book The Finite Simple Groups. And I started wondering if the importance of groups of prime power order — which we see highlighted in Sylow’s theorems and many other results — is all related to localization in algebraic topology, which is a technique to focus attention on a particular prime.
The answer is yes:
- Carles Broto, Ran Levi, and Bob Oliver. The theory of -local groups: a survey, Contemporary Mathematics 346 (2004), 51–84.
It’s a nicely written article, so it’s a bit pointless to summarize it, but still, here’s one of the first punchlines.
There’s a functor from to , called -completion. A map induces a homotopy equivalence iff it induces an isomorphism of cohomology groups .
So, -completion takes a space and destroys all information about it that’s not visible using cohomology with coefficients, while damaging that space as little as possible.
If you hand me a finite group , there’s a strong relation between the -completion of the classifying space and the Sylow -subgroups of . The paper explains this in detail.
The rough idea is that one can recover from a category where:
the objects are -subgroups of : that is, subgroups having order a power of ;
the morphisms are ways of conjugating one of these -subgroups and then including it in another.
This category is called the fusion category of at the prime . Since every -subgroup is contained in a Sylow -subgroup and all Sylow -subgroups are conjugation, the fusion category is equivalent to the full subcategory where:
the objects are subgroups of a fixed Sylow -subgroup of ;
the morphisms are ways of conjugating one of these -subgroups and then including it in another.
Roughly, you can get by taking the nerve of the fusion category and then -completing it. But this isn’t really true: we need a fancier category, similar to the fusion category, to make something like this true. In this fancier category the objects are not all -subgroups, but only certain special ones called ‘-centric subgroups’, and the hom-sets need to be adjusted a bit too.
There are, however, some results for which the fusion category is enough. For example, Cartan and Eilenberg showed that is determined by the fusion category of .
So I don’t yet see what’s really going on, but I’m encouraged that there’s something going on here. I would like, someday, to have a more conceptual — for example, a homotopical — understanding of what Sylow’s theorems are telling us. And I’d also like to understand the analogy between Sylow -subgroups of a finite group and maximal tori in a compact Lie group.
Do you have any clues that can help me? The simpler the better, if possible.


Re: p-Local Group Theory
There’s a generalisation of Sylow theory to finite ∞-groups, that is, ∞-groups with finitely many non-trivial homotopy groups which are all finite. See