Question on Condensed Matter Physics
Posted by John Baez
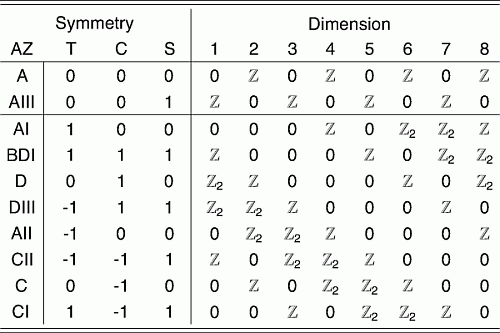
The tenfold way is a mathematical classification of Hamiltonians used in condensed matter physics, based on their symmetries. Nine kinds are characterized by choosing one of these 3 options:
- antiunitary time-reversal symmetry with , with , or no such symmetry.
and one of these 3 options:
- antiunitary charge conjugation symmetry with , with , or no such symmetry.
(Charge conjugation symmetry in condensed matter physics is usually a symmetry between particles - e.g. electrons or quasiparticles of some sort - and holes.)
The tenth kind has unitary “” symmetry, a symmetry that simultaneously reverses the direction of time and interchanges particles and holes. Since it is unitary and we’re free to multiply it by a phase, we can assume without loss of generality that .
What are examples of real-world condensed matter systems of all ten kinds?
Posted at January 23, 2023 7:43 PM UTC
p-n junctions
I think p-n junctions would be the tenth type. Not confident though. https://en.wikipedia.org/wiki/P%E2%80%93n_junction