Network Theory Talks at Oxford
Posted by John Baez
One of my dreams these days is to get people to apply modern math to ecology and biology, to help us design technologies that work with nature instead of against it. I call this dream ‘green mathematics’. But this will take some time to reach, since living systems are subtle, and most mathematicians are more familiar with physics.
So, I’ve been warming up by studying the mathematics of chemistry, evolutionary game theory, electrical engineering, control theory and information theory. There are a lot of ideas in common to all these fields, but making them clear requires some category theory. I call this project ‘network theory’. I’m giving some talks about it at Oxford.
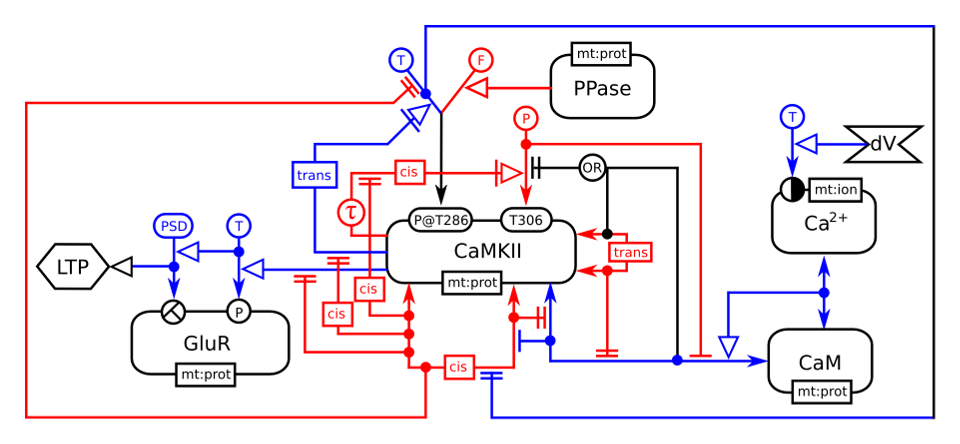
(This diagram is written in Systems Biology Graphical Notation.)
Here’s the plan:
Network Theory
Nature and the world of human technology are full of networks. People like to draw diagrams of networks: flow charts, electrical circuit diagrams, signal-flow graphs, Bayesian networks, Feynman diagrams and the like. Mathematically minded people know that in principle these diagrams fit into a common framework: category theory. But we are still far from a unified theory of networks. After an overview, we will look at three portions of the jigsaw puzzle in three separate talks:
I. Electrical circuits and signal-flow graphs.
II. Stochastic Petri nets, chemical reaction networks and Feynman diagrams.
III. Bayesian networks, information and entropy.
All these talks will be in Lecture Theatre B of the Computer Science Department—you can see a map here, but the entrance is on Keble Road. Here are the times:
• Friday 21 February 2014, 2 pm: Network Theory: overview. Also available on YouTube.
• Tuesday 25 February, 3:30 pm: Network Theory I: electrical circuits and signal-flow graphs. Also available on YouTube.
• Tuesday 4 March, 3:30 pm: Network Theory II: stochastic Petri nets, chemical reaction networks and Feynman diagrams. Also available on YouTube.
• Tuesday 11 March, 3:30 pm: Network Theory III: Bayesian networks, information and entropy. Also available on YouTube.
I thank Samson Abramsky, Bob Coecke and Jamie Vicary of the Computer Science Department for inviting me, and Ulrike Tillmann and Minhyong Kim of the Mathematical Institute for helping me get set up. I also thank all the people who helped do the work I’ll be talking about, most notably Jacob Biamonte, Jason Erbele, Brendan Fong, Tobias Fritz, Tom Leinster, Tu Pham, and Franciscus Rebro.
Ulrike Tillmann has also kindly invited me to give a topology seminar:
Operads and the Tree of Life
Trees are not just combinatorial structures: they are also biological structures, both in the obvious way but also in the study of evolution. Starting from DNA samples from living species, biologists use increasingly sophisticated mathematical techniques to reconstruct the most likely “phylogenetic tree” describing how these species evolved from earlier ones. In their work on this subject, they have encountered an interesting example of an operad, which is obtained by applying a variant of the Boardmann–Vogt “W construction” to the operad for commutative monoids. The operations in this operad are labelled trees of a certain sort, and it plays a universal role in the study of stochastic processes that involve branching. It also shows up in tropical algebra. This talk is based on work in progress with Nina Otter.
I’m not sure exactly where this will take place, but probably somewhere in the Mathematical Institute, shown on this map. Here’s the time:
• Monday 24 February, 3:30 pm, Operads and the Tree of Life.
If you’re nearby, I hope you can come to some of these talks — and say hi!
(This diagram was drawn by Darwin.)


Re: Network Theory Talks at Oxford
All these great things have been happening at Oxford, and I keep missing them! First, the grand opening of the new site, and now this. And I’m extra sorry now that I’ve had a sneak preview of your and Tobias’s new paper :-)