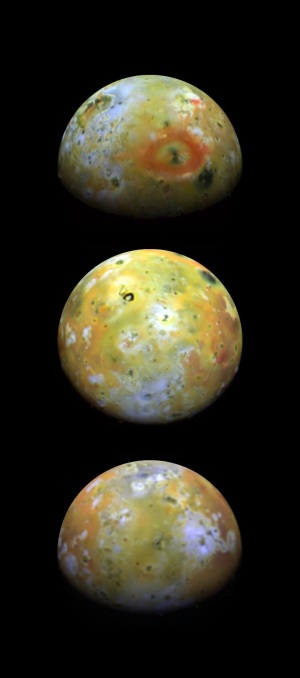
In week269 of This Week’s Finds, see more of Jupiter’s moon Io:
Then learn about honeycombs, the work of Kelvin, the Weaire-Phelan structure, and gas clathrates.
Posted at August 31, 2008 4:31 AM UTCTrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/1779
What’s the problem? You don’t see the geysers? First look at the bluish-white plume above the top horizon of Io. It may look small but it’s 140 kilometers high.
The other one is a bit harder to see because we’re looking straight down at it, but it’s the bluish-white blob right in the middle of the picture:
Maybe I should use a photo that’s easier to understand. I was planning to save the really dramatic ones for later.
The Galileo spacecraft was launched in 1989 and arrived in the Jovian system in 1995.
Thank you, Blake Stacey.
In another thread I mentioned my ground software for Galileo that was entirely designed with old fashioned matrices and Euler angles (over 200 pages of equations), to avoid repeating any mistakes that may have crept into the Flight Software, which used quaternions, because that was more compressed in memory.
NASA is not run by Mathematical Physicists, but there have been a lot of us drawing the paychecks to do really cool stuff.
John wrote:
Here’s a picture of two such geysers, taken by the Galileo spacecraft in 1979 […]
Blake wrote:
The Galileo spacecraft was launched in 1989 and arrived in the Jovian system in 1995.
Whoops! The picture was taken in 1997, but the article about this picture said the Prometheus plume has been visible ever since the Voyager flyby in 1979. I got my digits twisted.
Thanks — I fixed it.
In email, John McKay mentions — with some skepticism — an old theory that the ‘Bermuda Triangle events’ were caused by ocean-floor methyl hydrates being released as gas.
Unsurprisingly, one can find more discussions of this idea online, both approving and skeptical.
But, regardless of this, John’s comments have helped improve week269: it seems there are methane hydrates on continental shelves all over, not just in northern oceans as I’d claimed. It’s interesting how the high pressures make these ice-like clathrate structures stable at temperatures up to 18° C!
It’s also pleasant that methane hydrates are among the clathrates that favor the Weaire–Phelan structure — a mix of 12-sided and 14-sided polyhedra.
In email, Gavin Wraith mentions a lecture by J. D. Bernal that Wraith attended as an undergraduate in Cambridge in the late 1950s:
He demonstrated an experiment he had devised to find the average number of flat surfaces one may expect to find on a polyhedron in a space-filling packing. He put roundish lumps of clay in a bag and jiggled the bag about so that they impinged on each other, creating flat faces where they did so. He repeated this process with varying numbers of lumps and counted the number of flat faces obtained over many experiments. I seem to remember that the average turned out to be a little over 13; I forget the precise initial digits. Well, his answer did lie between 12 and 14!
Of course the implicit question is: how is this random packing problem related to the Weaire–Phelan structure?
I’m reminded of another experiment I’d read about. Some old bigshot took a bunch of nice round peas, squashed them down, and then studied the polyhedra they formed.
My attempts to learn about this experiment have been frustrated so far: Google Books suggests Soft and Fragile Matter, a book published by the evil CRC Press, but the relevant page won’t load for me. So, all I can see is a sentence beginning: “In one set of experiments he filled a container with English peas, filled the remaining volume with water and then drained the water and measured its volume…”
That sounds like a slightly different experiment than the one I’m (mis?)remembering.
The bigshot was Stephen Hales (1677–1761), an early plant physiologist whose work on sap pressure led him to investigate blood pressure in animals. Matzke (1950) cites Hales’ Vegetable Staticks (1727) as saying,
I compressed several fresh parcels of Pease in the same Pot, with a force equal to 1600, 800 and 400 pounds; in which Experiments, tho’ the Pease dilated, yet they did not raise the lever, because what they increased in bulk was, by the great incumbent weight, pressed into the interstices of the Pease, which they adequately filled up, being thereby formed into pretty regular Dodecahedrons.
Hales was probably exaggerating.
The experiment became known as the “peas of Buffon” (because the Comte de Buffon later wrote about a similar experiment), and most biologists accepted it without question until Edwin B. Matzke, a biologist at Columbia University, repeated the experiment. Because of the irregular sizes and shapes of peas, their nonuniform consistency and the random packing that results when peas are poured into a container, the shapes of peas after compression are too random to be identifiable.
Thanks! Great! I think the ‘bigshot’ was Buffon.
One funny thing about Hales’ claim is that you can’t fill space with regular dodecahedra.
If he meant rhombic dodecahedra, that would be more interesting, because you can fill space with those.
D’Arcy Thompson’s On Growth and Form has some interesting things to say:
If we squeeze a mass of clay pellets together, like Buffon’s peas, they come out, or all the inner ones do, in neat garnet-shape, or rhombic dodecahedra. But a young student once showed me (in Yale) that if you wet these clay pellets thoroughly, so that they slide easily on one another and so acquire a sort of pseudo-fluidity in the mass, they no longer come out as regular dodecahedra, but with square and hexagonal facets recognizable as those of ill-formed or half-formed tetrakaidekahedra.
I think he’s referring here to the 14-sided figure with square and hexagonal faces usually called the ‘truncated octahedron’:
Later he writes:
A somewhat similar result, and a curious one, was found by Mr. J. W. Marvin, who compressed leaden small-shot in a steel cylinder, as Buffon compressed his peas; but this time the pressure on the plunger ran from 1000 to 35,000 lb, or nearly twenty tons to the square inch. When the shot was introduced carefully, so as to lie in ordinary close packing, the result was an assemblage of regular rhombic dodecahedra, as might be expected and as Buffon had found. But the result was very different when the shot was poured at random into the cylinder, for the average number of facets on each grain now varied with the pressure, from about 8.5 at 1000 lb to 12.9 at 10,000 lb, and to no less than 14–16 after all interstices were eliminated, which took the full pressure of 35,000 lb to do. An average of just over fourteen facets might seem to indicate a tendency to the production of tetrakaidekahedra, just as in the froth of soap-bubbles; but this is not so. The squeezed grains are irregular in shape, and pentagonal facets are much the commonest…
And much more. The editor of this edition corrects Thompson by pointing out that ‘Buffon’s peas’ are actually due to Hale.
I found that if you click on one of the page arrows, it loads up. The quote you want is on page 319; they found that peas didn’t work for them, but Israeli couscous did, and that when they added ink, it was pressed away from where the faces met and dyed the edges of the couscous, making it very easy to count faces.
I found another quote by Kelvin that I added to the ‘quote of the week’ for week269. It’s especially poignant when taken in combination with the one I already had there. So, here are both of them:
My suggestion is that Aepinus’ fluid consists of exceedingly minute equal and similar atoms, which I call electrions, much smaller than the atoms of ponderable matter. — from his paper Aepinus Atomized
One word characterizes the most strenuous effors for the advancement of science I have made perserveringly during fifty-five years; that word is Failure. I know no more of electric or magnetic force, or of the relation between ether, electricity and ponderable matter, or of chemical affinity, than I knew and tried to teach to my students of natural philosophy fifty years ago in my first session as professor. — from a speech given at a celebration of his life’s work attended by over 2000 guests
Re: This Week’s Finds in Mathematical Physics (Week 269)
John Baez wrote,
?