The n-Category Café

Let

be a space and let

be vector bundle over $X$ with connection

Equivalently this means # that we have a locally smoothly trivializable functor

that sends paths in $X$ to the parallel transport along them obtained from the connection $\nabla$.

Quantizing the single particle charged under this bundle with connection consists of a kinematical and of a dynamical aspect:

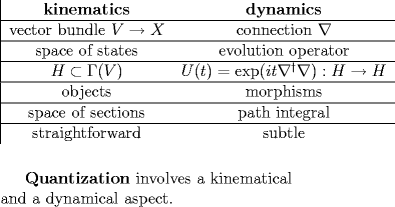
In this first part of the story we amuse ourselves by just doing the trivial kinematics – but in a nice way.

So, let’s forget the connection immediatey by just looking at constant paths in $X$. I’ll write

for the above functor restricted to constant paths, ie. to the discrete category over $X$. It does nothing but sending each point in $X$ to the vector space sitting over it – but smoothly so.

We can play the same game on the base space

that consists of nothing but a single point.

A trivial rank one vector bundle over a point is a functor

that does nothing but sending the single point to the complex numbers:

I admit that I am presupposing a certain tolerance for fancy-looking trivialities here. But enduring these will pay off eventually.

Using the uniqe functor from $X$ to the point

we can pull back the trivial vector bundle over the point to $X$. The result

is the trivial rank one bundle on $X$. This functor simply sends each point of $x$ the typical fiber $\mathbb{C}$:

In as far as any of this is interesting at all, it is for the following simple fact:

a morphism of functors:

is precisely a section of the vector bundle $V$: $e$ is nothing but an assignment

of a linear map from $\mathbb{C}$ to the fiber $V_x$ for each point $x$. That’s nothing but a choice of vector in each fiber.

So, the space of all such functor morphisms

from the trivial one into the one defining our vector bundle is nothing but the space of sections of $V$.

Since $\Gamma(V)$ is a vector space, and since vector bundles over the point are nothing but vector spaces, I want to think of $\Gamma(V)$ as a vector bundle over the point. So I regard it as a functor

On top of all these trivialities, I’ll finally allow mysef to think of $\Gamma(V)$ as morphisms from the trivial line bundle on the point into this guy:

The upshot is that, taken together, we get the isomorphism

If you like, you can convince yourself that this isomorphism of Hom-spaces in indeed natural in both arguments. But this means that pulling back functors from points to $X$

is the adjoint of taking sections

This, in turn, says that forming the space of sections of $\mathrm{tra}_V$ is the result of pushing $\mathrm{tra}_V$ forward to a point.

Of course that’s neither new nor very deep. But part of a nice story that still needs to be told.