
Above are the roots of some polynomials with 8 coefficients of 1 and 9 of -1:

Above are the roots of some polynomials with 9 coefficients of 1 and 8 of -1:

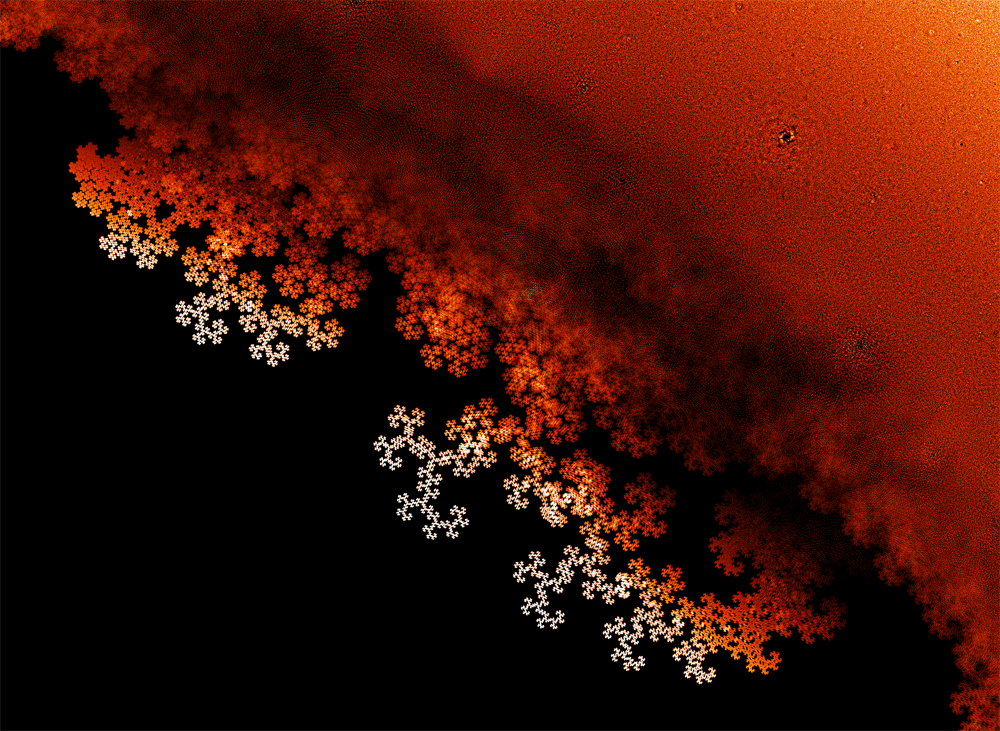
Above are the roots of some polynomials with 10 coefficients of 1 and 7 of -1:
The general effect seems to be that as you shift the coefficient that gets the plus sign you can move the roots around various curves, but I can’t see any simple formula for what’s going on here.

















Re: This Week’s Finds in Mathematical Physics (Week 285)
Wow! Exquisite as those pictures by Christensen and Derbyshire are, what really gets me is that this is such an obvious thing to do, yet apparently no one had thought to do it before. (Or had they?)
It reminds me of something I read about the resurgence of interest in complex dynamics, in the late 1970s. (If I understand correctly, the field had been largely dormant since about 1920, when Fatou and Julia did their pioneering work.) A bunch of people, including Brooks, Matelski, Douady, Hubbard and Mandelbrot, began to study the iteration of quadratic maps, with the aid of the emerging computer technology.
Douady was working in Paris, close to the IHES. It must have been an amazing place and time. Deligne had solved the Weil conjectures not so long ago. And Douady… Douady was studying quadratic equations. There’s some interview with or about him, which I can’t find now, where he recalls someone asking “These quadratics — do you really hope to find anything new?”
And while I’m recalling anecdotes, I can’t resist adding this one, from Milnor’s book Dynamics in One Complex Variable.