8 | The n-Category Café

September 7, 2008

8

Posted by John Baez

$MathML-enabled post (click for more details).$

Next Saturday I’m going to the University of Glasgow to give talks about some of my favorite numbers: 5, 8 and 24. Different numbers have different personalities, as I try to explain here:

Damien Henderson, Do numbers have personalities? You can count on it, The Herald, Sept. 6, 2008.

If you can’t attend, you can still pretend. So far I’m only satisfied with my talk on the number 8 — click on the title below to see the transparencies.

$MathML-enabled post (click for more details).$

$8$

Abstract: The number 8 plays a special role in mathematics due to the “octonions”, an 8-dimensional number system where one can add, multiply, subtract and divide, but where the commutative and associative laws for multiplication — $a b = b a$ and $(a b)c = a(b c)$ — fail to hold. The octonions were discovered by Hamilton’s friend John Graves in 1843 after Hamilton told him about the “quaternions”. While much neglected, they stand at the crossroads of many interesting branches of mathematics and physics. For example, superstring theory works in $10$ dimensions because $10 = 8+2$ : the 2-dimensional worldsheet of a string has $8$ extra dimensions in which to wiggle around, and the theory crucially uses the fact that these $8$ dimensions can be identified with the octonions. Or: the densest known packing of spheres in $8$ dimensions arises when the spheres are centered at certain “integer octonions”, which form the root lattice of the exceptional Lie group $E_8$ . The octonions also explain the curious way in which topology in dimension $n$ resembles topology in dimension $n+8$ .

If you can attend, I hope you do! I think Eugenia Cheng, Simon Willerton and Danny Stevenson may be there… and surely Tom Leinster will, since he’s organizing the show. Details on the schedule can be found here.

By the way, I don’t like how the newspaper suggests I said schoolchildren have a “limited” understanding of mathematics. Did I really say that? Of course it’s true in some sense, but it misses the point in a mean-spirited way. It’s a bit like calling a baby “a short, weak kid who cries a lot and never talks”.

Note added later: you can now see a streaming video of this talk.

Posted at September 7, 2008 12:53 AM UTC

TrackBack URL for this Entry: https://golem.ph.utexas.edu/cgi-bin/MT-3.0/dxy-tb.fcgi/1783

77 Comments & 0 Trackbacks

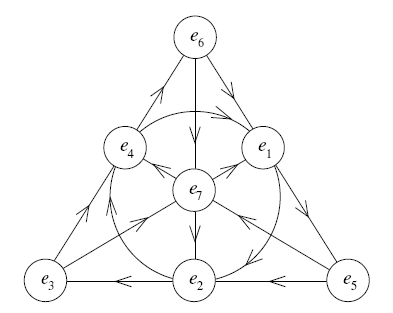
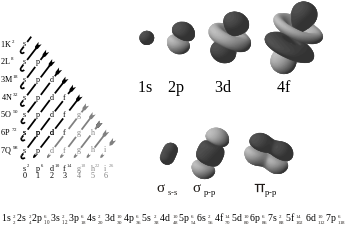
Re: 8

It looks like there is a typo in the slides, page 9, i^2 = j^2 = k^2 = ijk = 1. Probably this should be -1, as is stated later on page 11.

Posted by: Ian on September 7, 2008 8:24 AM | Permalink | Reply to this

Re: 8

Whoops! An embarrassing typo — thanks for catching it.

Posted by: John Baez on September 7, 2008 9:08 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

slide 14: “they octonions”

slide 20: not that it matters, but just as a remark: those oscillations “perpendicular to this surface [the string’s worldsheet]” are technically usually called “transversal” oscillations.

slide 21: not that it matters, but just as a remark: in which dimensions one can have “consistent” superstrings depends a bit on what counts as “consistent”. The more precise – but of course less generally understandable statement – would be that the “critical” superstring on a geometric target space requires to have 2+8 dimensions. A direct and concrete but maybe little insightful reason for this is that direct computation shows that only in this case is the quantization of the string’s BRST operator, which encodes the action Lie algebroid of the conformal group acting on the string’s configuration space, still nilpotent in that various corrections from re-ordering of operators which could spoil this cancel each other.

As we recently mentioned elsewhere, the assumption “geometric background” here is crucial. What is really required in critical superstring theory is that the worldsheet SCFT has central charge 15. For geometric backgrounds (SCFTs coming from sigma-models) this is the case in 10 dimensions. But generally there is no reason why the world we observe has to be a purturbation about a geometric background. For other backgrounds the usual statement that “superstring theory predicts spacetime to be 10-dimensional” is at best imprecise, since the notion of dimension is ambiguous in these cases.

Nothing of this matters for your talk, of course. I just felt like mentioning it.

Posted by: Urs Schreiber on September 7, 2008 2:30 PM | Permalink | Reply to this

Re: 8

Thanks for the corrections. Since the audience will be very broad and I won’t have much time to cover all this material, I’ll probably only fix ‘they octonions’.

My discussion of ‘perpendicular to the worldsheet’ will involve making one hand look like a string worldsheet, wiggling it, and using the index finger of my other hand to indicate the perpendicular direction. (I’ve got it thoroughly practiced.)

On a different note: could you explain what’s better about ‘critical’ strings than ‘noncritical’ ones, and why string theorists seem to focus only on the critical ones?

And: what’s magic about the number 15?

I will restrict myself to geometric backgrounds, since my audience will probably be background-free (in the nontechnical sense).

Posted by: John Baez on September 7, 2008 9:30 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

could you explain what’s better about ‘critical’ strings than ‘noncritical’ ones, and why string theorists seem to focus only on the critical ones?

You know this maybe not under the term “critical string”, but it’s the familiar idea:

The worldsheet theory of the (super)string is really a 2-dimensional QFT of (super)gravity. Hence in particular the action functional and hence the classical theory are invariant under worldsheet diffeomorphisms.

Since gravity in 2 dimensions is almost but not quite, entirely dull it is usually quantized by first fixing pretty much all the diffeomorphism gauge symmetry and then quantizing. The little gauge invariance which remains unfixed is the conformal group. After quantization, the invariance under this conformal group remains unbroken if and only if the string is “critical”.

In your slides you mention the “reason” for the critical string to have a target space of dimension 2+8. There are maybe two ways to understand “reason” here:

a) the frist, something like “pure logical necessity” comes from direct computation: compute the quantum anomaly of the gauge invariance and check in which cases it vanishes. Direct computation shows that it vanishes in the “critical dimension”, 10 for the superstring, 26 for the bosonic version.

b) the other, which is the one you are really after, is the “moral reason”, that which gives us an impression of deeper understanding. You want an intuitive, heuristic way to understand why the string is special on a 2+8-dimensional target.

Concerning that kind of “reason” I can’t quite provide much more than what you already mention: one observes that triality of the transversal SO(8) plays a crucial role for the supersymmetry of the superstring and that is related to all things octonionic in one way or another.

This also serves, then, as a “reason” for the 11-dimensions of the target space for the super-membrane (the 3-particle), since its worldvolume is 3-dimensional and 11= 3 + 8.

what’s magic about the number 15?

In the sense of a): 15 just happens to drop out of the computation.

In the sense of b): the only magic I see is the one you already discuss: the 15 here is really 10 times (1+1/2): every worldsheet boson (= every diemsnion of target space) contributes 1 to the central charge, every worldsheet fermion contributes 1/2. Hence for a supersymmetric worldsheet theory with one fermion per boson the central charge is $\frac{3}{2} d$ , with $d$ the dimension of target space.

Hence $15 = \frac{3}{2}(2 + 8)$ and we are back at the number 8, where we started.

since my audience will probably be background-free

So you will give a background-independent talk, which is what really matters. :-)

Posted by: Urs Schreiber on September 8, 2008 6:11 PM | Permalink | Reply to this

Why We Love Numbers; Re: 8

“Do numbers have personalities?”

Yes, they do.

Imagine a hotlink here to the Ramanujan taxicab number anecdote. Another to Pythagorus. One to Feynman and Nabokov on the colors of numerals and variables.

The Herald did a reasonable job, given that the typical newspaper reporter is MORE “limited” than a child, in no longer asking good questions about numbers.

Philosophically interesting to me: why do so many people have a response to “what is your favorite number?” And what is the distribution of the answers? I have many friends who answer “i” or “pi” or the like.

Theomathematically, if we are finite beings, we can perceive personalities in finite sets of integers. A transfinite being can perceive personality in infinite sets of real numbers.

I’ve read no science fiction of beings who actually think in Category Theory or n-Category Theory. If I were John Baez, I’d outsource that to Greg Egan.

I just tried, by the way, to explain how much I liked your summary of why it matters to superstring theory that the universe has 10 dimensions because 10 = 8 + 2. Because she’s an experimental Physicist, she gave me the kind of look that only wives of crazy husbands can give.

Posted by: Jonathan Vos Post on September 7, 2008 6:30 PM | Permalink | Reply to this

Re: Why We Love Numbers; Re: 8

$MathML-enabled post (click for more details).$

Jonathan wrote:

I’ve read no science fiction of beings who actually think in Category Theory or n-Category Theory. If I were John Baez, I’d outsource that to Greg Egan.

Since I actually think in category theory, I have no huge desire to read SF about other beings who do that. But it might be fun for other people.

And Greg could surely do the job as well as anyone. I just got a copy of Incandescence, so I got to read the rest of the section quoted by Joceyln Paine. It continues as follows:

Paba offered them a description of the work that the friends were pursuing. Rakesh absorbed only the first-level summary, but even that was enough to make him giddy. Starting with foundations in the solid ground of number theory and topology, a glorious edifice of generalisations and ever-broader theorems ascended, swirling into the stratosphere. High up, far beyond Rakesh’s own habitual understanding, no less than five compelling new structures that the trio had identified had started to reveal interesting echoes of each other, as if they were, secretly, variations on a single theme.

[…]

“How long have you been searching for something like this?” Rakesh asked.

“Thirteen hundred years,” Paba replied. Rakesh glanced at her precís; that was most of her life. “Not full-time,” she added. “Over the years, for one or two days in every ten or twenty as the mood has struck us.”

Ah, to have that sort of time! Past a certain point it’ll be necessary to live that long to get a really intuitive understanding of a reasonable hunk of the math that’s been developed… unless of course we speed up our thoughts (which these characters don’t seem to be doing, here). We could already use a couple of centuries.

Posted by: John Baez on September 7, 2008 9:59 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

You can also read the Herald article here. This is a photo of the actual newspaper article (high-resolution enough to read), and unlike the online version, contains a photo of John and a whimsical inset on why “Five is magic”.

Posted by: Tom Leinster on September 7, 2008 10:32 PM | Permalink | Reply to this

Re: 8

Thanks!

It’s actually a very nice article, all things considered. How often do you see a newspaper article saying things like this?

Maths has a longer pedigree as one of the purest branches of knowledge which has provoked a passionate following among its advocates.

Plato, who believed mathematical relationships to be truer than perceived reality, had the message “let no-one ignorant of geometry enter” inscribed on the entrance to his academy, while his predecessor, Pythagoras, pursued the subject with a religious fervour.

Maybe you get such stuff all the time up in Glasgow. But in the L A Times, our biggest dose of classical culture comes in articles about Xena: Warrior Princess.

Posted by: John Baez on September 7, 2008 11:26 PM | Permalink | Reply to this

Re: 8

Ah, that gem “Let no one enter…”! I wonder what the latest is on this. Here’s a comment by Ed Johnston on the MATH-HISTORY-LIST from 1999.

David H. Fowler discusses in details the inscription in the section 6.1 of his monumental _The Mathematics of Plato’s Academy_ 2nd ed., OUP 1999, pp. 199-204…

Quoting from the Oxford Classics Dictionary, Tzetzes was “a 12th century copious, careless, quarrelsome Byzantine polymath ….. who nevertheless preserves a few fragments of ancient poetry and offers an engaging glimpse of the life of a Byzantine scholar in a period of intense interest in ancient Greek literature.”

Translation:

On the front of his doorway Plato had written
‘Let no one who is not a geometer enter my house.’
That is, ‘Let no one who is unjust come in here’,
for geometry is equality and justice.

But Fowler himself does not seem to support this *late* interpretation; he thinks that it might have been “introduced” by the 4th century A.D. orator Sopatros, while my own excursion through the TLG [Thesaurus Lingua Graeca] did not allow me to trace it any earlier than the Byzantine philosopher Arethas (9th - 10th century).

Another interpretation, traced (TLG) back to “pseudo-Galenos” (some time (?) after 2nd century A.D.), and several 6th+ century scholiasts of Aristotle, suggests that geometry was viewed by Plato et al as a *prerequisite* for philosophy and theology, hence the quote at the entrance of Plato’s Academy. But the apparent lack of any reference to the quote for 5+ centuries casts doubts on its authenticity. It is even possible that the inscription was inscribed by neo-Platonists, any time between “pseudo-Galenos” and the Academy’s forced closing in 529 A.D..

The one thing that is certain is that “ageometritos” does not appear anywhere in Plato’s work, although it does appear in Aristotle, under the double meaning “ignorant of geometry” and “independent of geometry”. A few centuries later, historian Strabo uses “ageometritos” under the meaning “not having a well known geometrical shape”; and after him there are no recorded users prior to the various scholiasts already mentioned above. Contemporary Greeks would rarely, if ever, use “ageometritos” = “ignorant of geometry”, but they use “ageographitos” = “ignorant of geography” all the time :-)

Posted by: David Corfield on September 8, 2008 9:18 AM | Permalink | Reply to this

Re: 8

Ok the newspaper article has me excited now.

Posted by: Bruce Bartlett on September 7, 2008 11:29 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Are you actually gonna be there, Bruce??

Posted by: John Baez on September 8, 2008 5:18 PM | Permalink | Reply to this

Re: 8

Hi John, yes I aim to be there though I’m not sure if I will be able to make the whole week.

Posted by: Bruce Bartlett on September 9, 2008 5:50 PM | Permalink | Reply to this

Re: 8

On the page next after the bridge:

ki = j = -ki

Posted by: Georgiy on September 8, 2008 7:08 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Aargh!

Fixed. Thanks very much!

Posted by: John Baez on September 8, 2008 5:26 PM | Permalink | Reply to this

Easy As 1, 2, 3; Re: 8

As Easy As 1, 2, 3: Number Sense Correlates With Test Scores

ScienceDaily (Sep. 8, 2008) — Knowing how precisely a high school freshman can estimate the number of objects in a group gives you a good idea how well he has done in math as far back as kindergarten, researchers at The Johns Hopkins University found.

Good “number sense” at age 14 correlates with higher scores on standardized math tests throughout a child’s life up to that point and weaker “number sense” at 14 predicts lower scores on those standardized tests, said Justin Halberda, assistant professor of psychological and brain sciences in the university’s Krieger School of Arts and Sciences.

“We discovered that a child’s ability to quickly estimate how many things are in a group significantly correlates with that child’s performance in school math for every single year, reaching all the way back to when he or she was in kindergarten,” Halberda said.
[truncated]

Posted by: Jonathan Vos Post on September 8, 2008 3:01 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Thanks to everyone for catching some egregious typos!

I’ve added a second appendix, after the table of densities of sphere packings in dimensions $\le 8$ . This describes how the number 5 and the number 8 learn to play together. Namely, how to start from the symmetry group of the dodecahedron and get the $E_8$ lattice. Right now, at least, I don’t describe the most famous way to do this — the McKay correspondence. Instead, I describe a much more elementary recipe from Sphere Packings, Lattices and Groups.

Posted by: John Baez on September 8, 2008 5:23 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Our press office has clearly got very excited about this. By the time John arrives, he’ll be so famous in Glasgow, he’ll have to wear dark glasses. I expect him to arrive at the airport looking like the Beckhams.

Posted by: Tom Leinster on September 9, 2008 12:51 PM | Permalink | Reply to this

Re: 8

Both of ‘em? That’ll be tough.

I do, however, expect to see a crowd of people supporting my presidential campaign.

Posted by: John Baez on September 9, 2008 4:18 PM | Permalink | Reply to this

Re: 8

247 is just dull.

Much maligned number! Didn’t you know that the 247th hexagonal number is equal to the 221st heptagonal number? Or that if you repeated raise the digits of a number to the power five and add, then if you start with 247, you end up with a sequence of period 1?

Posted by: David Corfield on September 9, 2008 1:59 PM | Permalink | Reply to this

Re: 8

Sorry — you should never say a number is uninteresting when David Ramanujan Corfield is in the room.

But frankly: if that’s the best you can do, I think you’ve just proved my point.

Posted by: John Baez on September 9, 2008 4:08 PM | Permalink | Reply to this

Re: 8

You win. Perhaps it’s the smallest uninteresting number.

Posted by: David Corfield on September 9, 2008 4:45 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

I’m afraid it doesn’t even have that distinction.

I’ll grant you this: 247 is the first rank-2 Switzenburg number. In other words, it’s the $n$ th uninteresting number for a value of $n$ that is itself uninteresting — and it’s the smallest number with this property. However, that isn’t enough to make it interesting, since the whole subject of Switzenburg numbers is incredibly dull. I took a course on them in grad school and the whole class, including the instructor, fell asleep ten minutes after the beginning of each lecture. In fact I’m getting sleepy just thinking about this.

Posted by: John Baez on September 12, 2008 2:37 AM | Permalink | Reply to this

Re: 8

Can I use your “8” presentation in a thread at physicsforums? (Perfect Symmetry)
jal

Posted by: jal on September 10, 2008 2:13 AM | Permalink | Reply to this

Re: 8

Sure, go ahead and use it!

Posted by: John Baez on September 10, 2008 5:40 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

I am travelling at the moment and feel like I should check some textbook references and recall some computations for details of the following, but if I am allowed to make a quick rough comment, here goes:

I am worried that the statement “superstrings exist in dimensions $d=3,4,6,10$ ” on p. 20 of your slides on “8” is a bit misleading,

I think the statement is really that 3,4,6,10 are the dimensions in which there are super-Yang-Mills theories.

Now, all of these super-Yang-Mills theories are in some way or other connected to superstrings, but only one of them in the direct way that you seem to indicate:

SYM in d=10 is part of the effective background theory of heterotic strings, yes

SYM in d=6 is part of “little string theories”, namely of the worldvolume theories of NS 5-branes – this is a highly interesting but also very little understood case: Witten used it to explain S-duality and now Langlands duality and said back then that there should be nonabelian gerbes on these 5-branes

SYM in d=4 is the CFT dual theory to heterotic strings on asymptotic AdS backgrounds

In general, I think you could say that all things related to division algebras are closely related directly to supersymmetric QFTs. The relation to superstrings is really in a sense via this intermediate step.

I think it all comes down to the following fact in ordinary differential geometry, which is discussed for instance nicely in old lecture notes by Jürg Fröhlich.

for a manifold to admit “1+1” supersmmetric particles it has to be Riemannian: then the supercharges are the deRham operator $d$ and its Hodge adjoint (yielding an “ $N=(1,1)$ spectral triple”)

for a manifold to admit “2+2” supersymmetric particles it has to be Kaehler: then the supercharges are the Dolbeault-deRham operator $\del$ , $\bar \del$ and their Hode adjoints (yielding an “ $N=(2,2)$ spectral triple”)

for a manifold to admit “4+4” supersymmetric particles it has to be hyper Kaehler: then the supercharges are the three copies of the Dolbeault operators $\del_i$ , $\bar \del_i$ and their Hodge adjoints (yielding an “ $N=(4,4)$ spectral triple”)

finally, a manifold can somehow be “8+8” supersmmetric. I forget how precisely this is formulated, but it corresponds to continuing the above pattern over real numbers, complex numbers and quaternions to octonions somehow.

The basic idea behind this relation of supersymmetry and division algebra is easy to prove, I think I recalled that somewhere in my masters thesis, but this very minute I seem to not quite recall the details.

The point is to start with a Dirac operator and then assume that it has various higher odd symmetries, i.e. that there are a bunch of odd operators all anticommuting with each other and with the Dirac operator. With these in hand, we can split the Dirac operator into parts. When one works this out for the simple case of a Dirac operator on flat space, one easily finds the pattern I just mentioned.

Posted by: Urs Schreiber on September 10, 2008 10:55 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Urs wrote:

I am worried that the statement “superstrings exist in dimensions d=3,4,6,10” on p. 20 of your slides on “8” is a bit misleading.

I think the statement is really that 3,4,6,10 are the dimensions in which there are super-Yang-Mills theories.

That’s true too — and it must be related. But I was referring to this statement from section 5.1.2 of Green, Schwarz and Witten:

Thus $S_2$ [the superstring action] is supersymmetric only in the following four cases:

$D = 3$ and $\theta$ is Majorana;

$D = 4$ and $\theta$ is Majorana or Weyl;

$D = 6$ and $\theta$ is Weyl;

$D = 10$ and $\theta$ is Majorana–Weyl.

Thus (in this formalism) the classical superstring theory exists only in these four cases. This is the counterpart of the statement that the classical bosonic string theory exists for any dimension. This result should not be a surprise, since supersymmetry is well-known to restrict the possible values of $D$ even at the classical level. Quantum considerations will single out the $D = 10$ case as special, of course.

So, I think my statement was okay. I wish I understood this all much better, though. In particular, I don’t know if there is any link between the $D = 4$ and $D = 6$ supersymmetric theories that you mention and the classical superstring Lagrangians in these dimensions. Quite possibly not, except for the fact that these are the dimensions where supersymmetry works nicely!

Finally, a manifold can somehow be “8+8” supersymmetric. I forget how precisely this is formulated, but it corresponds to continuing the above pattern over real numbers, complex numbers and quaternions to octonions somehow.

This is surely the most exciting case, no? I would like to learn more about this case. In week195 I described a concept of ‘ $X$ -manifold’ which includes spin manifolds, Kähler manifolds, hyperKähler manifolds and certain 8-dimensional manifolds I call ‘octonionic manifolds’ as special cases. However, I don’t think those are what you’re talking about.

The basic idea behind this relation of supersymmetry and division algebras is easy to prove, I think I recalled that somewhere in my masters thesis, but this very minute I seem to not quite recall the details.

As you know, my talk focuses on this piece of the puzzle: when $Spin(n)$ has a spinor representation that’s the same dimension as its vector representation, the map

$[vectors] \otimes [spinors] \to [spinors]$

gives a division algebra. I described this more precisely here. (There I carefully distinguish between different kinds of spinors — something I’m glossing over here).

Underlying this idea there’s an even more basic one, described here: whenever we have an $n$ -dimensional normed division algebra, we get a representation of $Cliff(n-1)$ on $\mathbb{R}^n$ .

But you may be thinking about different pieces of the puzzle, more related to the existence of supersymmetry transformations. It’s a fascinating business!

Posted by: John Baez on September 10, 2008 6:04 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

the classical superstring theory exists only in these four cases

Ah, I see, that’s what you are thinking of. I would still think it is somehow better to speak then just of (supergravity coupled to) super-Yang-Mills Lagrangians on the target (some of which may arise as effective background field theories of quantum superstrings) but of course when GSW use the term in your sense, who am I to stop you ;-)

Posted by: Urs Schreiber on September 10, 2008 8:35 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

John wrote:

If you can’t attend, you can still pretend.

Better yet, you can watch the videos. We’ll be filming all John’s talks, and if all goes according to plan, they’ll be available via the Rankin Lectures web page before too long.

Posted by: Tom Leinster on September 10, 2008 9:06 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

This is great fun (as is the one on 24)!

I think there’s a minor formatting error in slide 42: three $\mathbb{R}$ s that should be Rs, seeing as they’re representations, not the real numbers.

Posted by: Greg Egan on September 12, 2008 4:06 AM | Permalink | Reply to this

Re: 8

Thanks! I’ll fix that mistake. And then it’s time for bed, where I get to read Incandescence.

Posted by: John Baez on September 12, 2008 6:54 AM | Permalink | Reply to this

Re: 8

In slide 16, the Fano Plane representation only covers half of the Octonions, the Right Octonions. The other non-isomorphic half, the Left Octonions require the vertex bisecting arrows to all point in the opposite direction.

http://www.octospace.com/files/Octonion_Algebra_and_its_Connection_to_Physics.pdf

http://www.octospace.com/files/Why_octonions_2.pdf

Rick Lockyer

Posted by: Rick Lockyer on September 14, 2008 3:34 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

All 8-dimension normed division algebras are isomorphic to the usual octonions, so people don’t usually talk about ‘left’ and ‘right’ octonions. I guess you’re talking about two nonisomorphic multiplication tables which give algebras isomorphic to the octonions?

There’s certainly room for this sort of thing, since there are some arbitrary-looking choices in orienting the lines of the Fano plane to get an octonion multiplication table:

Geoffrey Dixon and Tony Smith have thought about this stuff. Tony Smith says there are 480 octonion multiplication tables, which may be organized into two types, such that if $e_i e_j = e_k$ in some table of the first type, then $e_j e_i = e_k$ in some table of the second type.

There are 240 tables of each type.

Does anyone who attended my talk remember that number: 240? It’s the maximum number of equal-sized spheres you can get to kiss a given sphere of that size in 8 dimensions! In other words: the number of roots in the $E_8$ lattice!

Geoffrey Dixon goes further here:

Geoffrey Dixon, Octonion X-product and E8 lattices.

Briefly, this goes as follows. I just showed you a multiplication table for the “seven dwarves” — a basis of the imaginary octonions — but there are lots of other multiplication tables that would also give an algebra isomorphic to the octonions. Given any unit octonion a, we can define an “octonion $\times$ -product” as follows:

b $\times$ c = (b a)(a* c)

where a* is the conjugate of a (as defined in “week59”) and the product on the right-hand side is the usual octonion product, parenthesized because it ain’t associative. For exactly 480 choices of the unit octonion a, the $\times$ -product gives us a new multiplication table for the seven dwarves, such that we get an algebra isomorphic to the octonions again! 240 of these choices have all rational coordinates (in terms of the seven dwarves), and these are precisely the 240 closest neighbors of the origin in a copy of the E₈ lattice! The other 240 have all irrational coordinates, and these are the closest neighbors to the origin of a different copy of the E₈ lattice. (Here we’ve rescaled the E₈ lattice so the nearest neighbors have distance 1 from the origin.)

By the way, if we combine this stuff with ideas explained in the appendix of my talk, we get a beautiful series of relationships between:

the 60 symmetries of the dodecahedron (i.e., the 60 elements of $A_5$ )
the 120 faces of the 120-cell (i.e., the 120 unit icosians)
the 240 spheres touching a central sphere in 8 dimensions (i.e., the 240 roots of $E_8$ )
the 480 octonion $\times$ -products

So, there are 8 octonion $\times$ -products per symmetry of the dodecahedron. I hadn’t noticed that until just now!

Posted by: John Baez on September 24, 2008 6:33 PM | Permalink | Reply to this

Re: 8

John,

I subscribe to the definition put forth in Kantor and Solodovnikov, “Hypercomplex Numbers, An Elementary Introduction to Algebras”:

“Definition 14.1 Two n-dimensional algebras are said to be isomorphic if they have bases with identical multiplication tables.”

Identical is perhaps better stated as equivalent, since row and column swaps make the multiplication tables look different without changing product rules. Since what I call Right and Left Octonion Algebras have non-equivalent multiplication tables, the algebras produced by each are not isomorphic algebras, but have all of the features typically given to Octonions.

In light of this, I think a statement of something being isomorphic to the Octonions begs an additional question of “which flavor of Octonions?”

This question can can be rendered moot by confining product forms to those that do not change sign when the basis product rules are changed up, what I call “Alebraic Invariants”. It just so happens that this is not at all restrictive, since interesting forms in physics expressed within the algebra of Octonions are all Algebraic Invariants.

As for there being 480 different multiplication tables, this can only happen if basis name aliasing is included, since there are only 2^7=128 possible ways to change up the 7 basis permutation product rules while avoiding aliasing, and only 16 of these are consistent with Artin’s Rule. 8 of 16 are Right Octonion and 8 are Left Octonion.

I caution anyone looking into algebra modification on the consistency with Artin’s Rule. It is not a given, even though you may have a reasonable mapping strategy.

Posted by: Rick Lockyer on September 28, 2008 1:28 AM | Permalink | Reply to this

Re: 8

Rick wrote:

I subscribe to the definition put forth in Kantor and Solodovnikov, “Hypercomplex Numbers, An Elementary Introduction to Algebras”:

“Definition 14.1 Two n-dimensional algebras are said to be isomorphic if they have bases with identical multiplication tables.”

Identical is perhaps better stated as equivalent, since row and column swaps make the multiplication tables look different without changing product rules. Since what I call Right and Left Octonion Algebras have non-equivalent multiplication tables, the algebras produced by each are not isomorphic algebras, but have all of the features typically given to Octonions.

Even though they have non-equivalent multiplication tables using certain bases, this does not rule out the possibility that they’re isomorphic in Kantor and Solodovnikov’s sense — which is, indeed, the usual sense. The reason is that you might be able to find some other bases for which the multiplication tables are identical.

I think that’s what’s going on. Note that in K & S’s definition you need the freedom, not just to permute the bases by ‘row and column swaps’, but to pick whatever basis you like.

It’s well-known that all 8-dimensional normed division algebras are isomorphic to the octonions. So, I’m willing to bet that your ‘left and right octonions’ are what I’d call different choices of multiplication table for the octonions.

Posted by: John Baez on September 28, 2008 10:53 PM | Permalink | Reply to this

Re: 8

One thing I am certain of is the concept Happy wife, happy life. So when the wife says I will not be touching a computer during our vacation to New England, I do not.

Left and Right Octonions do not have equivalent multiplication rules and hence can’t have equivalent multiplication tables, and therefore should not be isomorphic by K-S definition. There is no way to swap rows and columns of a Right Algebra to get a Left Algebra. However, any of the eight Right can be swapped up to be identical with any other Right, same for Left.

The method of modification of rule is not permute but negation. Not cyclic shift within a triplet but exchange of two members within selected triplets. As I said, this must all be done in a way that meets the requirements of Artin’s Rule, and this is as far as your freedom of fundamental basis choice can take you.

The operation of multiplication is the most distinguishing feature of an algebra. It makes little sense to me to consider isomorphic two algebras with distinctly different multiplication rules, even if they share other common features.

For quaternions, the different non scalar multiplication rule is that of a single permutation. Negation by swap of members here is an isomorphism only because the rule remains that of a single permutation, the fundamental definition of the algebra does not care what we call the basis members, or that after we have done so, we decide to swap two. Not so with octonions. Here we have seven permutations. The freedom to negate any or all gives us the ability to not only create multiple rule sets for octonions, but also to create algebras with rules that are no longer octonion by any stretch of the imagination. In fact, of the 128 binary operations, only 16 are actually octonions, and these 16 are 8 Right and 8 Left.

What is going on here is not basis choice by linear combination of fundamental basis components that are unchanged across all choices, what might be called a functional isomorphism. This is a fundamental definition of the algebra choice, which must be decided on and stuck to before any functional considerations.

Please look closer at this John. I think you will find that I have it right.

Posted by: Rick Lockyer on October 5, 2008 3:26 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Excuse my butting in here, but here it sounds like you are claiming that if we define the multiplication of “left octonions” by the rule

$m_{left}(e_i, e_j) = m_{right}(e_j, e_i)$

(where $m_{right}$ is defined according to the slide 16 representation) then the algebra of left octonions is not isomorphic to the right octonions.

But the linear map defined by

$e_i \mapsto -e_i$

is such an isomorphism, since

$(-e_i)(-e_j) = -(e_j e_i).$

One way of characterizing the octonions is that they form the unique (up to isomorphism) 8-dimensional composition algebra over the real numbers. A proof is given by Conway and Smith in their book On Quaternions and Octonions (and I think John has also written about this). That appears to contradict this assertion:

Since what I call Right and Left Octonion Algebras have non-equivalent multiplication tables, the algebras produced by each are not isomorphic algebras, but have all of the features typically given to Octonions.

at least assuming that the algebras of “right octonions” and “left octonions” each have the feature of giving an 8-dimensional composition algebra.

Posted by: Todd Trimble on October 5, 2008 8:06 PM | Permalink | Reply to this

Re: 8

I define Right and Left Octonion representations consistent with John’s Fano plane a few posts up being “Right” and the same but with the vertex bisector arrows pointing in the opposite direction as “Left”.

Let me use the shorthand (ijk) to represent basis units ei, ej and ek and their permutation inferred multiplication signs in normal fashion. The seven following triplets could be worked into John’s Fano plane with “4” being the central value:

(123)
(761)
(572)
(653)
(541)
(642)
(743)

This is “Right” octonion with 1,2 and 3 at the midpoint of the sides of the Fano plane triangle, being included also in one of the seven triplets. Now grab each of the three triplets that include one of the units, in succession, and allow me a cyclic shift to put the selected unit in the central position:

1:
(312)
(617)
(415)

2:
(123)
(725)
(426)

3:
(231)
(536)
(437)

4:
(541)
(642)
(743)

5:
(257)
(653)
(154)

6:
(761)
(365)
(264)

7:
(176)
(572)
(374)

Notice the right three members of each group of three triplets are found within another permutation triplet and the left side members are not. This is where the name “Right” comes from.

Now draw the vertex bisectors the other way with 4 still central. We have now

(123)
(761)
(572)
(653)
(145)
(246)
(347)

and

1:
(312)
(617)
(514)

2:
(123)
(725)
(624)

3:
(231)
(536)
(734)

4:
(541)
(642)
(347)

5:
(257)
(653)
(451)

6:
(761)
(365)
(462)

7:
(176)
(572)
(473)

Now the three left members are found inside a single permutation and the right side is not. This is “Left” Octonion.

This could have been totally anticipated by cycling each unit number through the central position in both of the Fano plane representations I indicated above.

This consistent right vs. left formation in the permutations is an intrinsic requirement for the algebra of octonions. If this is missing, you will not have an algebra that is octonion.

My point is that these are not isomorphic representations since the multiplication tables they represent are not equivalent, they are distinctly different.

I do not think I have missed something here, but maybe I have. Rather than dogmatically stating it cannot be, could someone demonstrate the errors of my ways? This would require demonstrating the two multiplication tables are equivalent.

Todd and John, please check out the information on my website: www.octospace.com for more details.

Posted by: Rick Lockyer on October 5, 2008 11:36 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Rick, if you take your example of a right multiplication table, then rename $e_4$ as $-e_4$ , you’ll get your example of a left multiplication table. And vice versa.

In other words, the basis $\{e_0,e_1,e_2,e_3,-e_4,e_5,e_6,e_7\}$ , where the $e_i$ are the elements of the original basis, will have a multiplication table that is “left” if that of the original basis is “right”, and “right” if that of the original basis is “left”.

Posted by: Greg Egan on October 6, 2008 1:05 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Todd: I think the problem is that Rick is treating an algebra as what we’d call an ‘algebra with specified basis’.

So, what the rest of the world considers to be the same algebra with two different bases, he considers to be two nonisomorphic algebras — unless he can permute one basis to get the other. More general changes of basis, like $e_i \mapsto -e_i$ , can’t give algebra isomorphisms as far as he’s concerned.

I tried to explain this, but it didn’t work. Maybe you can do better, but it’ll probably require some serious pedagogical work. After all, Lockyer approvingly cites Kantor and Solodovnikov’s correct definition:

Definition 14.1 Two $n$ -dimensional algebras are said to be isomorphic if they have bases with identical multiplication tables.

but he reads it as saying the basis is part of the structure of the algebra, instead of what’s really intended: two algebras are isomorphic if there exist bases for these algebras that give them identical multiplication tables!

Posted by: John Baez on October 6, 2008 12:02 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Yes, it sounds like you’ve pinpointed the source of the misunderstanding, and probably this sort of misunderstanding was quite common at one point in history, when vector spaces and algebras were primarily thought of as given by bases and multiplication tables – in other words, by taking presentations as primary. In fact, I wouldn’t be surprised if this type of misunderstanding were still commonplace [not meaning to diss physicists, but the way they often define specific Lie algebras in graduate textbooks tends to have a very basis-dependent feel to it, as if Lie algebras were primarily finite sets of generators obeying rules for taking brackets].

I’m not sure I’m up for a serious pedagogical work, and you may have already made the point effectively enough with your last comment. The point of view we are taking is that of modern algebra, that an algebra is a vector space $V$ equipped with a multiplication map

$m: V \times V \to V$

which is bilinear and possesses a unit. No specific basis (and therefore no specific multiplication table) need be presupposed, although by picking a basis we do get a corresponding multiplication table in terms of that basis.

There is a well-known definition of what it means for two algebras (understood according to the definition of algebra above) to be isomorphic, and this definition of isomorphism is also basis-independent. Perhaps it would be useful for the discussion to remember that definition: two algebras $A$ , $B$ (defined over the real numbers $\mathbb{R}$ ) are isomorphic if there is an invertible $\mathbb{R}$ -linear transformation $f: A \to B$ such that

$f(m_A(a, a')) = m_B(f(a), f(a')) \qquad f(1_A) = 1_B$

where 1’s denote multiplicative units in the respective algebras.

I’m assuming of course that Rick is listening in (after all, why would I be telling you any of this stuff, which you know so well?). But since the definition of algebra isomorphism is phrased in a way which is slightly different from how K-S put it, we should make clear how this definition is equivalent to (our reading of) K-S’s definition.

So, again for Rick’s benefit: if $f: A \to B$ is an algebra isomorphism in the sense of modern algebra (i.e., as defined above), and if $e_1, \ldots, e_n$ is a basis for $A$ , then the algebra structure on $A$ specifies a multiplication table in terms of the $e_i$ . The point is that the basis $f(e_i)$ for $B$ is a basis having a multiplication table whose structure is identical to the one for $A$ . It might not be the same table as one defined in terms of some other (preferred) basis for $B$ you may have started with to specify the structure of $B$ , but that was of course your point: we’re not playing favorites with choice of basis; a basis is not considered part of the algebra structure.

But since getting away from basis-dependent thinking takes time, it might be good to say this one more time. So: suppose that $A$ and $B$ are two algebras defined by multiplication tables, say in terms of bases $e_1, \ldots, e_n$ and $e_{1}', \ldots, e_{n}'$ respectively. Then a linear transformation $f: A \to B$ defined by a matrix $(a_{i j})$ :

$f(e_i) = \sum_{j=1}^n a_{i j}e_{j}'$

is an algebra isomorphism if the new basis $f(e_i)$ of $B$ , multiplied out by expanding each of these elements as linear combinations of the $e_{j}'$ , following the multiplication table for the $e_{j}'$ ’s, and then writing the results back in terms of the new basis $f(e_i)$ , gives a multiplication table for the $f(e_i)$ which is identical in structure to the table for the $e_i$ . That is, if we rewrite the multiplication table of the $e_{j}'$ purely in terms of the new basis $f(e_i)$ , we should get a table identical to that for the $e_i$ . That of course is what K-S meant, as you were saying.

So getting back to this from Rick:

I define Right and Left Octonion representations consistent with John’s Fano plane a few posts up being “Right” and the same but with the vertex bisector arrows pointing in the opposite direction as “Left”.

Right, just as I thought. Looking at John’s Fano plane representation, if for example the “right product” of $e_3$ followed by $e_4$ is $e_3 e_4 = e_6$ , then for the left product we have $e_6 e_4 = e_3$ (following the directions of the arrows). For either product each of the $e_i$ ’s are square roots of -1, so for the left product we have, e.g.,

$e_3 \cdot_{l} e_4 = (e_6 \cdot_l e_4) \cdot_l e_4 = e_6 \cdot_l (e_4 \cdot_l e_4) = -e_6 = e_4 \cdot_r e_3$

where in the second equation I have used a case of the alternating law. In general we have

$e_i \cdot_l e_j = e_j \cdot_r e_i$

and we can take this as another definition of the left product in terms of the right product.

So, the claim is that there is an algebra isomorphism, i.e., an invertible linear map

$f: (\mathbb{R}^8, \cdot_r) \to (\mathbb{R}^8, \cdot_l)$

such that

$f(e_i \cdot_r e_j) = f(e_i) \cdot_l f(e_j)$

Indeed, define $f(e_i) = -e_i$ , $f(1) = 1$ , and linearly extend the definition of $f$ to all of $\mathbb{R}^8$ . Then for $i \neq j$ we have

$f(e_i) \cdot_l f(e_j) = (-e_i) \cdot_l (-e_j) = e_i \cdot_l e_j = e_j \cdot_r e_i$

which is $-(e_i \cdot_r e_j) = f(e_i \cdot_r e_j)$ if $i \neq j$ , and $-1 = f(-1) = f(e_i \cdot_r e_j)$ if $i = j$ . So we do get an algebra isomorphism.

I do not think I have missed something here, but maybe I have. Rather than dogmatically stating it cannot be, could someone demonstrate the errors of my ways? This would require demonstrating the two multiplication tables are equivalent.

I hope this clears it up.

[Edit: Greg Egan has already made my last point very succinctly, but perhaps my spelling it out will serve a useful purpose after all. We’ll see.]

Posted by: Todd Trimble on October 6, 2008 3:16 AM | Permalink | Reply to this

Re: 8

Thank you all for clearing up my misunderstanding. Yes, I was looking at things from a structure position and not considering the basis change possibility. I did read John’s response, but could not immediately respond back since I am technically still on vacation and using my niece’s computer here in VA and had to go to dinner. I am flying back home to CA tomorrow.

John’s response allowed me to make the connection Greg mentioned above of changing e4 to -e4. It further allowed me to understand that a “Right” to “Right” change of say, negating the 4 permutations that do not contain e4 can be had not only by row-column swaps in the multiplication table, but also by (e1 to -e1) AND (e5 to -e5), or (e2 to -e2) AND (e6 to -e6), or (e3 to -e3) AND (e7 to -e7) with the set of seven permutations I stated.

My training is physics, so I guess the crack above was appropriate in my case. No offense taken. My interest in the variability of definition for the octonions is purely structure oriented, perhaps I have been too focused on this.

Thanks again.

Posted by: Rick Lockyer on October 6, 2008 4:23 AM | Permalink | Reply to this

Re: 8

Todd, I hope I’m not injecting any confusion here, but Rick’s example for his “left” octonion multiplication table, given here, only inverted some of the Fano plane arrows, not all of them.

That doesn’t change anything in principle, but I just want to be clear that the isomorphism you give addresses the multiplication table when all the arrows are inverted, while the one I give addresses the (different) multiplication table that Rick spells out explicitly here.

Posted by: Greg Egan on October 6, 2008 4:23 AM | Permalink | Reply to this

Re: 8

You’re right, I misread. Thanks for clearing that up!

Posted by: Todd Trimble on October 6, 2008 12:52 PM | Permalink | Reply to this

Re: 8

Well actually it goes something like this.

Without getting too “pedagogical” hopefully, there are 16 different ways to define the octonion basis (+e0,+e1,+e2,+e3,+e4,+e5,+e6,+e7) where I add the “+” here for emphasis. The method for their determination is assuming a permutation rule for ei ej = ek, ijk not equal or zero, the fact that permutation (ijk) is different than (jik), and that Artin’s Rule must hold. Doing the math on the 128 combinations of choice (ijk) or (jik) leads to the 16 possibilities.

When you claim the basis change ei to -ei gives the same multiplication table as the potentially non-isomophic algebra, what you are actually stating is “if I modify something, then unmodify it, do I get back to the same thing”. Obviously this is true, for (-ei,ej,ek) is equivalent to (ej,ei,ek), and this was the method by which the distinction between the 16 ways above was made in the first place.

The inflicted structure change you claim restores isomorphism is not required when comparing “Right” Octonion to other “Right”, or “Left” to other “Left”, it is only necessary when comparing “Right” to “Left”. Similarly, you can’t drop a (+)basis “Left” basis into a “Right” Fano plane representation without resorting to a negation which essentially switches arrow directions to a “Left” Fano plane representation and vice versa.

I also believe another K-S claim that an algebra is defined by its multiplication table. Any (+)basis “Left” multiplication table is distict from any (+)basis “Right” multiplication table. The (+)basis representation of the 16 forms is perfectly legitimate, perhaps more so than your “do” then “undo” method.

Posted by: Rick Lockyer on October 8, 2008 4:54 PM | Permalink | Reply to this

Re: 8

Allow me to bottom line the octonion basis element negation process.

Starting with a valid octonion basis, say (+e0,+e1,+e2,+e3,+e4,+e5,+e6,+e7), any even number of negations of elements 1 through 7 is an isomorphism. Any odd number of negations is not an isomorphism.

As I have discussed within information on my website, there are two modifications to the set of seven permutations that do not break Artin’s Rule. One is the negation of the three permutations that include any one of the seven non-scalar elements. The other is negation of the four permutations that do not include any one of the elements. The former is “Right” to “Left” or vise versa. The latter is “Right” to “Right” or “Left” to “Left”. Of course any number or combination of these elemental moves is valid.

The negation of the three permutations that include ei can be produced by changing ei to -ei. Assuming one permutation is (ijk), changing ei to -ei and ej to -ej is equivalent to negating the four permutations that do not contain ek. I brought this up in a previous post in this thread. The combinations of these moves demonstrates the even-odd rule above.

Look at the (+)basis multiplication tables for “Right” and “Left”. They are different, and define non-isomorphic representations. Your mistake was assuming the move ei to -ei does not change the definition of the algebra. It does.

Posted by: Rick Lockyer on October 10, 2008 4:08 PM | Permalink | Reply to this

Re: 8

Rick, can you please tell me which – if any – of the following statements you disagree with?

If you agree with all of them then I’m happy to go on to discuss whatever specific calculation (by myself or by Todd?) you are disputing, but before spending any time on that I’d like to know if we’re having another disagreement on basic principles.

(1) The product on an algebra can be completely defined by specifying the multiplication table for some basis. If you want to multiply any pair of vectors, you just expand them in the basis for which you have the multiplication table, and use the linearity of the product to get your answer.

(2) But what that does not mean is that two algebras that happen to have different multiplication tables for certain choices of basis on each one are necessarily non-isomorphic.

The definition of isomorphic algebras that you have quoted is:

Definition 14.1 Two n-dimensional algebras are said to be isomorphic if they have bases with identical multiplication tables.

(3) Not all choices of bases for the two algebras have to yield identical multiplication tables; all that’s required is that a choice of basis exists, separately, for each algebra such that the two multiplication tables become identical.

(4) Under this definition, there is only one 8-dimensional normed division algebra, and it’s always called the octonions.

(5) It’s possible to write down lots of different multiplication tables for this algebra, but if you give me two such multiplication tables, I can always find a new basis such that the product defined by the second multiplication table and the old basis, yields a multiplication table under the new basis that is identical to the first multiplication table under the old basis.

(6) In applying the definition of algebra isomorphism to show that two non-identical multiplication tables nonetheless describe isomorphic algebras, the simplest thing is to treat the two multiplication tables as applying in two different 8-dimensional vector spaces. We leave the basis and the multiplication table on the first vector space untouched; we change the basis on the second vector space, and also change the second multiplication table in such a way that the product (as a basis-independent function of two vectors) is unchanged. This change allows us to come up with a multiplication table on the second vector space identical to that on the first vector space.

Posted by: Greg Egan on October 11, 2008 1:44 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

I wrote:

I’m happy to go on to discuss whatever specific calculation (by myself or by Todd?) you are disputing …

Since I’m going to be travelling out of internet range for 2 weeks from Sunday, I’ll take the liberty of re-stating my own calculation.

Rick, two examples of multiplication tables you have previously cited are, for “Right”:

e 1 e 2 e 3 e 4 e 5 e 6 e 7 e 1 - 1 e 3 - e 2 - e 5 e 4 - e 7 e 6 e 2 - e 3 - 1 e 1 - e 6 e 7 e 4 - e 5 e 3 e 2 - e 1 - 1 - e 7 - e 6 e 5 e 4 e 4 e 5 e 6 e 7 - 1 - e 1 - e 2 - e 3 e 5 - e 4 - e 7 e 6 e 1 - 1 - e 3 e 2 e 6 e 7 - e 4 - e 5 e 2 e 3 - 1 - e 1 e 7 - e 6 e 5 - e 4 e 3 - e 2 e 1 - 1

and for “Left”:

e 1 e 2 e 3 e 4 e 5 e 6 e 7 e 1 - 1 e 3 - e 2 e 5 - e 4 - e 7 e 6 e 2 - e 3 - 1 e 1 e 6 e 7 - e 4 - e 5 e 3 e 2 - e 1 - 1 e 7 - e 6 e 5 - e 4 e 4 - e 5 - e 6 - e 7 - 1 e 1 e 2 e 3 e 5 e 4 - e 7 e 6 - e 1 - 1 - e 3 e 2 e 6 e 7 e 4 - e 5 - e 2 e 3 - 1 - e 1 e 7 - e 6 e 5 e 4 - e 3 - e 2 e 1 - 1

What I claim is that these two different multiplication tables define isomorphic algebras. To show that, imagine that each multiplication table has been used to define a product on a different vector space: on vector space $V$ we’ve chosen a basis, then used the first (“Right”) multiplication table to define our product on $V$ , and on vector space $W$ we’ve chosen a basis, then used the second (“Left”) multiplication table to define our product on $W$ .

We leave everything as it is on $V$ , but now ask the question: if we express the product we’ve defined on $W$ in terms of a multiplication table for a new basis, can we get the same multiplication table for the product on $W$ as we have on $V$ ?

And the answer is: Yes! If we choose the basis $\{e_0,e_1,e_2,e_3,-e_4,e_5,e_6,e_7\}$ as our new basis on $W$ , then there will be two kinds of changes we have to make to the multiplication table. The first is that every time we get an answer of $e_4$ or $-e_4$ in the table, we have to swap the sign. The other is that all the signs in the row and the column where $e_4$ is one of the elements we’re multiplying have to change (except, of course, $e_4 * e_4 = -1$ ).

Making those changes to the second (“Left”) multiplication table converts it into an exact copy of the first (“Right”) multiplication table. So we have shown that the algebras defined via the two different multiplication tables are actually isomorphic.

You wrote:

Any odd number of negations is not an isomorphism.

I don’t really know what you’re claiming here, but maybe you’re getting stuck on the fact that negating an odd number of elements of an orthonormal basis is not a rotation. That’s certainly true, but it doesn’t change anything. In the K-S definition, if you have two products defined on the same vector space $V$ , then those products describe isomorphic algebras so long as you can find two bases (one for each product) such that the multiplication tables become identical. What it does not require is that those two bases have the same orientation! If one basis has to be a mirror image of the other, that’s perfectly fine. It doesn’t stop the algebras being isomorphic.

Posted by: Greg Egan on October 11, 2008 6:24 AM | Permalink | Reply to this

Re: 8

Greg,

There is no need to repeat these claims, I do see the math quite clearly. That is not where the problem is. Answering your questions above also does not seem to be the best path to your understanding my position.

We seem to have some common ground with the K-S book. On page 55 of the edition I have, they state:

“Our definition makes it clear that an n-dimensional algebra is completely determined by its “multiplication table”(7.5)…..In principle, these numbers are not subject to any restrictions; each choice determines a certain algebra”

You wrote:

(4) Under this definition, there is only one 8-dimensional normed division algebra, and it’s always called the octonions.

Yet you also state there are many multiplication tables, and agree my “Left” and “Right” multiplication tables are different. Apparently you do not subscribe to the K-S quote I just referenced, for if you did, you would not be able to claim (4) without demonstating there is actually only one multiplication table for the octonions.

I have shown that fundamentally there are not 1, 480, many, but two multiplication tables for the octonions, and hence two definitions for octonion multiplication, and by virtue of the provided K-S quote two definitions for octonion algebra. “Right” octonion lives in one multiplication table, and “Left” octonion lives in the other.

The basis change e4 to -e4 is certainly allowed for purposes of defining vector spaces. But as you have stated, you can change the multiplication table with this move. What you fail to see is that by what I consider the proper interpretation of

Definition 14.1 Two n-dimensional algebras are said to be isomorphic if they have bases with identical multiplication tables.

you have changed the multiplication table (by design) and hence have made a non-isomorphic move by definition. Yet you use this non-isomorphic move (yes by my interpretation) to demonstrate continuity of isomorphism. That is not logical.

You hinge your argument on the free ability to negate basis elements as you could for defining vector spaces. For the purposes of defining the operation of multiplication with basis elements you are not so free. By changing +e4 to -e4, you are redefining the algebra by virtue of changing its multiplication table. You have lost continuity between the algebras and hence have no claim to a demonstration of isomorphism between them.

Posted by: Rick Lockyer on October 11, 2008 3:21 PM | Permalink | Reply to this

Re: 8

With your response back here, I had assumed the matter was settled. Since then you have posted three times, and in each of these cases you demonstrate your (renewed?) ignorance of the standard notion of algebra isomorphism, which was explained back here. Greg has patiently explained, several times by now, why your right octonions and left octonions are isomorphic according to this standard definition; you say you see the math clearly, and yet you refuse to acknowledge well-known and clearly demonstrated facts.

Since you cite “K-S” as your point of reference for everything you’ve said, I might suggest you contact the authors and ask them to evaluate your claim that the right octonions and left octonions are non-isomorphic according to the standard notion of algebra isomorphism. [In fact, I’m tempted to do this for you.] At any rate, I’m beginning to think that efforts on the part of people here to convince you otherwise are simply a waste of time.

Posted by: Todd Trimble on October 11, 2008 5:44 PM | Permalink | Reply to this

Re: 8

A little while back, Todd said

“this sort of misunderstanding was quite common at one point in history, when vector spaces and algebras were primarily thought of as given by bases and multiplication tables… In fact, I wouldn’t be surprised if this type of misunderstanding were still commonplace”

Unfortunately in my experience it is very commonplace, not only among physicists but also among undergraduate mathematicians. A related problem that I have experienced too much is students being convinced that a vector is a column (or row) of numbers, and that a vector space is a collection of such things.

PS I’m sorry that I can never remember how to quote other people in that nice indented way.

Posted by: Eugenia Cheng on October 11, 2008 8:50 PM | Permalink | Reply to this

Re: 8

One more try: If there are two algebras in dispute, they have each a basis
multiplication tables are not with repect to a common basis.

Posted by: jim stasheff on October 12, 2008 1:53 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Our definition makes it clear that an n-dimensional algebra is completely determined by its “multiplication table”(7.5) … In principle, these numbers are not subject to any restrictions; each choice determines a certain algebra.

Each choice of multiplication table determines a certain algebra, but it doesn’t follow that different choices of multiplication table determine different algebras. Each choice of two distinct points in space determines a straight line, but it doesn’t follow that two different pairs of points necessarily determine different lines.

Look at the definition:

Definition 14.1 Two n-dimensional algebras are said to be isomorphic if they have bases with identical multiplication tables.

There is no restriction placed here on the choice of basis that can be made in order to bring the multiplication tables into agreement. You’re quite happy to choose a new basis which is a permutation of the original basis; that’s the underlying process by which you perform row and column swaps to bring two of what you call “Right” multiplication tables into perfect agreement. But restricting the choice of the new basis to permutations of the old one is just something you’re imposing yourself; it does not appear in Definition 14.1.

When the product of two vectors is defined according to some basis $B_1=\{e_0,e_1,e_2,e_3,e_4,e_5,e_6,e_7\}$ and your “Left” multiplication table, then it follows that the same product yields a multiplication table with respect to the basis $B_2=\{e_0,e_1,e_2,e_3,-e_4,e_5,e_6,e_7\}$ which is precisely your “Right” multiplication table. What is it you can point to in Definition 14.1 that rules out $B_2$ as a “basis”? The fact that it has a different multiplication table? Any change of basis changes the multiplication table in some way; that’s the whole point!

When you conclude that two non-identical “Right” multiplication tables describe isomorphic algebras, what validates that conclusion under Definition 14.1 is the fact that the multiplication tables change under changes of basis. The fact that permutations of the basis yield a restricted set of changes to the multiplication table is beside the point; nothing in Definition 14.1 calls for such a restriction.

you would not be able to claim (4) without demonstrating there is actually only one multiplication table for the octonions.

No, what would prove claim (4) is showing that, given any two multiplication tables for 8-dimensional normed division algebras, it would be possible to bring those two multiplication tables into agreement by changing the bases that are being multiplied. And the new bases can be any two sets of n linearly independent vectors; that’s the definition of a basis, and nothing in Definition 14.1 restricts the term further.

Giving a proof that the octonions are the unique 8-dimensional normed division algebra is beyond me; John Baez cites Richard D. Schafer, Introduction to Non-Associative Algebras, Dover, New York, 1995. But proving that your examples of “Right” and “Left” octonions are isomorphic is trivial; the change of basis I’ve described does that.

It’s a shame to be quibbling over definitions, but if you search the literature you’ll find that nobody else on the planet talks about two kinds of octonion depending on the orientation issues you’re describing. Arguably this is ultimately just a matter of convention … but it is the convention that Definition 14.1 describes, so you’re going to have a lot of trouble communicating with mathematicians if you insist on adopting a different convention of your own.

Posted by: Greg Egan on October 11, 2008 5:30 PM | Permalink | Reply to this

Re: 8

Lets talk money!

I ask you to pull a penny out of you pocket. You do so. I ask you, “can you see both sides at the same time?”. Of course your answer is “no, I can not”. I ask you, “are both sides of the coin identical?”. Since it is the penny we are all familiar with, you say “no, the sides are different”.

Since neither of us can see both sides at the same time, we agree there is no loss of generality by placing the coin on my table with one side up. You do so. I ask you to flip the orientation of the coin an even number of times, your choice of count, then I ask you “did the face change when you did this?”. your answer is of course “no”. Now I ask you to do an odd number of flips, again your choice of count. I ask you now “did the face change when you did this?”. Now your answer is “yes”.

I lift my hand and reveal my own coin, and I tell you I will slap your hand if you try to touch my money. I ask you “are our coins identical?”. Now, the next part can play out two different ways. I will do one way with full confidence you can figure out the other on your own.

You look at my coin face, then you look at your coin face, and you notice they are different. You say to yourself “Self, I see these are not the same, but I recognize yours is the same as the other side of mine, so I will flip my coin over, since you have not told me I can’t, and will show that yes, they are the same”.

But I say “hold the bus, you do not know what is on the other side of my coin, so how can you say that they are the same?”. At this point, you are left saying “ahh, err, hmm, I guess you are correct”.

Now the next scene can be played out two ways when I reveal the other side of my coin. I may have a penny that somehow did not get stamped on the other side, in which case we both agree the coins are not the same. Or, I may have a normal penny, and on an ADDITIONAL comparison, we see that yes, the coins are the same.

Of course, the octonions are the “coin” and not “a face”. However, each “face”, here “Left” octonion and “Right” octonion, are full blown algebras on their own. Neither needs the other, and they DO have fundamentally different multiplication tables. We all believe that the 8 “Right” forms are isomorphic, and the 8 “Left” forms are isomorphic, since we can come to the same conclusion by applying our interpretations of the concept of isomorphism.

We have been bashing each other with K-S definition 14.1. There is nothing in this definition that would require me to turn over my coin before asking the above question of sameness. For I can say “No, I will not do so. I have presented you an algebra by showing you this side only, therefore my question should be answerable”. We all can see the only answer is, “what you show me is isomorphic to ONE side of my coin. I do not know if the other side of your coin is isomorphic to mine until you show it to me and allow me a second test”. This has been my position all along. You think?!!

I can’t state it any plainer than this. Whether or not you agree, I think you need a new word to cover the octonions and their duality of algebraic structure embodied by the two different multiplication tables. Finding “oneness” through isomorphisn is a hack on the definition.

Posted by: Rick Lockyer on October 12, 2008 7:42 PM | Permalink | Reply to this

Re: 8

I think you need a new word to cover the octonions and their duality of algebraic structure embodied by the two different multiplication tables.

“Involutory automorphism”. Now, can we move on?

Posted by: John Armstrong on October 12, 2008 8:17 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Rick wrote:

I think you need a new word to cover the octonions and their duality of algebraic structure embodied by the two different multiplication tables.

John A. wrote:

“Involutory automorphism”.

An involutory automorphism is, as you know, an isomorphism from a structure to itself, which gets you back where you started when you do it twice.

Rick seems to be using ‘algebra’ to mean what we’d call ‘algebra with a chosen basis’. As such, the left and right octonions are distinct entities. They’re not even isomorphic. So for him, the term ‘automorphism’ would be inappropriate for the relation between them.

Now, can we move on?

I had, but…

Posted by: John Baez on October 12, 2008 10:38 PM | Permalink | Reply to this

Re: 8

I think we can profitably end this thread here. Everyone agrees on the definition of ‘algebra isomorphism’ except Rick Lockyer, who has his own better definition.

Posted by: John Baez on October 12, 2008 8:15 PM | Permalink | Reply to this

Re: 8

Hi everybody. I would like adding an additional reason to love 8: 8 is also a number magic, both, in the shell electronic atomic model (electronic configuration) and the nuclear shell model. Moreover, any reason to link higher magic numbers and the octonionic stuff??? I love this blog, many thanks…

Posted by: Juan Francisco on September 19, 2008 7:01 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Near the top of the periodic table, an element whose atomic number is twice a square number will be exceptionally stable: a noble gas. Helium has 2 electrons, neon has 8, and argon has 18.

The reasons behind this are well-understood. But, instead of explaining the reasons, let me just note: the irreducible representations of $SO(3)$ have dimensions that are odd numbers, the sums $1, 1+3, 1+3+5, \dots$ are perfect squares, and the factor of 2 comes from the fact that each electron comes in ‘spin-up’ and ‘spin-down’ states.

For elements with more electrons, you can’t ignore interactions between electrons, which make things a lot more complicated. At this point in the periodic table we get the transition metals, and the pattern I described breaks down.

For an infinitely better explanation of all this stuff, try:

Shlomo Sternberg, Group Theory and Physics, Cambridge University Press, Cambridge, 1994.

I don’t understand the magic numbers in nuclear physics, so I don’t know why 8 is also one of those.

I doubt very much that the octonions are involved.

Posted by: John Baez on September 23, 2008 3:31 AM | Permalink | Reply to this

Re: 8

Hi, John. Yes, I perfectly know the reasons to close shells in the atomic model. I didn’t know that book by Sternberg!Thanks for the reference. The nuclear shell model is based on a hamiltonian that is, H_free+H_harmonic oscillator+H_spin_orbit.The magic numbers are precisely numbers of nucleons ( protons and/or neutrons) that makes nuclei more stable to decay( in the same way like the one you mentioned for the atoms are more stable …)…The spin_orbit is the term whick allows us to mimic the magic numbers seen: Z,N=2,8,20,28,50,82,126…
The deep reason of this stuff, as far as I know, beyond the spin-orbit explanation, are unknown ( in fact there was discussion between nuclear physicists thinking 114 was also magic-a bad use of the hyperdeformation was the cause of that wrong fact. The amusing think, is that ,as far as I know, no one knows if periodic table ends or not (both in atoms or nuclei)…Since very soon I hope we can produce elements in the 8th period,…someone has tried to derive some kind of periodicity 8 in the periodic table on theoretical ground and TERMINATE the periodic table?Multielectronic and multinucleonic states are complex of course, but I was wondering if new ways to picture Mendeleiev’s table had been tried.

Posted by: Juan Francisco on September 23, 2008 6:29 PM | Permalink | Reply to this

Re: 8

John wrote in part:

Helium has 2 electrons, neon has 8, and argon has 18.

No, neon has 10 electrons.

The atomic numbers of the noble gasses are 0, 2, 10, 18, 36, 54, 86, 118, …. The differences between these (the lengths of the periods) are 2, 8, 8, 18, 18, 32, 32, …. The differences between these (ignoring repetitions) are 6, 10, 14, …. And the differences between these are always 4.

That, at least, is how I understand the periodic table. (You also have to know where to stick the new groups in when the period lengths increase, but that’s easy if you look along the left side.)

Posted by: Toby Bartels on September 28, 2008 12:25 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Toby wrote:

No, neon has 10 electrons.

Ouch.

I actually did know that oxygen has 8 electrons. Honest. Somehow I got seduced by the story I was trying to tell.

The atomic numbers of the noble gasses are 0, 2, 10, 18, 36, 54, 86, 118, …. The differences between these (the lengths of the periods) are 2, 8, 8, 18, 18, 32, 32, …. The differences between these (ignoring repetitions) are 6, 10, 14, …. And the differences between these are always 4.

Wow, okay. Do you have any idea why it works like this?

Here’s my new guess — it’s still wrong, but maybe it’s closer. Each new ‘shell’ should have $2n^2$ electrons in it, because there’s a hidden $SO(4)$ symmetry in the Kepler problem, and $SO(4)$ has a bunch of irreps of dimension $n^2$ coming from the homomorphism $SU(2) \times SU(2) \to SO(4)$ and the fact that $SU(2)$ has one irrep of dimension $n$ for each $n \ge 1$ . The extra factor of 2 comes from the two choices of spin for each electron.

This would predict rows of the periodic table with lengths $2,8,18...$ , and thus noble gases with these numbers of electrons: $2,$ $2+8 = 10,$ $2+8+18 = 28,$ and so on.

But you’re saying the right answer is $2,$ $2+8 = 10,$ $2+8+8 = 18,$ $2+8+8+18 = 36,$ $2+8+8+18+18 = 54,$ $2+8+8+18+18+32 = 86,$ and so on. That seems to work. But why the repetition of each number… except the number 2?

Posted by: John Baez on September 28, 2008 11:14 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

The number of electrons in each orbital, or $(n,l)$ pair, looks like this:

$\array{&l=0&l=1&l=2&l=3&l=4&l=5\\&s&p&d&f&g&h\\n=1&2\\n=2&2&6\\n=3&2&6&10\\n=4&2&6&10&14\\n=5&2&6&10&14&18\\n=6&2&6&10&14&18&22}$

The order in which the orbitals are filled follows the Aufbau principle, in which the lowest values of $n+l$ are filled first (and the lowest $n$ for equal $n+l$ ):

Helium, $Z=2$ , and Neon, $Z=10$ , have full shells up to $n=1$ and $n=2$ respectively, but Argon, $Z=18$ , only fills the first two orbitals of the $n=3$ shell. But that’s still a relatively stable configuration, because the next orbital to be filled is the $n=4, l=0$ or $4s$ orbital.

So you find the number of electrons in a noble gas by looking at how you fill the $l=1$ , or $p$ , orbital, for each value of $n$ , because the next orbital to fill will be the $s$ orbital for a higher value of $n$ .

Posted by: Greg Egan on September 29, 2008 1:19 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

People with a childish streak may enjoy the song 8 is a Number by a group called Central Services.

The group They May Be Giants has come out with a whole album of number songs called Here Come the 123s. Unfortunately their song on the number 8 is not freely available online. But, you can hear Seven.

Posted by: John Baez on September 23, 2008 3:06 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Joseph Yoon has kindly emailed me the lyrics to the song Eight is a Number:

In a world of danger there is only one number that you need
In the ancient era it means strength, it stands for wealth in Chinese
It’s the number of the planets that our solar system has left
Now that Pluto couldn’t hack it because it didn’t have the heft

8 is the number of the center fielder
8 is the number of pawns in chess
8 is the number of sides in a stop sign
How many bits are in a byte, you guessed it: 8

Ooo, say you’re in a jam with no escape, oh-oh
And the only way to best your fall is to bake a cake
Well there’s 8 fluid ounces in a cup, 8 pinches to a teaspoon
If you know there’s 8 tablespoons to a gill you’re in a meet we’ll lose

8 is the number of legs on a spider
V8 has 8 vegetables
The time for the old movie starts at 8
Because 7 is too early and 9 is too late

8 is the number of the month of August
8-8 is the birthday of Smokey the Bear
8-8-8 means your call is free
When 8 seeks a nap that’s infinity

8 is the 7th Fibonacci number after 3 and 5
8 electrons make up the second orbital shell
18 years 11 days and 8 hours after an eclipse a nearly identical will come
Oxygen the very thing we breathe has an atomic number of 8

8 is the number that I wore in soccer
I played for 8 years until the 8th grade
I really liked to but I was only average
Because I was too afraid to head the ball

8 is the number of Yogi Berra
There are 8 countries in Central America
8 is the number of notes in a octave
The original Nintendo was only 8 bits

8 is the number of ghosts in Macbeth
8-8 is the birthday of Svetlana Savitskaya
8-8-8 means the call is free
When 8 seeks a nap that’s infinity

8 is a number
8 is a number
8 is a number
8 is a number
An integer, a digit
8, 8, 8
8 is a number
8 is a number
And 8 is enough

There could be mistakes in here. “Best your fall”? “You’re in a meet we’ll lose”? I’ll be glad to fix them if anyone listens to the song and get them figured out.

Posted by: John Baez on September 24, 2008 4:35 PM | Permalink | Reply to this

Re: 8

I love the 2-line non-8-related digression about his soccer career!

Posted by: Jamie on September 25, 2008 10:27 AM | Permalink | Reply to this

Re: 8

Some corrections to the song lyrics:

In the ancient Tarot (not era)
…to best your foe (not fall)
…to a gill your enemy will lose
…time that all movies start should be 8
…nearly identical one will occur

And the big correction is that this song is not by They Might Be Giants, rather The Board of Education (alter-ego of Central Services)

Posted by: Marc on October 20, 2009 12:30 PM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Thanks for the song lyric corrections. It makes a bit more sense now.

Posted by: John Baez on October 20, 2009 8:06 PM | Permalink | Reply to this

Re: 8

On the octonionic subject of non-associativity, I wonder what the simplest everyday example of that would be?

My guess would be (a*b) defined as the biological offspring of a and b. Then (a*b)*c describes a person with very different relationships to a, b and c than a*(b*c).

Posted by: Greg Egan on September 26, 2008 3:35 AM | Permalink | Reply to this

Re: 8

Hello everybody again!I found fun your explication on the periodic stuff, modulo your mistake with neon.Don’t worry:D. On the Greg last question, the easiest thing I can imagine is related to cross product. You know: (axb)xc is not equal to ax(bxc). Note that wedge producto IS associative ( from de grassmann number axiom system). So, as cross product can be obtained from wedge product via dual mal ( multiplication by the imaginary unit), then the cross product is somekind of “double” operation: first take the wedge product, then take the dual. From the physics viewpoint, wedge product is a bivector, cross product is, in tridimensional space a (pseudo)vector. What everyday physical objects describe cross products?Let me change the question:what common physical quantities are described/can be described by cross products, that is, non associative “things”?The answer,I think,is interesting. What this example shows is that cross products are something more than simple rotations in 3d. Imagine a rectangular piece of paper, and a cartesian system OXYZ.Put the paper in the OXY plane. If I define : a)U=90 counterclockwise rotation around OX,V=90 counterclockwise rotation around OY,W=90 counterclockwise rotation around OZ, we can see that (UV)W (paper) = U (VW) (paper). If we want nonassociativity, we could include in the “rotation definition” a supplementary add, something like a twist around or a bend with respect to the orthogonal axis. If you want, you can also take the dual from the oriented rotated paralelogram. It’s easier than bent papers, I guess. Then the result is non-associativity. Check if I haven’t got a mistake. In fact, I remember a certain nonassociative algebra “naturally” arise in the Monster group theory(griess algebra…But, of course, that is not a simple everyday example).

Posted by: Juan Francisco on October 4, 2008 7:44 PM | Permalink | Reply to this

Re: 8

Thanks, Juan. That still seems a bit artificial to me, but maybe there’s some simpler way the cross product can turn up.

Another example that occurred to me is cooking. Given three ingredients A, B and C and the operation “mix together thoroughly then heat for ten minutes”, there will be plenty of choices where (AB)C is nothing like A(BC).

Posted by: Greg Egan on October 5, 2008 1:31 AM | Permalink | Reply to this

Re: 8

John wrote:

The group They May Be Giants …

Might not “May”. Needless to say, this lame song is not their best work, or even their best math/science-nursery-rhyme-ish work.

Posted by: Greg Egan on October 5, 2008 3:14 AM | Permalink | Reply to this

Re: 8

John isn’t the first to accidentally say “May”. Indeed, on the album Miscellaneous T, John and John (Flansburgh and Linnell) include a recording from their answering machine where someone who has called their Dial-A-Song service doesn’t realize she’s being recorded and asks someone else, “Who’s ‘They May Be Giants’?”

And I’ve just completely outgeeked everyone else here. I’m going away now…

Posted by: John Armstrong on October 5, 2008 6:10 AM | Permalink | Reply to this

Re: 8

John Armstrong wrote:

And I’ve just completely outgeeked everyone else here. I’m going away now…

Ha! I can do better than that; I can tell you that it was two New York policemen discussing what kind of scam the Dial-A-Song line could possibly be. “How can they make money? It don’t make no sense.”

Posted by: Greg Egan on October 5, 2008 10:16 AM | Permalink | Reply to this

Re: 8

I wrote: two New York policemen.

Oops, sexist language alert. Two New York police officers, one male, one female.

Posted by: Greg Egan on October 5, 2008 10:58 AM | Permalink | Reply to this

Re: 8

$MathML-enabled post (click for more details).$

Greg wrote:

On the octonionic subject of non-associativity, I wonder what the simplest everyday example of that would be?

My guess would be $(a*b)$ defined as the biological offspring of $a$ and $b$ .

and then approximately

Another example that occurred to me is cooking. Given three ingredients $a, b$ and $c$ and the operation “mix together thoroughly then heat for ten minutes”, there will be plenty of choices where $(a b)c$ is nothing like $a(b c)$ .

Both these are a bit like taking the average of numbers:

$(a + b)/2$

which is also nonassociative.

I used to know it was They Might Be Giants. In fact I probably still have a cassette tape they released back in the 80’s — around the time when they were recording songs onto an answering machine and taking out ads for “Dial-A-Song”. One of the songs on this cassette went something like:

They might be giants
They might be giants
They might be big
They might be large
They might be big big big large large!

I found them a bit too goofy for my taste. I prefer my goofiness mixed with a bit more angst and alienation, as in early Talking Heads or Brian Eno.

Posted by: John Baez on October 5, 2008 7:38 AM | Permalink | Reply to this

Re: 8

John Baez wrote:

Both these are a bit like taking the average of numbers.

Ah, that’s the simple “non-chemical” example I was failing to think of.

I found [They Might Be Giants] a bit too goofy for my taste.

I agree sometimes, but I still love “Ana Ng”, and sometimes the “goofiness” gets closer to audacious surrealism and clever puns. And how can you go past these lines?

We were once so close to Heaven
Peter came out and gave us medals
Declaring us
The nicest of the
Damned

But I’d better shut up now, or I’ll end up in thread hijack purgatory.

Posted by: Greg Egan on October 5, 2008 10:30 AM | Permalink | Reply to this

The n-Category Café

Skip to the Main Content

September 7, 2008

8

Posted by John Baez

Some Related Entries

77 Comments & 0 Trackbacks

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Why We Love Numbers; Re: 8

Re: Why We Love Numbers; Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Easy As 1, 2, 3; Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8

Re: 8