News about E8
Posted by John Baez
The exceptional Lie group E8 is a marvelous 248-dimensional monster, with mysterious connections to the octonions and string theory. Here’s a nice webpage about a new calculation involving :
- American Institute of Mathematics, Mathematicians map E8.
As part of a project called the Atlas of Lie Groups and Representations, a team of mathematicians led by Jeffrey Adams have computed the Kazhdan–Lusztig–Vogan polynomials for .
You may have heard some hype about this, because it’s a really big calculation, and the American Institute of Mathematics has coaxed a lot of science reporters to write about it — in part by comparing it to the human genome project.
To see what was really done, try these:
- David Vogan, Narrative of the project to compute Kazhdan–Lusztig polynomials for E8.
- Jeffrey Adams, Technical details.
Computing the Kazhdan–Lusztig–Vogan polynomials for is certainly nowhere nearly as important as the human genome project, nor as hard!
However, the final result involves more data, in a sense. The answer is a 453,060 × 453,060 matrix of polynomials which takes 60 gigabytes to store. For comparison, see my webpage on information. The human genome is a mere 1 gigabyte – a pickup truck full of books. A good recording of the complete works of Beethoven takes 20 gigabytes. A library floor of academic journals holds 100 gigabytes.
So, the computation was indeed big.
But what’s , and what’s a Kazhdan–Lusztig–Vogan polynomial? You probably won’t hear the popular media tackle those questions. Let me give it a try.
The quickest way to describe may be this. Take equal-sized balls in 8 dimensions and find the densest possible lattice packing. If you center one ball at the origin, the centers of the balls form a lattice that is, a discrete set closed under addition and subtraction.
Then, form the quotient which is an 8-dimensional torus. There’s a way to measure distances on this torus, coming from the usual way to do this in . We’ve just wrapped up 8-dimensional Euclidean space into a torus!
Next, it’s a fact that every compact Lie group has a ‘maximal’ torus — a subgroup shaped like a torus that’s as big as possible while having this property. In fact there are lots of maximal tori, but they’re all alike, so people usually speak of ‘the’ maximal torus. You can measure distances on the maximal torus, since there’s a unique distance function on the Lie group that’s invariant under all the symmetries the group has. (Unique up to an overall scale factor, anyway — let’s not worry about that.)
The cool part is this: if someone hands you the maximal torus of a compact Lie group, together with how to measure distances on this torus, you can recover the Lie group! At least, you can if you’ve taken a course on this stuff.
So, the 8-dimensional torus I just described comes from a unique compact Lie group, and this group is !
I said this might be the quickest way to describe . But, to actually use this description to get your hands on is not so easy! The trick for getting compact Lie groups from the distance function on their maximal torus is something kids learn in grad school. The really hard part is to find the densest lattice packing of balls in 8 dimensions. Luckily, you can just look it up. This lattice consists of all vectors such that
- the numbers are either all integers or all half-integers (a ‘half-integer’ being an integer plus 1/2)
- the sum is even.
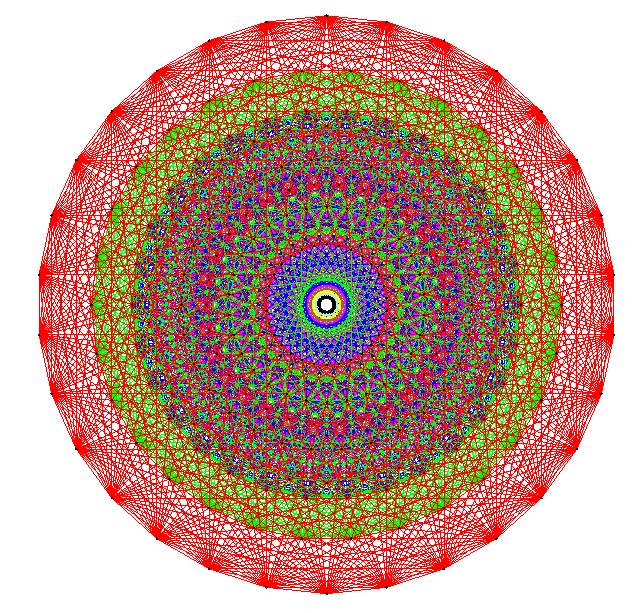
Here’s a picture of the densest lattice packing of balls in 8 dimensions:
Drawn by John Stembridge, this shows the centers of all 240 balls that touch a given one, with the center of each ball connected by a line to the centers of its nearest neighbors. Of course, the picture has been projected from 8 dimensions down to a mere 2.
Not very easy to visualize! If you’re really interested in this stuff, you may prefer the more precise description using coordinates given here:
- John Baez, review of On Quaternions and Octonions, by John Conway and Derek Smith.
Now, what about Kazhdan–Lusztig–Vogan polynomials? I don’t really understand them, but let me give it a try.
Actually, I’ll just discuss the Kazhdan–Lustzig polynomials for an easier bunch of groups, namely the groups , also known as . This will already be enough to make most of you run away screaming. But you shouldn’t: these groups describe the symmetries of -dimensional projective geometry. For , this is the geometry that governs the use of perspective in paintings!
In -dimensional projective geometry we have points, lines, planes, and so on up to the th dimension, and all we can talk about is whether a point lies on a line, or a point lies on a plane, or a line lies on a plane, etcetera. There are some axioms, a bit like the axioms of Euclidean geometry, but simpler, because the concepts of distance and angle are not present! After all, if you draw a painting of a scene from different perspectives, distances and angles on your painting will change — but the fact that two lines intersect at a given point will not.
As I explained back in week186, a flag in -dimensional projective geometry is a point, lying on a line, lying on a plane, lying on a… and so on, up to the th dimension. The set of all such flags is called the flag variety of .
If you pick one flag and call it your favorite, you can classify other flags by how they’re related to your favorite flag. For example, maybe the point of the other flag lies on the line of your favorite flag. Or maybe the plane of the other flag contains the point of your favorite flag. Or maybe both these things are true. There are lots of possibilities.
In fact, there turn out to be exactly possibilities. I won’t explain why, but there are lots of beautiful proofs of this beautiful fact.
So, if we classify flags according to this scheme, the flag variety gets partitioned into subsets. These are called Schubert cells.
The closure of a Schubert cell is called a Schubert variety.
We can define projective geometry, and thus the flag variety and Schubert varieties, over any field. Let use the finite field with elements. Then, if we fix two Schubert varieties, we can count the number of points in the intersection of their closures. This number will be some polynomial in . This is called an –polynomial. Since it depends on a choice of two Schubert cells, there are of these, naturally arranged in a square matrix.
The Kazhdan–Lusztig polynomials can be defined as certain linear combinations of -polynomials. They don’t count the points in the intersection of two Schubert varieties… they do something related, but subtler: they convey information about their intersection cohomology. Also, by the Kazhdan–Lusztig conjecture, subsequently proved by Brylinski, Kashiwara, Beilinson and Bernstein, they encode facts about the representation theory of the complex form of . A bit more precisely, they say stuff about how many times any given Verma module contains any given irreducible representation as a subquotient.
This is a quite deep subject — for some reason that’s what I always say when something is over my head. The so-called Weil conjectures (now theorems) play a role here, since they relate the number of points in a smooth variety over a finite field to the cohomology of the corresponding smooth variety over the complex numbers. But Schubert varieties fail to be smooth, so we need a tricky form of cohomology — intersection cohomology — and a generalization of the Weil conjectures. For more, try these:
- Francesco Brenti, Kazhdan-Lusztig polynomials: history, problems, and combinatorial invariance.
- Frances Kirwan, An Introduction to Intersection Homology Theory, Pitman Research Notes in Mathematics 187, Longman Scientific & Technical, 1988. See especially chapter 8: the Kazhdan–Lusztig conjecture.
- David Kazhdan and George Lusztig, Representations of Coxeter groups and Hecke algebras, Inv. Math. 53 (1979), 165–184.
- David Kazhdan and George Lusztig, Schubert varieties and Poincaré duality, in Geometry of the Laplace Operator, Proc. Symp. Pure Math. 36, Amer. Math. Soc. (1980), 185–203.
- Sergei Gelfand and Robert MacPherson. Verma modules and Schubert cells: a dictionary, in Seminaire d’algebre Paul Dubriel et M. P. Malliavin, Lecture Notes in Mathematics no. 925 (1982), Springer Verlag, 1–50.
Whew! A lot of work, and we’re still fairly far from the actual calculation that was just done!
First, we need to go from to . Everything I’ve discussed for generalizes to other simple Lie groups; just happens to be the trickiest example of these. Just as is the symmetry group of -dimensional projective geometry, is the symmetry group of a kind of geometry first studied in depth by Hans Freudenthal. Instead of points, lines, planes and so on, this geometry has 8 kinds of figures following certain rules of their own! We could define a ‘flag’ in this context to consist of one of each of the 8 kinds of figures, all incident to each other. We can then use this to define a flag variety, and Schubert varieties, and -polynomials and Kazhdan–Lusztig polynomials for .
Second, the Kazhdan–Lusztig–Vogan polynomials are more subtle than the Kazhdan–Lusztig polynomials. They are defined not in terms of intersection cohomology, but directly in terms of representation theory. I mentioned that Kazhdan–Lusztig polynomials give information about Verma modules. Verma modules are among the easiest to understand of the infinite-dimensional representations of Lie groups. But there are lots of other infinite-dimensional representations, and the Kazhdan–Lusztig–Vogan polynomials give similar information about these. The ones that were just computed give information about the infinite-dimensional unitary representations of the ‘split real form’ of . They say how many times any given ‘standard’ representation contains any irreducible representation as a subquotient.
Okay. I’ll quit here before I lapse into jargon that I don’t really understand. At best I’ve given you a rough flavor of the ideas leading to what was actually done. Maybe now you’re ready to look at this:
- Jeffrey Adams, Technical details.
To go deeper still, read this:
- Jeffrey Adams and Fokko du Cloux, Algorithms for representation theory of real reductive groups.
By the way: I’ve improved the original version of this blog entry with the help of corrections in the comments below. So, with any luck, most of the corrections you see there concern mistakes that I’ve fixed by now!

Re: News about E8
From what I understand, they are calculating the representations of the split real form of E8 — i.e. the real Lie group with E8 Dynkin diagram and which has a maximal torus which is a product of R+’s (rather than some R+ and some S1 — e.g. SLn(R) has a torus, the diagonal matrices or their connected component, which is a product of R+’s, but SUn does not — this uniquely characterizes the real form).
The Kazhdan–Lusztig polynomials have two interpretations, identified by the Kazhdan–Lusztig conjectures (Beilinson–Bernstein, Vogan–Lusztig in the real case) — one is by counting points like you explained, the other as the multiplicities of irreducible representaitons in “standard” (basically induced) representations. So one way to say what they did was calculate the multiplicities of simples in standards for the split real form of E8. (Well, the web page said they did a block of this calculation — the matrix of multiplicities has block diagonal form — but maybe it’s known how to reduce everything to this block).
Geometrically this can also be said in terms of counting points, but now for orbits on the flag variety of E8 not of upper triangular matrices, but of a group KC = complexification of the maximal compact subgroup of the real group E8(R) – such subgroups always have finitely many orbits on the flag variety but their combinatorics is MUCH more involved than that of Schubert varieties.
Hope this helps somewhat…
David