It turns out most young adults don’t care about the Mars program — so NASA may resort to propaganda in an attempt to change their minds. They don’t seem to have noticed the possibility that the program really is intrinsically boring. Here’s part of a story from CNN which is being discussed by Sean Carroll and others over at Cosmic Variance:
NASA’s vision lost on Web generation
CAPE CANAVERAL, Florida (AP) – Young Americans have high levels of apathy about NASA’s new vision of sending astronauts back to the moon by 2017 and eventually on to Mars, recent surveys show.
Concerned about this lack of interest, NASA’s image-makers are taking a hard look at how to win over the young generation – media-saturated teens and 20-somethings growing up on YouTube and Google and largely indifferent to manned space flight.
“If you’re going to do a space exploration program that lasts 40 years, if you just do the math, those are the guys that are going to carry the tax burden,” said Mary Lynne Dittmar, president of a Houston company that surveyed young people about the space program.
The 2004 and 2006 surveys by Dittmar Associates Inc. revealed high levels of indifference among 18- to 25-year-olds toward manned trips to the moon and Mars.
The space shuttle program is slated to end in 2010 after construction of the international space station is completed with 13 more shuttle flights. The recent 13-day mission by Discovery’s seven astronauts was part of that long-running construction job.
When the shuttles are retired they will be replaced by the Orion spacecraft, which NASA hopes takes humans back to the moon and then on to Mars.
Even though the Dittmar surveys offer a bleak view, NASA Administrator Michael Griffin believes ventures to the moon and Mars will excite young people more than the current shuttle trips to low-Earth orbit.
“If we make it clear that the focus of the United States space program for the foreseeable future will be out there, will be beyond what we do now, I think you won’t have any problem at all reacquiring the interest of young people,” Griffin said in a recent interview.
At an October workshop attended by 80 NASA message spinners, young adults were right up there with Congress as the top two priorities for NASA’s strategic communications efforts.
Tactics encouraged by the workshop included new forms of communication, such as podcasts and YouTube; enlisting support from celebrities, like actors [from obsolete TV shows such as] David Duchovny (“X-Files”) and Patrick Stewart (“Star Trek: The Next Generation”); forming partnerships with youth-oriented media such as MTV or sports events such as the Olympics and NASCAR; and developing brand placement in the movie industry.
Outside groups have offered ideas too, such as making it a priority to shape the right message about the next-generation Orion missions.
And NASA should take a hint from Hollywood, some suggested.
“The American public engages with issues through people, personalities, celebrities, whatever,” said George Whitesides, executive director of the National Space Society, a space advocacy group. “When you don’t have that kind of personality, or face, or faces associated with your issue, it’s a little bit harder for the public to connect.”
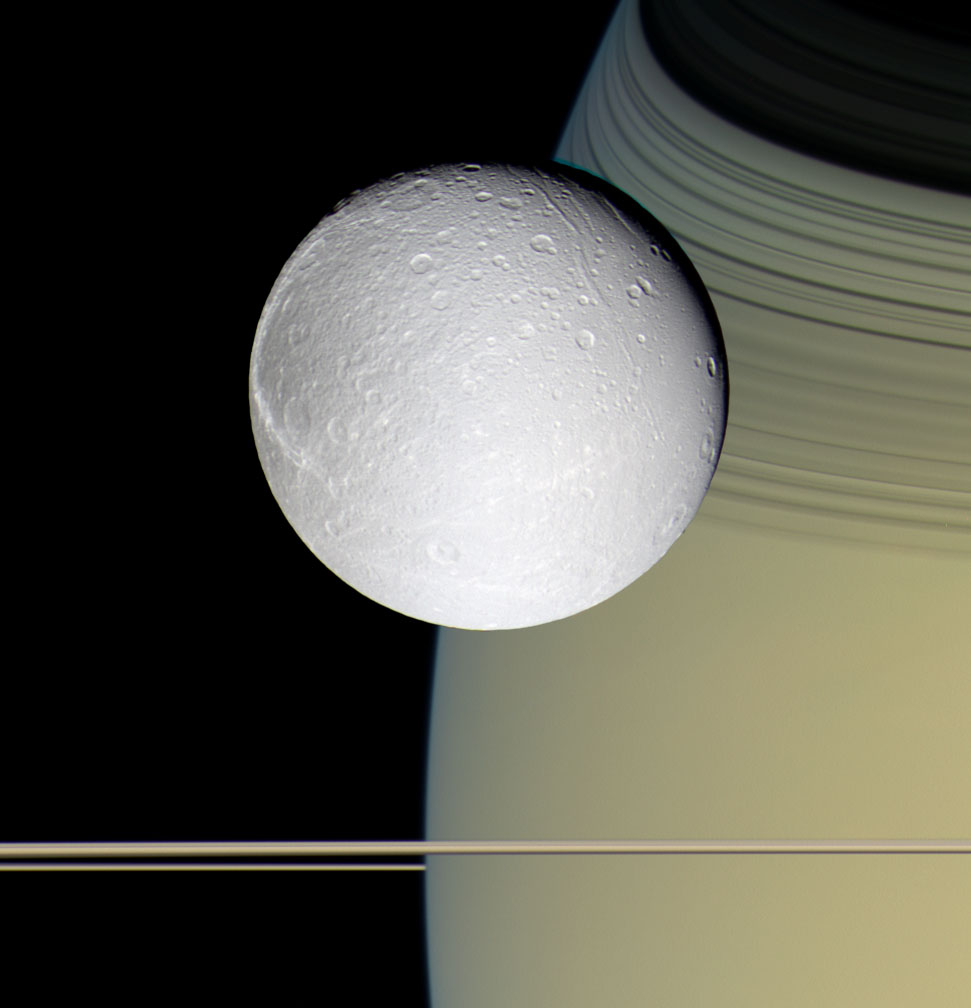

Re: This Week’s Finds in Mathematical Physics (Week 242)
So that’s what I did wrong. I should have set my advisor up with a popular internet soapbox prior to submitting my dissertation. Curses, foiled again.