Musings

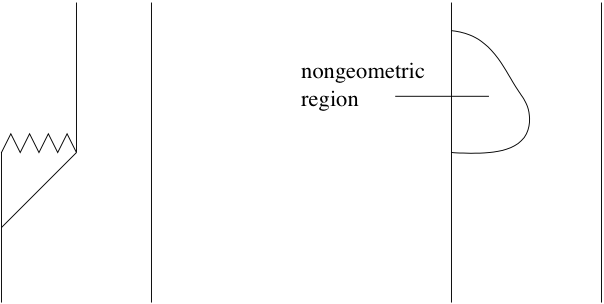
Fig. 4: The diagram on the left is the standard picture of an evaporating black hole in AdS. We believe the diagram on the right is a better description of the physics since it is clear from the gauge theory that the evolution enters a nongeometric phase and remains unitarity

This makes the same error that Ashtekar and Bojowald (whom they cite, for the same observation) make in the same context. Here, the Penrose diagram is for asymptotically AdS space, rather than asymptotically flat space, but the point is the same. One should remove the region of spacetime where (classically) curvatures would be large, and hence where quantum gravity effects are important. There is, a-priori, no classical geometrical description of what’s going on there. So the Penrose diagram (which encodes the causal structure of the part of the spacetime where a semiclassical geometrical description is possible) should not include it. Let me, therefore, excise the “non-geometric region” from both diagrams, above.

The non-geometrical region is the region behind the dashed red line. There’s no meaning to trying to assign it a classical causal structure (which is what a Penrose diagram does). Only the exterior of that region can be given a semiclassical description, and that’s all that can legitimately be placed on the Penrose diagram.

But, now, the two diagrams are conformally equivalent. They describe exactly the same semiclassical region. There is no sense in which one is “better” than the other.

Ashtekhar and Bojowald note that the “non-geometrical” region is visible from asymptotic infinity, and that this “solves” the blackhole informoration puzzle. Unfortunately, it doesn’t.

The blackhole information puzzle is a statement about physics below the dashed purple line. The point where that line intersects asymptotic infinity is the time when asymptotic observers first start seeing the non-geometrical region.

If Hawking’s semiclassical analysis is valid in the region below the dashed purple line, then we are in trouble. Hawking’s calculation says two things

1. 99.99999% of the mass of the blackhole is radiated away (and escapes out to asymptotic infinity) in the region below the purple line.
2. That radiation is perfectly thermal, and carries no information.

It’s true that the radiation that comes out between the dashed purple and dashed blue lines is highly non-thermal. But it also carries very little energy. To carry out all the information during that epoch, would require using very low-energy quanta, and hence would take a very long time. Essentially (if that’s how you believe the information comes out), it’s the situation of a long-lived remnant.

Anyway, none of this is actually relevant to the paper of Garry, Albion and Eva.

The static version of their AdS₅ blackhole looks like

If ${d\sigma}^2$ were the constant negative-curvature metric on $\mathbb{H}_3$, this would just be the metric on the Poincaré patch of AdS₅ (in Milne coordinates). Instead, ${d\sigma}^2$ is the constant negative-curvature metric on a compact hyperbolic manifold, $M$, obtained as a quotient of $\mathbb{H}_3$. There’s a singularity at $t_p=0$, a horizon, etc.

An outside observer can use standard Schwarzschild coordinates, in which the metric takes to form

where $$f(r) = \left(\frac{r_p^2}{\ell^2}-1\right)$$ The relation between the two coordinate systems (outside the horizon) is $$r= - \frac{r_p t_p}{\ell},\qquad t= -\ell \log \left(\frac{t_p^2}{\ell^2}-\frac{\ell^2}{r_p^2} \right)$$

The gauge theory description, corresponding to (1) lives on the cone $${d s}^2 = -{d t_p}^2 + t_p^2 {d\sigma}^2$$ or, equivalently (after a change of coordinates, and a conformal rescaling), on the static cylinder¹,

The gauge theory description corresponding to (2) is a somewhat complicated DBI action. But the key point is that there’s a dictionary between that description (which is only good outside the horizon) and the gauge theory on (3), which describes physics both inside and outside the horizon.

There’s a generalization of (2), with

where $\mu$ is a constant related to the mass of the blackhole.

Garry and company argue that one can study the formation of such blackholes in the gauge theory, by introducing a collapsing shell of D3-branes. In the Poincaré coordinates for the gauge theory, this corresponds to an initial scalar field configuration with eigenvalues (the transverse positions of the D3-branes) starting far from the origin (with magnitude $\propto r_p$) , and heading inward. When the eigenvalues approach the origin, the off-diagonal modes are excited, and the field configuration becomes trapped near the origin.

However, the metric (3) (because of the $RTr(\phi^2)$ coupling) gives the eighenvalues an inverted harmonic oscillator potential. Every now and then, an eignevalue tunnels out, and the blackhole (4) eventually decays back down to the “BTZ” one (though this takes an exponentially long time).

Anyway, this is a really interesting model to study many issues to do with blackholes in AdS. I just wish Garry hadn’t distracted me by exercising one of my pet peeves …

---