The n-Category Café

Re: The Modular Flow on the Space of Lattices

Re: The Modular Flow on the Space of Lattices

Re: The Modular Flow on the Space of Lattices

Here’s some stuff I wrote about certain aspects of this business:

May 20, 2006

This Week’s Finds in Mathematical Physics (Week 233)

On Tuesday I’m supposed to talk with Lee Smolin about an idea he’s been working on with Fotini Markopoulou and Sundance Bilson-Thompson. This idea relates the elementary particles in one generation of the Standard Model to certain 3-strand framed braids:

1) Sundance O. Bilson-Thompson, A topological model of composite preons, available as hep-ph/0503213.

2) Sundance O. Bilson-Thompson, Fotini Markopoulou, and Lee Smolin, Quantum gravity and the Standard Model, hep-th/0603022. It’s a very speculative idea: they’ve found some interesting relations, but nobody knows if these are coincidental or not.

Luckily, one of my hobbies is collecting mysterious relationships between basic mathematical objects and trying to figure out what’s going on. So, I already happen to know a bunch of weird facts about 3-strand braids. I figure I’ll tell Smolin about this stuff. But if you don’t mind, I’ll practice on you!

So, today I’ll try to tell a story connecting the 3-strand braid group, the trefoil knot, rational tangles, the groups SL(2,Z) and PSL(2,Z), conformal field theory, and Monstrous Moonshine.

I’ve talked about some of these things before, but now I’ll introduce some new puzzle pieces, which come from two places:

3) Imre Tuba and Hans Wenzl, Representations of the braid group B₃ and of SL(2,Z), available as math.RT/9912013. 4) Terry Gannon, The algebraic meaning of genus-zero, available as math.NT/0512248.

You could call it "a tale of two groups".

On the one hand, the 3-strand braid group has generators

     |   |   |
      \ /    |
A  =   /     |
      / \    |
     |   |   |

and

     |   |   |   
     |    \ /    
B =  |     /    
     |    / \  
     |   |   |

and the only relation is

ABA = BAB

otherwise known as the "third Reidemeister move":

|   |  |         |  |   | 
 \ /   |         |   \ /
  /    |         |    / 
 / \   |         |   / \
|   \ /           \ /   |  
|    /      =      /    | 
|   / \           / \   | 
 \ /   |         |   \ / 
  /    |         |    /
 / \   |         |   / \  

On other hand, the group SL(2,Z) consists of 2×2 integer matrices with determinant 1. It’s important in number theory, complex analysis, string theory and other branches of pure mathematics. I’ve described some of its charms in "week125", "week229" and elsewhere.

These groups look pretty different at first. But, there’s a homomorphism from B₃ onto SL(2,Z)! It goes like this:

      
        1   1
A |->  
        0   1

        1   0
B |->  
       -1   1

Both these matrices describe "shears" in the plane. You may enjoy drawing these shears and visualizing the equation ABA = BAB in these terms. I did.

I would like to understand this better… and here are some clues.

The center of B₃ is generated by the element (AB)³. This element corresponds to a "full twist". In other words, it’s the braid you get by hanging 3 strings from the ceiling, grabbing them all with one hand at the bottom, and giving them a full 360-degree twist:

|   |  |  
 \ /   | 
  /    |       A
 / \   |
|   \ /    
|    /         B 
|   / \   
 \ /   | 
  /    |       A
 / \   |
|   \ /    
|    /         B 
|   / \   
 \ /   | 
  /    |       A
 / \   |
|   \ /    
|    /         B 
|   / \   
|  |   |

This full twist gets sent to -1 in SL(2,Z):

 
             -1   0 
(AB)^3 |->  
              0  -1

So, the double twist gets sent to the identity:

             1   0 
(AB)^6 |->  
             0   1

In fact, Tuba and Wenzl say the double twist generates the kernel of our homomorphism from B₃ to SL(2,Z). So, SL(2,Z) is isomorphic to the group of 3-strand braids modulo double twists!

This reminds me of spinors… since you have to twist an electron around twice to get its wavefunction back to where it started. And indeed, SL(2,Z) is a subgroup of SL(2,C), which is the double cover of the Lorentz group. So, 3-strand braids indeed act on the state space of a spin-1/2 particle, with double twists acting trivially!

(For more on this, check out Trautman’s work on "Pythagorean spinors" in "week196". There’s also a version where we use integers mod 7, described in "week219".)

If instead we take 3-strand braids modulo full twists, we get the so-called "modular group":

PSL(2,Z) = SL(2,Z)/{+-1}

Now, SL(2,Z) is famous for being the "mapping class group" of the torus - that is, the group of orientation-preserving diffeomorphisms, modulo diffeomorphisms connected to the identity. Similary, PSL(2,Z) is famous for acting on the rational numbers together with a point at infinity by means of fractional linear transformations:

         az + b
z |->   -------
         cz + d

where a,b,c,d are integers and ad-bc = 1. The group PSL(2,Z) also acts on certain 2-strand tangles called "rational tangles". In "week229", I told a nice story I heard from Michael Hutchings, explaining how these three facts fit together in a neat package.

But now let’s combine those facts with the stuff I just said! Since PSL(2,Z) acts on rational tangles, and there’s a homomorphism from B₃ to PSL(2,Z), 3-strand braids must act on rational tangles. How does that go?

There’s an obvious guess, or two, or three, or four, but let’s just work it out.

I just said that the 3-strand braid A gets mapped to this shear:

 
        1   1
A |->  
        0   1

In "week229" I said what this shear does to a rational tangle. It gives it a 180 degree twist at the bottom, like this:

  
  |   |                |   |
  |   |                |   |
  |   |                |   |
 -------              -------
 |  T  |   |---->     |  T  |        
 -------              -------
  |   |                 \ /
  |   |                  / 
  |   |                 / \

Next, Tuba and Wenzl point out that

 
                  0   1
ABA = BAB |-> 
                 -1   0

which is a quarter turn. From "week229" you can see how this quarter turn acts on a rational tangle:

  
  |   |                       |     | 
  |   |              ____     |     |
  |   |             /     \   |     |
 -------           |     -------    |
 |  T  |   |---->  |     |  T  |    |    
 -------           |     -------    |
  |   |            |      |   \____/
  |   |            |      |    
  |   |            |      |  

It gives it a quarter turn!

From these facts, we can figure out what the braid B does to a rational tangle. So, let me do the calculation.

Scribble, scribble, curse and scribble…. Eureka!

Since we know what A does, and what ABA does, we can figure out what B must do. But, to make the answer look cute, I needed a sneaky fact about rational tangles, which is that A also acts like this:

  
  |   |                 \ / 
  |   |                  /
  |   |                 / \ 
 -------              -------
 |  T  |   |---->     |  T  |        
 -------              -------
  |   |                |   |
  |   |                |   | 
  |   |                |   |

This is proved in Goldman and Kauffman’s paper cited in "week228". With the help of this, I can show B acts like this:

 
  |   |              |          |
  |   |              |   ___    |
  |   |              |  /   \   |
 -------             | /    -------   
 |  T  |   |---->     \     |  T  |  
 -------             / \    ------- 
  |   |             |   \___/   | 
  |   |             |           | 
  |   |             |           |

And this is great! It means our action of 3-strand braids on rational tangles is really easy to describe. Just take your tangle and let the upper left strand dangle down:

           |
   ____    |               
  /    \   |              
 |    -------           
 |    |  T  |   
 |    -------  
 |     |   |  
 |     |   | 
 |     |   |

To let a 3-strand braid act on this, just attach it to the bottom of the picture!

(That’s why there were four obvious guesses about this would work: one can easily imagine four variations on this trick, depending on which strand is the "odd man out" - here it’s the upper right. It’s just a matter of convention which we use, but my conventions give this.)

In fact, even the group of 4-strand braids acts on rational tangles in an obvious way, but the 3-strand braid group is enough for now.

Let me summarize. The 3-strand braid group B₃ acts on rational tangles in an obvious way. The subgroup that acts trivially is precisely the center of B₃, generated by the full twist. Using stuff from "week229", it follows that the quotient of B₃ by its center acts on the projectivized rational homology of the torus. We thus get a topological explanation of why B₃ mod its center is PSL(2,Z).

But there’s more.

For starters, the 3-strand braid group is also the fundamental group of S³ minus the trefoil knot!

And, S³ minus the trefoil knot is secretly the same as SL(2,R)/SL(2,Z)!

In fact, Terry Gannon writes that the 3-strand braid group can be regarded as "the universal central extension of the modular group, and the universal symmetry of its modular forms". I’m not completely sure what that means, but here’s part of what it means.

Just as PSL(2,C) is the Lorentz group in 4d spacetime, PSL(2,R) is the Lorentz group in 3d spacetime. This group has a double cover SL(2,R), which shows up when you study spinors. But, it also has a universal cover, which shows up when you study anyons. The universal cover has infinitely many sheets. And up in this universal cover, sitting over the subgroup SL(2,Z), we get… the 3-strand braid group!

In math jargon, we have this commutative diagram where the rows are short exact sequences:

    1 ----> Z -----> B^3 -------> SL(2,Z) ----> 1
            |        |             |          
            |        |             |         
            v        v             v        
    1 ----> Z ---> SL(2,R)~ ---> SL(2,R) ----> 1

Here SL(2,R)~ is the universal cover of SL(2,R). Since π₁(SL(2,R)) = Z, this is a Z-fold cover. You can describe this cover explicitly using the Maslov index, which is a formula that actually computes an integer for any loop in SL(2,R), or indeed any symplectic group.

But anyway, fiddling around with this diagram and the long exact sequence of homotopy groups for a fibration, you can show that indeed:

π₁(SL(2,R)/SL(2,Z)) = B₃.

This also follows from the fact that SL(2,R)/SL(2,Z) looks like S³ minus a trefoil.

Gannon believes that number theorists should think about all this stuff, since he thinks it’s lurking behind that weird network of ideas called Monstrous Moonshine (see "week66").

And here’s the basic reason why. I’ll try to get this right….

Any rational conformal field theory has a "chiral algebra" A which acts on the left-moving states. Mathematicians call this sort of thing a "vertex operator algebra". A representation of this on some vector space V is a space of states for the circle in some "sector" of our theory. Let’s pick some state v in V. Then we can define a "one-point function" where we take a Riemann surface with little disk cut out and insert this state on the boundary. This is a number, essentially the amplitude for a string in the give state to evolve like this Riemann surface says.

In fact, instead of chopping out a little disk it’s nice to just remove a point - a "puncture", they call it. But, we get an ambiguous answer unless we pick coordinates at this point, or in the lingo of complex analysis, a choice of "uniforming parameter". Then our one-point function becomes a function on the moduli space of Riemann surfaces equipped with a puncture and a choice of uniformizing parameter.

If we didn’t have this uniformizing parameter to worry about, we’d just have the moduli space of tori equipped with a marked point, which is nothing but the usual moduli space of elliptic curves,

H/PSL(2,Z)

where H is the complex upper halfplane. Then our one-point function would have nice transformation properties under PSL(2,Z).

But, with this uniformizing parameter to worry about, our one-point function only has nice transformation properties under B₃. This is somehow supposed to be related to how B₃ is the "universal central extension" of PSL(2,Z): in conformal field theory, all sorts of naive symmetries hold only up to a phase, so you have to replace various groups by central extensions thereof… and here that’s what’s happening to PSL(2,Z)!

That last paragraph was pretty vague. If I’m going to understand this better, either someone has to help me or I’ve got to read something like this:

5) Yongchang Zhu, Modular invariance of characters of vertex operator algebras, J. Amer. Math. Soc 9 (1996), 237-302. Also available at http://www.ams.org/jams/1996-9-01/S0894-0347-96-00182-8/home.html

But I shouldn’t need any conformal field theory to see how the moduli space of punctured tori with uniformizing parameter is related to the 3-strand braid group! I bet this moduli space is X/B₃ for some space X, or something like that. There’s something simple at the bottom of all this, I’m sure.

Addenda: Another relation between the trefoil and the punctured torus: the trefoil has genus 1, meaning that it bounds a torus minus a disc embedded in R³. You can see this in the lecture "Genus and knot sum" in this course on knot theory:

6) Brian Sanderson, The knot theory MA3F2 page, http://www.maths.warwick.ac.uk/~bjs/MA3F2-page.html

This course also has material on rational tangles.

The fact that B₃ is a central extension of PSL(2,Z) by Z, and the quantum-mechanical interpretation of a central extension in terms of phases, plays an important role here:

7) R. Voituriez, Random walks on the braid group B₃ and magnetic translations in hyperbolic geometry, Nucl. Phys. B621 (2002), 675-688. Also available as http://arxiv.org/abs/math-ph/0103008.

Among other things, he points out that the homomorphism B₃ → SL(2,Z) described above is the "Burau representation" of B₃ evaluated at t = 1. In general, the Burau representation of B₃ is given by:

        t   1
A |->  
        0   1

        1   0
B |->  
       -t   t

(Conventions differ, and this may not be the best, but it’s the one he uses.) The Burau representation can also be used to define a knot invariant called the Alexander polynomial. I believe that with some work, one can use this to explain why Conway could calculate the rational number associated to a rational tangle in terms of the ratio of Alexander polynomials of two links associated to it, called its "numerator" and "denominator". In fact he computed this ratio of polynomials and then evaluate it at a special value of t - presumably the same special value we’re seeing here (modulo differences in convention).

Another issue: I wrote

For starters, the 3-strand braid group is also the fundamental group of S³ minus the trefoil knot!

And, S³ minus the trefoil knot is secretly the same as SL(2,R)/SL(2,Z)!

The first one is pretty easy to see; you start with the "Wirtinger presentation" of the fundamental group of S³ minus a trefoil, and show by a fun little calculation that this isomorphic to the braid group on 3 strands. A more conceptual proof would be very nice, though. (See "week261" for such a proof - and much more on all this stuff.)

What about the second one? Why is S³ minus the trefoil knot diffeomorphic to SL(2,R)/SL(2,Z)? Terry Gannon says so in his paper above, but doesn’t say why. Some people asked about this, and eventually some people found some explanations. First of all, there’s a proof on page 84 of this book:

8) John Milnor, Introduction to Algebraic K-theory, Annals of Math. Studies 72, Princeton U. Press, Princeton, New Jersey, 1971.

Milnor credits it to Quillen. Joe Christy summarizes it below. I can’t tell if this proof is essentially the same as another sketched below by Swiatoslaw Gal, which exhibits a diffeomorphism using functions called the Eisenstein series g₂ and g₃. They are probably quite similar arguments.

Joe Christy writes:

I wouldn’t be surprised if this was known to Seifert in the 30’s, though I can’t lay my hands on Seifert & Threfall at the moment to check. Likewise for Hirzebruch, Brieskorn, Pham & Milnor in the 60’s in relation to singularities of complex hypersurfaces and exotic spheres. When I was learning topology in the 80’s it was considered a warm up case of Thurston’s Geometrization Program - the trefoil knot complement has PSL(2,R) geometric structure.

In any case, peruse Milnor’s Annals of Math Studies for concrete references. There is a (typically) elegant proof on p.84 of "Introduction to Algebraic K-theory" [study 72], which Milnor credits to Quillen. It contains the missing piece of John’s argument: introducing the Weierstrass P-function and remarking that the differential equation that it satisfies gives the diffeomorphism to S³-trefoil as the boundary of the pair (discriminant of diff-eq, C² = (P,P’)-space).

This point of view grows out of some observations of Zariski, fleshed out in "Singular Points of Complex Hypersurfaces" [study 61]. The geometric viewpoint is made explicit in the paper "On the Brieskorn Manifolds M(p,q,r)" in "Knots, Groups, and 3-manifolds" [study 84].

It is also related to the intermediate case between the classical Platonic solids and John’s favorite Platonic surface - the Klein quartic. By way of a hint, look to relate the trefoil, qua torus knot, the seven-vertex triangulation of the torus, and the dual hexagonal tiling of a (flat) Clifford torus in S³. Joe

Swiatoslaw Gal writes:

In fact the isomorphism is a part of the modular theory:

Looking for f: GL(2,R)/SL(2,Z) → C² - {x²=y³}

(there is an obvious action of R+ on both sides: M |→ tM for M in GL(2,R), x |→ t⁶ x, y |→ t⁴ y, and the quotient is what we want).

GL(2,R)/SL(2,Z) is a space of lattices in C. Such a lattice L has classical invariants

g₂(L) = 60 sum_{z in L’} z^-4,

and

g₃(L) = 140 sum_{z in L’} z^-6, where L’=L-{0}

The modular theory asserts that:

1. For every pair (g₂,g₃) there exist a lattice L such that g₂(L) = g₂ and g₃(L) = g₃ provided that g₂³ is not equal to 27g₃². 2. Such a lattice is unique. Best, S. R. Gal

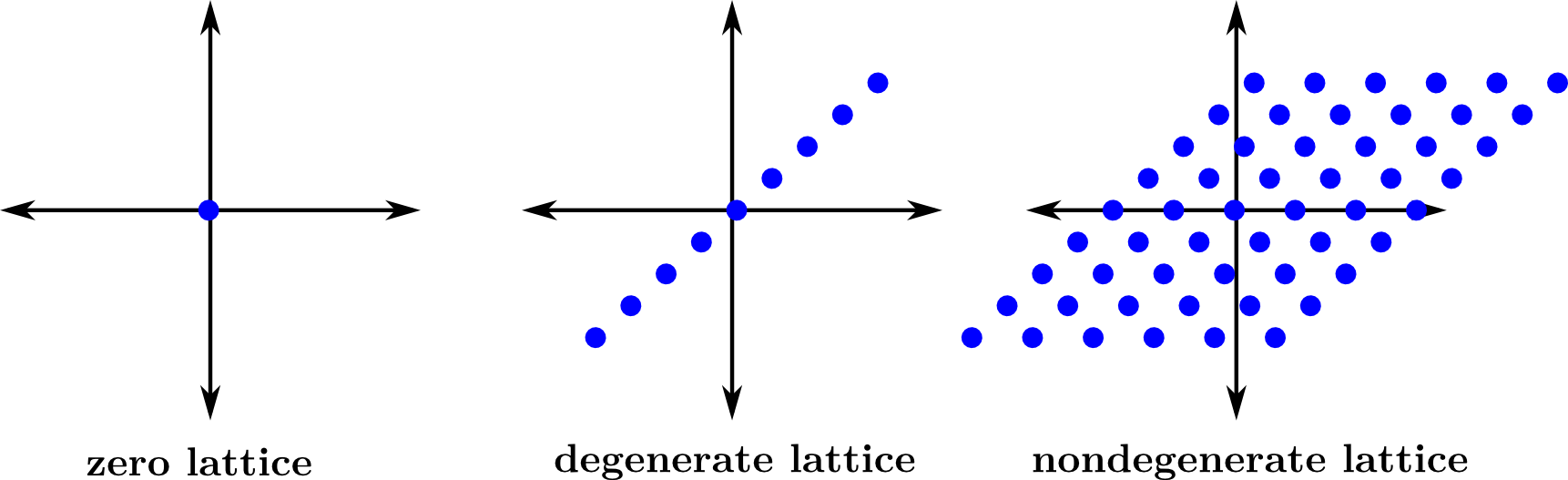
The quantity g₂³ - 27 g₃² is called the "discriminant" of the lattice L, and vanishes as the lattice squashes down to being degenerate, i.e. a discrete subgroup of C with one rather than two generators.

  |   |
  |   |
  |   |
  |   |

  |   |                |   |
  |   |                |   |
  |   |                |   |
 -------              -------
 |  T  |   |---->     |  T  |        =  "T + 1"
 -------              -------
  |   |                 \ /
  |   |                  / 
  |   |                 / \

  |   |             |     |     
  |   |             |     |    ____
  |   |             |     |   /    \
 -------            |    -------    |
 |  T  |   |---->   |    |  T  |    |    =  "-1/T"
 -------            |    -------    |
  |   |              \___/   |      |
  |   |                      |      |
  |   |                      |      |

Re: The Modular Flow on the Space of Lattices

Re: The Modular Flow on the Space of Lattices

Re: The Modular Flow on the Space of Lattices

Re: The Modular Flow on the Space of Lattices

In my post, I skipped over the `Great Dictionary’. By that, I mean the equivalence Ghys mentions in his talk (pg 26 of the slides) between:

• periodic orbits (i.e. knots) in the modular flow
• conjugacy classes of hyperbolic elements in $PSL(2, \mathbb{Z})$
• ideal classes in quadratic fields
• indefinite integral quadratic forms in two variables
• continuous fractions

This is clearly a long and beautiful story, well known to some of you much better than me. I’d like to point out here a cool fact about the “indefinite integral quadratic forms” picture (reference: Lemmermeyer).

Each closed orbit in the modular flow corresponds to an indefinite integral quadratic form in two variables in two variables $x$ and $y$, that is an integral polynomial of the form

whose discriminant $D := B^2 - 4AC$ is positive. That’s a cool fact by itself: a vivid picture of a quadratic form as a knot! But more is true. From the length (the number $t$ above) of the closed orbit, one can read off the discriminant $D$ of the corresponding quadratic form. And so the number of closed orbits with the same length is the class number h(D) — the number of equivalence classes of quadratic forms with discriminant $D$.

This `dynamical systems’ view of indefinite quadratic forms is very useful. You can construct a zeta function as in TTWF216 and apply the Selberg trace formula, and hopefully prove something in number theory. This is exactly what Peter Sarnak did in Class numbers of indefinite binary quadratic forms. He used the dynamical systems description of the space of quadratic forms to derive an asymptotic formula for the class number $h(D)$.