Towards the FFRS Description of 2dCFT (A)
Posted by Urs Schreiber
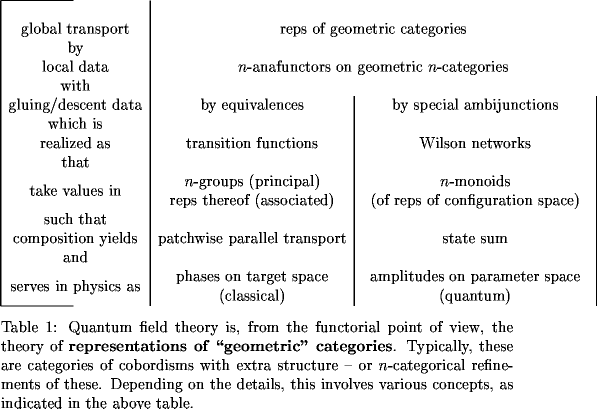
With a small group of students here in Hamburg, we want to talk about stuff that will eventually enable us to understand the FFRS theorem, which explains how 2-dimensional rational conformal field theories are characterized by special Frobenius algebra objects internal to modular tensor categories.
I have no idea if we will really get to this point in finite time, or what else will happen. But at least tomorrow I shall, informally, begin by explaining some basics of 2-dimensional topological field theory and its description in terms of Frobenius algebras.
Here are handwritten notes for the first session:
It is clear that this leaves a lot of room for improvement. But it is a start.
I shall try to indicate
- what cobordism categories are
- why representations of these are addressed as quantum field theories
- what the generators and relations of 2-dimensional cobordisms look like
- why that implies that the corresponding field theories are given by Frobenius algebras .
Much of this goes back to the famous lecture
Greg Moore
D-Branes, RR-Fields and K-Theory
(slides).
There are some rather obvious-looking facts involved in this, which were strictly proven only in
A. Lauda & H. Pfeiffer
Open-closed strings: Two-dimensional extended TQFTs and Frobenius algebras
math.AT/0510664
Parts of the old lecture by Moore has now made it into this paper:
Gregory W. Moore, Graeme Segal
D-branes and K-theory in 2D topological field theory
hep-th/0609042 .
Next time one should explain
- what state sum models are
- how the coloring by Frobenius algebras can be understood using adjunctions
- how state sum models can be understood as locally trivialized 2-functors, generalizing the way that gerbe holonomy is described by Frobenius algebroids with invertible products